Economic Development, Vertical Intra-Industry Trade and Gains from Trade ()
Keywords:Product Quality; Volume of Trade; Intra-Industry Trade; Economic Development; Gains from Trade
1. Introduction
It has been generally accepted that trade is concentrated among the industrialized countries, and trade among industrialized countries is principally vertical intra-industry trade (IIT). For example, Bergoeing and Kohoe [1] found that trade within the OECD countries has increased at a much more rapid rate than OECD trade with the rest of the world. Additionally, Gabrisch and Segnana [2] determined that more than 50% of trade among EU countries was IIT, and that the intra-industry trade among EU countries was composed, in large part, of vertical IIT.
In explaining these characteristics of trade, most studies have emphasized economies of scale, product differentiation, and imperfect competition as the determinants of intra-industry trade. For example, seminal papers by Krugman [3] and Lancaster [4] developed this theoretical framework. However, these models pertain to horizontal product differentiation, assuming that these products are identical in quality. Flam and Helpman’s [5] considered a model where the North exports high-quality products and the South exports low-quality products, and they evaluated the effects on trade of factors such as technical progress, income distribution, and population growth. Hallak [6] suggested that Linder’s hypothesis (see Linder [7]) might not be found due to a systematic bias caused by aggregation across sectors, and he proposed that this hypothesis should be formulated at the sector level with a control for inter-sectoral determinants of trade.
In the opposite direction, Falvey and Kierzkowski [8] returned to HOS fashion to explain IIT without modifying traditional trade theoretic models. Davis [9] and Bhagwati and Davis [10] showed that IIT can occur in traditional trade models as a consequence of technical differences within an industry. For this reason, they argued whether one should call this kind of trade vertical IIT or just inter-industry trade. Especially, Lüthje [11] stressed that foreign trade is mostly inter-industry trade because what called vertical IIT is in fact not IIT, but inter-industry trade.
The contribution of this paper is as follows. First, we considered determinants of the international trade with the assumption that products are vertically differentiated. It is worthwhile to note that Krugman [3] and Lancaster [4] investigated IIT regarding horizontally differentiated products so these models do not provide any explanation to vertical intra-industry trade. Second, our paper does provide an explanation to the gains from vertical IIT while previous works do not. This finding is, at least in the settings of this model, the evidence against the argument that vertical IIT is actually inter-industry trade. Furthermore, this paper proposes a new and simple model to investigate vertical IIT.
This paper is organized as follows. Section 2 describes the basic model, and Section 3 derives the determinants of trade volume and vertical IIT share using a theoretical model. Section 4 examines the sources of gains from trade. Section 5 presents empirical implications of our findings. The final section contains our conclusions.
2. The Model
We consider a 2 × 2 × 2 model: two countries, two firms, and two varieties of goods. Suppose that there is a world in which only two countries exist. One of them we will call Home, and the other we will refer to as Foreign. Both countries are assumed to be at the same level of economic development, and their per-capita incomes are assumed to be identical. We also assume that their technology levels differ from industry to industry. For example, Home enjoys higher-level technology in some industries, but Foreign has higher-level technology in other industries. As the two countries trade with one another, Home exports higher-quality goods in some industries, but also exports lower-quality goods in other industries. Even though there may be many different industries in a country, we hereinafter focus on the trade of goods in a single industry wherein goods are identical, but can be differentiated by quality. We utilize the term “qualitydifferentiated good” to reference this industry.
2.1. Supply Side
Each country has one firm that produces one type of the quality-differentiated good1. Without any loss of generality, we assume that the foreign firm produces high-quality goods and that the home firm produces low-quality goods in the considered industry; this implies that the technology level of the foreign firm is higher than that of the home firm in that industry2. In Grossman and Helpman’s [13] rising product quality model, the quality of each product is determined endogenously with R&D investments. In this paper, we assume that the quality of each product is a consequence of R&D investment; however, this expenditure has been sunk and become a fixed cost in the production process3. For this reason, we consider these qualities to be given and can be used as a proxy for technology level.
In producing the goods, two firms have cost functions as follows:
 (1)
(1)
In the cost function (1), we utilize H and L to designate the high and low quality firms, respectively. Thus, the total cost,  , is the cost of firm i associated with
, is the cost of firm i associated with  units of output. The marginal variable cost, or c, is identical for both firms. The fixed cost,
units of output. The marginal variable cost, or c, is identical for both firms. The fixed cost,  , is the quality cost incurred by firm i. The higher the quality is, the more a firm has spent on R&D. Thus, we can say
, is the quality cost incurred by firm i. The higher the quality is, the more a firm has spent on R&D. Thus, we can say . It is worth mentioning that the total cost functions of both firms possess internal economies of scale property.
. It is worth mentioning that the total cost functions of both firms possess internal economies of scale property.
2.2. Demand Side
The populations of consumers in Home and Foreign are the same and are normalized to 1. In each country, consumers are distributed uniformly between 0 and b according to the preference for quality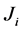 . Thus, parameter b measures the heterogeneity in consumer tastes for quality in a country4. Each consumer may purchase a good from one of the firms, or none at all. The consumer’s utility function when consuming a good with quality level q and price p is described as follows.
. Thus, parameter b measures the heterogeneity in consumer tastes for quality in a country4. Each consumer may purchase a good from one of the firms, or none at all. The consumer’s utility function when consuming a good with quality level q and price p is described as follows.
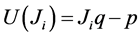 (2)
(2)
This function is an indirect utility function of consumer i, identified by the parameter . A consumer’s utility is zero if he does not purchase this good. In the case of goods purchased only from one firm, a consumer will buy the good if it generates non-negative utility. If goods from two firms are available, consumers will elect to purchase the good that generates a higher and non-negative utility.
. A consumer’s utility is zero if he does not purchase this good. In the case of goods purchased only from one firm, a consumer will buy the good if it generates non-negative utility. If goods from two firms are available, consumers will elect to purchase the good that generates a higher and non-negative utility.
We denote the corresponding quality level and price level of high-quality and low-quality goods by ,
,  and
and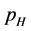 ,
,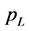 . The marginal consumer JLH is indifferent with regard to the consumption of either of the two products. That is, the consumer, JLH, satisfies the condition
. The marginal consumer JLH is indifferent with regard to the consumption of either of the two products. That is, the consumer, JLH, satisfies the condition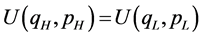 . In Equation (2), the marginal consumer, JLH, is defined as
. In Equation (2), the marginal consumer, JLH, is defined as
 (3)
(3)
Some consumers do not wish to buy any goods at the prevailing prices. We denote by JL a consumer who is indifferent with regard to the purchase of a low-quality product or refraining from buying. In Equation (2), this type of marginal consumer is defined as
 (4)
(4)
All consumers satisfying the condition Ji > JLH will purchase high-quality goods, all consumers having JL < Ji < JLH will purchase low-quality goods, and all consumers having Ji < JL will purchase no goods5.
2.3. Trade between Countries
Let us model a game as follows: There is not any trade barrier between Home and Foreign, and the two firms sell their goods to both countries. Thus, the competition is purely Bertrand in price. We also assume that no price discrimination is possible because the goods can move freely without any transportation costs between the two countries.
Now, we go back to the two-country world. If countries are in trade, each country exchanges its products with the other country6. Home buys high-quality products from Foreign, and Foreign buys low-quality products from Home. Put differently, home consumers with a higher preference for quality will consume high-quality goods imported from Foreign, whereas foreign consumers with a lower preference for quality will buy low-quality goods from Home.
Trade between the two countries can be explained by Figure 1. Home exports low-quality goods to Foreign, and imports high-quality goods from Foreign.
3. Trade Determinants
3.1. Price Competition
The optimal price of the two firms can be obtained by using the Nash Equilibrium. Based on Figure 1, demands for low-quality and high-quality goods are:
 (5)
(5)
 (6)
(6)
Using (1), (5) and (6), we can derive the profit functions of low-quality and high-quality firms, as follows:
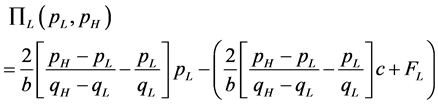 (7)
(7)
 (8)
(8)
The first part comes from the revenue of low-quality (or high-quality) firm; the second part is the total cost of low-quality (high-quality) firm.
From (7), the best response of the low-quality firm , is derived from the first order condition
, is derived from the first order condition , which is
, which is
 (9)
(9)
Similarly, from (8), the best response of the high-quality firm , is derived from the first order condition
, is derived from the first order condition , which is
, which is
 (10)
(10)
The Nash Equilibrium is derived by solving (9) and (10) with  and
and . We determine the opti-mal prices as follows:
. We determine the opti-mal prices as follows:
 (11)
(11)
 (12)
(12)
Lemma 1: Low-quality firm will not offer any goods if the consumer’s preference diversity for quality is low .
.
Proof: A low-quality firm will not produce low-quality goods if the optimal price is lower than the marginal variable cost, which is . From (11), we can readily derive that
. From (11), we can readily derive that .
.
Lemma 1 implies that low-quality firms will exit the market if the quality preference diversity of consumers is insufficient. In this case, high-quality firms will monopolize the market. In other words, the trade flow of the considered goods is only from Foreign to Home. Preference diversity b, is a measure of differences in the taste for quality among consumers. As conceptualized by Gabszewics and Thisse [17], a preference for quality is dependent on consumers’ income; the more income a consumer has, the more he is willing to pay for a given quality level. Additionally, Sutton [15] also came to a similar conclusion, and determined that the preference for quailty increases with rising income. For this reason, b variable can be seen as a proxy for a country income level7.
We allow  and
and . The parameter a, re-presents the relative level of technology between Foreign and Home. In addition, it is worthy noting that an increase in Q implies that technology levels in both countries increase (given that a is unchanged). Thus, Q can be used as a proxy for our world technology level (or regional technology level when we view the two countries as a region).
. The parameter a, re-presents the relative level of technology between Foreign and Home. In addition, it is worthy noting that an increase in Q implies that technology levels in both countries increase (given that a is unchanged). Thus, Q can be used as a proxy for our world technology level (or regional technology level when we view the two countries as a region).
A region is considered to have a high economic development level if it has high income and a high technology level. For this reason, we utilize bQ as a proxy for regional economic development. It is also worth noting that . In addition, we always have
. In addition, we always have  or bQ can be less than
or bQ can be less than  for any possible value of a. Based on lemma 1 with the product of b and Q serving as a proxy for the regional economic development level, we derive the following proposition:
for any possible value of a. Based on lemma 1 with the product of b and Q serving as a proxy for the regional economic development level, we derive the following proposition:
Proposition 1: Vertical intra-industry trade is unlikely to be observed in a region at very low economic development .
.
3.2. Determinants of Intra-Industry Trade
If , intra-industry trade between Home and Foreign will occur. Home exports low-quality products to Foreign, but imports high-quality products from Foreign. The export value and import value of Home can be expressed as follows.8
, intra-industry trade between Home and Foreign will occur. Home exports low-quality products to Foreign, but imports high-quality products from Foreign. The export value and import value of Home can be expressed as follows.8
 (13)
(13)
 (14)
(14)
Now, replacing optimal prices in (11) and (12) into (13) and (14), and then substituting 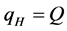 and
and , we obtain the following:
, we obtain the following:
 (15)
(15)
 (16)
(16)
Proposition 2: When both trading countries are at a higher level of economic development, the volume of trade between them will be higher.
Proof: From (15) and (16), we can conclude directly that X and M both increase in b and Q.
Now, note that the imports (M) shown in (16) always exceed the exports (X) shown in (15). Thus, the Grubel-Lloyd index used to compute the IIT index can be written as follows, and is related to the ratio of exports to imports, .
.
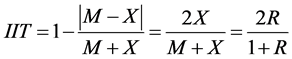 (17)
(17)
We can see that IIT increases directly with R. That is, IIT behaves as R does.
Proposition 3: The regional development exerts a positive impact on vertical IIT share. However, when the region achieves a certain level of economic development, vertical IIT share tends to no longer be affected by the development level.
Proof: Based on the signs of , we can conclude when regional development exerts a positive impact on IIT (See the appendix for detailed proofs).
, we can conclude when regional development exerts a positive impact on IIT (See the appendix for detailed proofs).
For the purpose of visually presenting propositions 1 and 3, we draw Figure 2 with 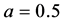 and
and . First, IIT is zero when
. First, IIT is zero when 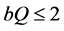 or the region’s trade is purely characterized by inter-industry trade, as stated in proposition 1. Second, IIT increases when x rises until
or the region’s trade is purely characterized by inter-industry trade, as stated in proposition 1. Second, IIT increases when x rises until . After this level, an increase in
. After this level, an increase in  will result in a small drop in IIT. However, this fall in IIT is quite small and tends toward zero when
will result in a small drop in IIT. However, this fall in IIT is quite small and tends toward zero when  approaches infinity.
approaches infinity.

Figure 2. Relationship between economic development and vertical IIT.
Proposition 4: The IIT index increases as the technology levels between countries become similar.
Proof: We only prove . (See the appendix for detailed proofs).
. (See the appendix for detailed proofs).
4. Gains from Trade
In this section, we try to identify why countries become better off when they are in trade with each other. We prove that countries in trade are better off for the following three reasons: goods are produced more efficiently, more goods are consumed, and a greater variety of goods are available. All of these findings are derived from analyses of the welfare of both countries before trade and after trade.
4.1. Closed Economy
If the two countries are not currently trading with each other, the high-quality firm will be a monopolist in Foreign and the low-quality firm will be a monopolist in Home.
For the purposes of our analyses, the following are the optimal prices, the consumers with the lowest preference for quality who can buy goods, and the welfare in both Home and Foreign. The detailed mathematical calculations are presented in the appendix section.
1) The optimal prices established by the high-quality and low-quality firms are
 (18)
(18)
(In Foreign, set by high-quality firm)
 (19)
(19)
(In Home, set by low-quality firm)
2) The marginal consumer with the lowest preference for quality can buy the good:
 (20)
(20)
 (21)
(21)
3) Welfare when countries are closed:
 (22)
(22)
 (23)
(23)
Note that the superscript NF or NH indicates the “notrade” case in Foreign or in Home.
4.2. In Trade
If Home and Foreign are currently trading, each country exchanges its products with the other country. Home consumers with a high preference for quality buy highquality products from Foreign, and foreign consumers with low preference for quality buy low-quality products from Home. From section III, we have the following:
1) From (11) and (12), the optimal prices established by high-quality low-quality firms are
 (24)
(24)
(High-quality good)
 (25)
(25)
(Low-quality good)
Note that these prices are the same in both countries.
2) Substituting (11) and (12) into (3) and (4), the marginal consumers with the lowest preference for quality can buy the high-quality good and the low-quality good as follows:
 (26)
(26)
(For high-quality goods in both countries)
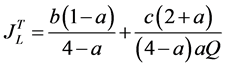 (27)
(27)
(For low-quality goods in both countries)
3) Welfares when countries are in trade (see appendix for detailed mathematic calculations).
Note that the TF or TH superscript indicates the “trade” case in Foreign or in Home.