1. Introduction
In 1881, C. Jordan gave the notion of variation of a function in [1] , and from this moment, many generalizations and extensions have been given. Consequently, the study of notions of generalized bounded variation forms an important direction in the field of mathematical analysis. Another important generalization of the space of bounded variation in the Jordan’s sense is the notion of the space of functions of second bounded variation studied by Ch. J. de la Vallée Poussin in 1908 in [2] . It is defined as follows:
Definition 1 Let
be a partition of the interval
of the form
, and
be a function
. The nonnegative real number
is called the second variation of
on
, where the supremum is taken over all partitions
of
. In the case that
, we say that
has bounded second variation on
and we denote it by
.
A well-known generalization of the functions of bounded variation was done by N. Wiener in 1924 in [3] . The p-variation of a function
is the supremum of the sums of the pth powers of absolute increments of
over non over- lapping intervals. Wiener mainly focused on the case
, the 2-variation.
Definition 2 Let
be a partition of the interval
of the form
,
be a function
and
. The nonnegative real number
is called the Wiener p-variation of
on
where the supremum is taken over all partitions
of
. In the case that
, we say that
has bounded Wiener p-variation on
and we denote it by
.
The pth-variations were reconsidered in a probabilistic context by R. Dudley in [4] and [5] , in 1994 and 1997, respectively. Many basic properties of the variation in the sense of Wiener and a number of important applications of the concept can be found in [6] [7] . The paper by V. V. Chistyakov and O. E. Galkin in [8] in 1998 is very important in the context of p-variation.
The class of nonlinear problems with exponent growth is a new research field and it reflects a new kind of physical phenomena. In 2000 the field began to expand even further. Motivated by problems in the study of electrorheological fluids, Diening [9] raised the question of when the Hardy-Littlewood maximal operator and other classical operators in harmonic analysis are bounded on variable Lebesgue spaces. These and related problems are the subject of active research to this day. These problems are interesting in applications (see [10] [11] [12] [13] ) and gave rise to a revival of the interest in Lebesgue and Sobolev spaces with variable exponent, the origins of which can be traced back to the work of Orlicz [14] in the 1930’s. In the 1950’s, this study was carried on by Nakano [15] [16] who made the first systematic study of spaces with variable exponent. Later, Polish and Czechoslovak mathematicians investigated the modular function spaces (see for example Musielak [17] [18] , Kovacik and Rakosnik [19] and Kozlowski [20] ). We refer to the book [13] for detailed information on the theoretical approach for the Lebesgue and Sobolev spaces with variable exponents. Recently, in [21] Castillo, Merentes and Rafeiro studied a new space of functions of generalized bounded variation. They introduced the notion of bounded variation in the Wiener sense with variable exponent
on
and study some of its properties.
Definition 3 Given a function
, a partition
of the interval
, and a function
, the nonnegative real number
(1.1)
is called the Wiener variation with variable exponent (or
-variation in Wiener’s sense) of
on
where
is a tagged partition of the interval
, i.e., a partition of the interval
together with a finite sequence of numbers
subject to the conditions that for each j,
.
In case that
, we say that
has bounded Wiener variation with variable exponent (or bounded
-variation in Wiener’s sense) on
. The symbol
will denote the space of functions of bounded
-variation in Wiener’s sense with variable exponent on
.
The aim of this paper is to provide a description of the new class formed by the functions of bounded
-variation in the sense of Wiener as an extension to the double case of the previous concept. Also, we prove structural properties for mappings of bounded
-variation in the Wiener’s sense. Finally, we show that any uniformly bounded composition operator that maps the space
into itself necessarily satisfies the so-called Mat- kowski’s conditions.
2. Preliminaries
In this section we present some definitions and propositions that will be used through out this paper.
Definition 4 Let
,
be a partition
of the interval
, and
be a function. The nonnegative real number
is called the De La Vallée Poussin-Wiener variation (or
-variation in Wiener’s sense) of
on
where the supremum is taken over all
partitions
of
. In the case that
, we say that
has bounded
-variation on
and we denote by
.
For the interested readers can see some of the properties in [2] [7] and other related problems in [22] .
Proposition 1 Let
be a function with
and consider
. Then
1)
if and only if
is a liner function.
2) If
, then
is bounded in
.
3)
is a convex function.
Proof. 1) Suppose first that
is a linear function. If
for all
, with
, then by Definition 4, it follows easily that
.
Now, if
, then by Definition 4 we have
Hence, for any partition
of the interval
, we should have that
Then, any term in the sum should be zero. Since the function
vanishes only at zero, it follows that
Therefore,
is equal to a linear function.
2) Suppose that
and
is not bounded, then there exists a sequence
,
,
such that
when
. Let
be a subsequence of
such that
converge to
. Then, as
is a subsequence of
, so
We have that
Moreover for
we get
In consequence,
, since
as
, which is a contradiction with
. Therefore
is bounded.
3) Let
be two functions,
such that
and
be a partition of
. Since
is convex and nondecreasing, we have that
Then,
is a convex function.
Definition 5 (Norm in
) The functional
defined by
(2.1)
is a norm.
In [7] , the authors have shown that the linear space
with the norm (2.1) is a Banach space and
.
3. Main Results
In [23] the authors present and study the space of functions of bounded
-variation as an extension of the space
. In this section, our goal is to study the corresponding space of functions of bounded second
-variation, with
be a variable exponent, as an extension of
.
Definition 6 Let
be a function
,
be a partition
of the interval
and
be a function. The nonnegative real number
is called the De La Vallée Poussin-Wiener variation with variable exponent (or
-variation in De La Vallée Poussin-Wiener’s sense) of
on
, where
is a tagged partition of the interval
, i.e., a partition of the interval
together with a finite sequence of numbers
subject to the conditions
for each
. It is worth to note that by definition (we take supremum over all partitions), the number
does not depend on the election of the argument of the exponent. In the case that
, we say that
has bounded
-variation on
.
We will denote by
the space of functions of bounded
-variation in Wiener’s sense with variable exponent in
. It is endowed with the functional:
(3.1)
Then,
Remark 3.1 Given a function
.
1) If
for all
, then
.
2) If
for all
and
then
, i.e., the space of bounded
-variation in De
la Vallée Poisson-Wiener’s sense with variable exponent is exactly the space of bounded
-variation in De la Vallée Poisson-Wiener’s sense.
Given a function
, that is, a variable exponent function, let us define as in the literature,
and
It is said that the exponent p is admissible if the range of p is in
and
is finite.
Let us recall a classical concept in the theory of function spaces. Let X be a vector space over
. A convex and left-continuous function
is called a convex pseudo-modular on X if for arbitrary x and y, there holds:
1)
,
2)
for every
such that
,
3)
for every
.
It is possible to see that for p be an admissible function, the functional
is a convex pseudo-modular.
Proposition 2 Let
be an admissible function. Then
is a convex pseudo-modular.
Proof. We have that for any
,
. Moreover, the fact that for any
,
whenever
follows immediately from the definition.
Finally, with the same kind of argument than in Proposition 1(c) it follows
that for
and
we have that
Definition 7 A convex and left-continuous function
is called semimodular on
if
1)
,
2)
for every
, and
3) if
for every
, then
.
For
be an admissible function, the functional
is a semimodular on
.
Proposition 3 Let
be an admissible function. Then
is a semimodular.
Proof. Let
and
be a tagged partition of
, then
On the other hand, if
for every
, necessarily it follows that
.
Proposition 4 Let
be a vector space,
be a semimodular on
and
. Then
1)
if and only if
,
2) if
, then
,
3) if
, then
,
4) for every
,
.
Theorem 1 Let
be a function and
be an admissible function, then
.
Proof. Let
be an admissible function,
be a tagged partition of the interval
,
and
Then,
The proof of the fact that
will be by contradiction. That is, we assume that
. Therefore, there exists a
tagged partition
such that
Since
and
we get
But this is satisfied only for a finite number of terms, because in opposite case we would get
which is a contradiction as
. Then, taking supremum we get
Theorem 2 Let
be an admissible function. If
, then it follows that for any
(3.2)
Proof. By the definition of
and
we have that, for each
, there are partitions
and
with
and
, and sequences of points
and
such that
for
and
for
that satisfies
and
Taking
and the points
, we get a partition of
such that
which implies that
(3.3)
Letting
first, and then taking the corresponding supremum in the left-hand side of (3.3), it follows (3.2).
Define
Lemma 1 Basic properties of the
-variation in De La Vallée Poussin-Wiener’s sense Let
be an arbitrary map. We have the following properties:
(P1) For any
, we have that
(P2) Monotonicity: If
and
, then
,
, and
(P3) Semi-additivity: If
, then
(P4) Change of variable: If
is a monotone function, then
(3.4)
(P5) Regularity:
Proof. (P1) We have that for any
,
(P2) Let
and the partition
. Then
The other cases follow in a similar way.
(P3) Semi-additivity: It is obtained in Theorem 2.
(P4) It follows as in ( [23] , Lemma 2 (P4)). Indeed, let
,
be a (not necessarily strictly) monotone function,
be a
tagged partition of the interval
,
and
with
, then
On the other hand, if a partition
of
is such that
for
then there exists
such that
and, again by the monotonicity of
(P5) By monotonicity
On the other hand, for any
such that there exists a tagged partition
of
with
We define
a partition of the interval
then
and
, i.e.,
Lemma 2 If
, then
for all
.
Proof. Let
such that
. Then, consider any partition
of
,
and any finite sequence of numbers
subject to the conditions
for each
. It follows that
as
. Then, as this inequality follows for all terms in the sum
Taking supremum in any partition, it follows that
Proposition 5 Let
be an admissible function. The space
is a vectorial space.
Proof. Let
and consider any partition
and any finite sequence of numbers
subject to the conditions
and
. By definition, there exists
such that
Let
. By Lemma 2, it follows that
The rest of the proof follows analyzing the possible cases.
1) If
, then
.
2) If
and/or
. Let
, and consider any tagged partition
of
,
which is any partition
of
and any finite sequence of numbers
subject to the conditions
for each
. Then, by convexity of
, when
, it follows that
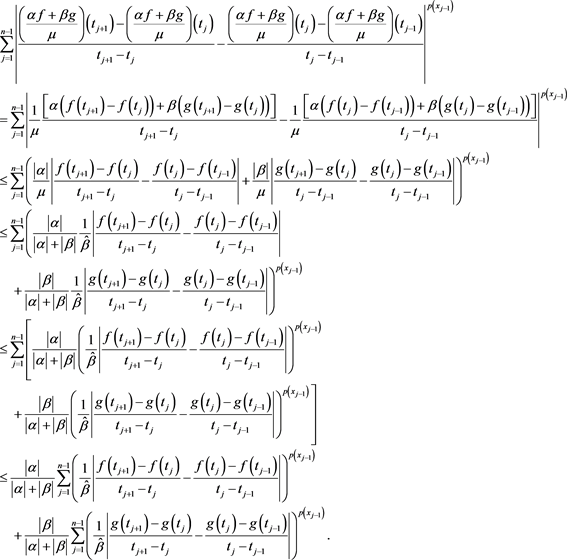
Therefore,
Then, taking supremum over all partitions, we get that
Therefore
.
The other properties of a vectorial space follow similarly.
Theorem 3 Let
be an admissible function. The space
is a normed space.
Proof. Let
be an admissible function. Let us analyze all the properties of a norm.
1) By definition of
, we have that
for all
2) To prove that
for any
, we consider the possible cases:
- If
, then
for any
.
- If
, then
3) Property
is satisfied by using that
,
and the previous proposition.
4) Let us see that
if and only if
.
- If
, then by definition of the norm,
and
, and
Hence, we have by Proposition 3 and Proposition 4 (2) that
Therefore,
, and hence,
Therefore, for any tagged partition
of the interval
, that is a partition
together with a finite sequence of numbers
subject to the conditions
for each
, we have that
So that
Consider the partition
. We get that
Then
As
is obtained that
for all
.
- In other hand, if
, then
for all
. Hence,
and
. Therefore, by definition,
Theorem 4 Let
be an admissible function. The space
is a
Banach space endowed with the norm in (3.1).
Let
be a Cauchy sequence in
. Then, for all
,
there exists
such that
Therefore, by definition it follows that
(3.5)
(3.6)
and
(3.7)
Then, by (3.5) and Proposition 4 (2) we have that
It implies that for fixed
,
is a Cauchy sequence in
. Indeed,
then for all
,
we get
so
As
thus
Therefore
and by property of log
Then
and hence
i.e.
Let
for any
and let
be any partition
of
and a sequence
such that
for any
. It follows that for all
Then, letting
, for any
it follows that
(3.8)
Therefore, as (3.8) follows for any tagged partition
of
, taking supremum over all tagged partitions it follows that
(3.9)
Moreover, by (3.6) and (3.7), we have that
Then, letting
, we have that
(3.10)
Then, (3.9) and (3.10) imply that for
sufficiently large
Hence, as
we obtain that
Theorem 5 Let
be an admissible function. Then, we have:
1) If
, then
is bounded in all the interval
.
2)
for functions
Let us proof (a). Suppose that
and
is not bounded. Then, there exists a sequence
,
,
such that
when
. Let
be a subsequence of
such that
converge to
. As
is a subsequence of
, so
Case 1: Suppose that
and let
such that
for some
, then
and since
is continuous
On the other hand
tend to infinity as
. Then
and hence
, which is a contradiction.
Case 2: Suppose that
and let
such that
for some
, then
Since
is continuous
On the other hand
tend to infinity as
then
and then
, which is a contradiction.
Let us proof (b). Taking
, since
, it follows that
for any tagged partition
and any sequence of points
such that
for
Therefore,
since in particular
for any
. Taking supremum to both sides, we obtain that
. Then, by definition it follows that
and the general case follows from the homogeneity of the norm. ,
4. Functions in
and Hölder Continuous Functions
In this section we prove also that if a function is the composition of a bounded monotone function with a
-Hölder continuous function with
, then the function is in
.
Definition 8 A function
is Hölder continuous of exponent
, where
is a positive function such that
, if
for all
. The least number
satisfying the above inequality is called the Hölder constant of
.
Proposition 6 Let
be an admissible function and
such that
, where
is a bounded monotone function and
is
-Hölder continuous with
. Then
.
Proof. Assume that
is nondecreasing. Since
, by virtue of the change of variable
(4.1)
If
is a partition of
and
is a sequence of points
for
then
Therefore, by taking supremum over any tagged partition, it follows that
by the boundedness of
. Hence, by (4.1)
5. The Matkowski’s Condition
Let us show as an application that, any uniformly bounded composition operator that maps the space
into itself satisfies the Matkowski’s condition.
Theorem 6 Suppose that the composition operator
generated by
maps
into itself and satisfies the following inequality
(5.1)
for any function
. Then, there exist functions
such that
(5.2)
Proof. By hypothesis, for
fixed, the constant function
,
belongs to
. Since
maps
into itself, we have that
.
From inequality (5.1) and definition of the norm
, we have for
,
and then
(5.3)
Consider
and let
be the equidistant partition defined by
Given
with
, define
by
and
Then, the difference
satisfies that
for all
. Therefore, by the inequality (5.1)
and hence, by definition
(5.4)
From the inequality (5.4), and the definition, it follows that for any partition
of
However, by the definition of
and
, we have that
Then, since
and
, it follows that
Hence, necessarily
So that, we conclude that
satisfies the Jensen equation in
. The continuity of
with respect to the second variable implies that for every
there exists
such that
Since
,
,
and
for each
we obtain that
.
Now we will give the definition of uniformly bounded mapping introduced by J. Matkowski in [24] .
Definition 9 Let
and
be two metric (or normed) spaces. A mapping
is uniformly bounded if, for any
there exists a nonnegative real number
such that for any nonempty set
we have
With the same kind of argument than in ( [23] , Theorem 7), we can see that any uniformly bounded composition operator acting between general Lipschitz function normed space must be of the form (5.2):
Theorem 7 Let
and
the composition operator associated to
. Suppose that
maps
into itself and it is uniformly continuous, then there exists functions
, such that
Proof. It follows as ( [23] , Theorem 7) by Theorem 6.
6. Absolutely Continuous Functions
We now define the analog of absolute p-continuous functions of order two in the framework of variable space.
Definition 10 Given a function
, by modulus of
-continuity of order two of a function
, we mean
where the supremum is taken over all tagged partitions
of the interval
together with a finite sequence of numbers
subject to the conditions
for each
such that the norm of
is at most
.
Lemma 3 Let
be an admissible function. The modulus of
- continuity of order two is a sub-additive function.
Proof. Let
.
If
and
, we say that
is absolutely
-continuous of order two, that is,
.
Theorem 8 Let
be an admissible function. Then
is a closed subspace of
.
Proof. We take a sequence
of functions in
such that
(6.1)
By the sub-additivity of
we have that
Moreover, since
and
,
using Proposition 2.3. in [21] and the strong limit (6.1) we have that, for each
fixed
,
when
. Since
when
by hypothesis, we obtain that
when
.
Acknowledgments
We thank the editor and the referee for their comments. We thank also the anonymous comments to correct and improve this research. It has been partially supported by the Central Bank of Venezuela. We want to give thanks also to the library staff of B.C.V for compiling the references.