Reflected BSDEs Driven by Lévy Processes and Countable Brownian Motions ()
Received 25 March 2015; accepted 20 December 2015; published 23 December 2015

1. Introduction
Recently, Y. Ren [1] proved via the Snell envelope and the fixed point theorem, the existence and uniqueness of a solution for the following RBDSDEs driven by a Lévy process and a extra Brownian motion with Lipschitz coefficients, where the obstacle process is right continuous with left limits (càdlàg):

where the 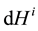 is a forward semi-martingale Itô integrals (see He et al. [2] ) and the
is a forward semi-martingale Itô integrals (see He et al. [2] ) and the 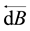 is a backward Itô integral.
is a backward Itô integral.
Note that, in all the previous works, the equations are driven by finite Brownian motions. In their recent work, Pengju Duan et al. [3] introduced firstly the reflected BDSDEs driven by countable extra Brownian motions:
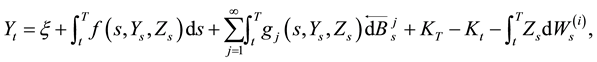 (1.1)
(1.1)
where the dW is the standard forward stochastic Itô integral and the 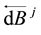 is the backward stochastic Itô integral. Under the global Lipschitz continuity conditions on the coefficients f and g, they proved via Snell envelope and fixed point theorem, the existence and uniqueness of the solution for RBDSDEs (1.1). Next, J.-M. Owo [4] relaxed the Lipschitz continuity condition on the coefficient f to a continuity with sub linear growth condition and derive the existence of minimal and maximal solutions to RBSDEs (1.1).
is the backward stochastic Itô integral. Under the global Lipschitz continuity conditions on the coefficients f and g, they proved via Snell envelope and fixed point theorem, the existence and uniqueness of the solution for RBDSDEs (1.1). Next, J.-M. Owo [4] relaxed the Lipschitz continuity condition on the coefficient f to a continuity with sub linear growth condition and derive the existence of minimal and maximal solutions to RBSDEs (1.1).
Motivated by [1] [3] [4] , in this paper, we mainly consider the following RBDSDEs driven by a Lévy process and countable Brownian motions, in which the obstacle process is right continuous with left limits (càdlàg):
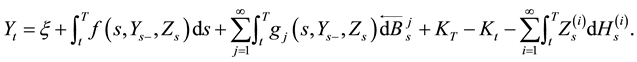 (1.2)
(1.2)
The paper is devoted to prove the existence and uniqueness of a solution for RBSDEs driven by a Lévy process and countable Brownian motions.
The paper is organized as follows. In section 2, we give some preliminaries and notations. In section 3, we establish the main results.
2. Preliminaries and Notations
Throughout this paper, T is a positive constant and 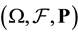 is a probability space on which,
is a probability space on which,  are mutual independent one-dimensional standard Brownian motions and
are mutual independent one-dimensional standard Brownian motions and 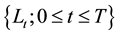 be a
be a 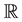 -valued pure jump Lévy process of the form
-valued pure jump Lévy process of the form 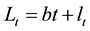 independent of
independent of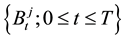 , which correspond to a standard Lévy
, which correspond to a standard Lévy
measure 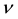 satisfying
satisfying 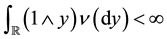 and
and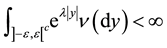 , for every
, for every ![]() and for some
and for some![]() .
.
Let ![]() denote the class of P-null sets of
denote the class of P-null sets of![]() . For each
. For each![]() , we define
, we define
![]()
where for any process![]() ;
;![]() ,
,![]() .
.
Note that ![]() is an increasing filtration and
is an increasing filtration and ![]() is a decreasing filtration. Thus the
is a decreasing filtration. Thus the
collection ![]() is neither increasing nor decreasing so it does not constitute a filtration.
is neither increasing nor decreasing so it does not constitute a filtration.
Let us introduce some spaces:
・ ![]() denotes the space of real-valued processes
denotes the space of real-valued processes ![]() such that
such that ![]() is
is ![]() -measurable, for a.e.
-measurable, for a.e. ![]() and
and![]() .
.
・ ![]() denotes the sub set of
denotes the sub set of ![]() formed by the
formed by the ![]() -predictable processes;
-predictable processes;
・ ![]() stands for the set of real-valued, càdàg, random processes
stands for the set of real-valued, càdàg, random processes ![]() such that
such that ![]() is
is ![]() - measurable, for any
- measurable, for any ![]() and
and![]() .
.
・ ![]() denotes the space continuous, real-valued, increasing processes
denotes the space continuous, real-valued, increasing processes![]() , such that
, such that ![]() is
is ![]() - measurable, for a.e.
- measurable, for a.e.![]() ,
, ![]() and
and![]() .
.
・ ![]() denotes the set of real valued sequences
denotes the set of real valued sequences ![]() such that
such that ![]()
We will denote by ![]() and
and ![]() the corresponding spaces of
the corresponding spaces of ![]() -valued processes
-valued processes ![]() such that
such that
![]()
In the sequel, for ease of notation, we set![]() .
.
Furthermore, we denote by ![]() the Teugels Martingale associated with the Lévy process
the Teugels Martingale associated with the Lévy process![]() . More precisely
. More precisely
![]()
where ![]() for all
for all ![]() and
and ![]() are power-jump processes. That is,
are power-jump processes. That is, ![]() and
and ![]() for
for![]() , with
, with![]() .
.
In [5] , Nualart and Schoutens proved that the coefficients ![]() correspond to the orthonormalization of the polynomials
correspond to the orthonormalization of the polynomials ![]() with respect to the measure
with respect to the measure![]() , i.e.
, i.e. ![]() . The martingale
. The martingale ![]() can be chosen to be pairwise strongly orthonormal martingale. That is, for all
can be chosen to be pairwise strongly orthonormal martingale. That is, for all![]() ,
,![]() .
.
Definition 2.1. A solution of a (1.2) is a triplet of ![]() -valued process
-valued process![]() , which satisfies (1.2), and
, which satisfies (1.2), and
1) ![]()
2) ![]()
3) K is a continuous and increasing process with ![]() and
and ![]()
Throughout the paper, we let the coefficients ![]() and
and![]() , the terminal value
, the terminal value ![]() and the obstacle
and the obstacle ![]() satisfying the following assumptions:
satisfying the following assumptions:
(H1) for all![]() ,
, ![]() are
are ![]() -measurable such that
-measurable such that
![]()
(H2) for all ![]() and
and![]() ,
,
![]()
where![]() ,
, ![]() and
and ![]() are constants with
are constants with ![]() and
and![]() .
.
(H3)![]() , i.e.
, i.e. ![]() is a
is a ![]() -measurable random variable such that,
-measurable random variable such that, ![]() ,
,
(H4) S is a real-valued, càdàg process such that ![]() is
is ![]() -measurable, for a.e.
-measurable, for a.e. ![]() and
and ![]() a.s.,
a.s.,
with![]() , where
, where![]() . Moreover, we assume that its jumping times are inaccessible
. Moreover, we assume that its jumping times are inaccessible
stopping times (see He et al. [2] ).
3. The Main Results
We first establish the existence and uniqueness result for RBSDEs driven by finite Brownian motions and a Lévy process:
![]() (3.1)
(3.1)
For any![]() , we have the following existence and uniqueness result.
, we have the following existence and uniqueness result.
Lemma 3.2. Assume (H1) - (H4). Then, there exists a unique solution ![]() of Equation (3.1).
of Equation (3.1).
Proof. For![]() , we obtain the existence and uniqueness result due to Y. Ren [1] . For any
, we obtain the existence and uniqueness result due to Y. Ren [1] . For any![]() , we can prove the desired result following the same ideas and arguments as in Y. Ren [1] : it is a straightforward adaptation of the proofs of Theorem 2 and Theorem 3 in Y. Ren [1] . Firstly, we consider the special case that is the function f and
, we can prove the desired result following the same ideas and arguments as in Y. Ren [1] : it is a straightforward adaptation of the proofs of Theorem 2 and Theorem 3 in Y. Ren [1] . Firstly, we consider the special case that is the function f and ![]() do not depend on (Y, Z), i.e.
do not depend on (Y, Z), i.e.![]() ,
, ![]() , for all
, for all![]() . It suffices to replace suitably
. It suffices to replace suitably ![]() and
and ![]() in the proof of Theorem 2
in the proof of Theorem 2
respectively by ![]() and
and![]() . On the other hand, it suffices to replace
. On the other hand, it suffices to replace
![]() ,
, ![]() , C and
, C and ![]() in the proof of Theorem 3 respectively by
in the proof of Theorem 3 respectively by![]() ,
,
![]() ,
, ![]() and
and![]() . Therefore, we omit the details.
. Therefore, we omit the details.
Now, we are ready to establish the main result of this paper which is the following theorem.
Theorem 3.3. Under assumptions (H1)-(H4), there exists a unique solution ![]() of Equation (1.2).
of Equation (1.2).
Proof. (Existence.) By Lemma 3.1, for any![]() , there exists a unique solution of (3.1), denoted by
, there exists a unique solution of (3.1), denoted by ![]() , i.e.,
, i.e., ![]() and
and
![]() (3.2)
(3.2)
The idea consists to study the convergence of the sequence![]() , and to establish that its limit is a solution of (1.2). To this end, we first establish the following estimates:
, and to establish that its limit is a solution of (1.2). To this end, we first establish the following estimates:
![]() (3.3)
(3.3)
where ![]() is a non-negative constant independent of n. Indeed, applying Itô’s formula to
is a non-negative constant independent of n. Indeed, applying Itô’s formula to![]() , we have
, we have
![]()
From assumption (H2) and Young’s inequality, for any ![]() we have
we have
![]()
![]()
Using again Young inequality, we have for any![]() ,
,
![]()
Since
![]()
we have, for any![]() ,
,
![]()
Therefore,
![]()
![]()
Consequently,
![]()
We choose ![]() such that,
such that, ![]() Then, there exists a constant
Then, there exists a constant![]() , such that
, such that
![]()
Applying Gronwall’s inequality, we get
![]()
Therefore, we have the existence of a constant ![]() such that
such that
![]()
which by Burkhölder-Davis-Gundy’s inequality provides
![]()
Now, we show that ![]() is a Cauchy sequence in
is a Cauchy sequence in![]() . To this end, without loss of generality, we let
. To this end, without loss of generality, we let![]() . Then, by difference, we obtain
. Then, by difference, we obtain
![]() (3.4)
(3.4)
Applying Itô’s formula to![]() , we get
, we get
![]() (3.5)
(3.5)
Taking expectation in both side of (3.5) and noting that![]() , we have
, we have
![]() (3.6)
(3.6)
Using again Young’s inequality, assumption (H2) and the estimates (3.3), we obtain,
![]()
where![]() .
.
Therefore, by Gronwall’s inequality, we have
![]()
which, by Burkholder-Davis-Gundy inequality provides
![]()
Well, from assumptions (H1)-(H2), we have
![]()
Consequently, we get,
![]() (3.7)
(3.7)
Moreover, from (3.4) together with Hölder’s and Burkholder-Davis-Gundy’s inequalities, we have
![]()
which, together with assumption (H2) and (3.7), provides
![]() (3.8)
(3.8)
Consequently, ![]() is a Cauchy sequence in
is a Cauchy sequence in ![]() which is a Banach space. Therefore, there exists a process
which is a Banach space. Therefore, there exists a process![]() , such that
, such that
![]() (3.9)
(3.9)
Now, let us show that the process ![]() satisfies our Equation (1.2). From Cauchy- Schwarz inequality, together with (H2), we have
satisfies our Equation (1.2). From Cauchy- Schwarz inequality, together with (H2), we have
![]()
Also, by Burkhölder-Davis-Gundy’s inequality, we get
![]()
and
![]()
Now, from (H1)-(H2) and the fact that![]() , we have
, we have
![]()
which implies that
![]()
Moreover,
![]()
Therefore,
![]()
On the other hand, from the result of Saisho [6] (see p. 465), we have
![]()
Finally, passing to the limit in (3.2), we conclude that ![]() is a solution of (1.2).
is a solution of (1.2).
(Uniqueness.) Let ![]()
![]() be two solutions of (1.2).
be two solutions of (1.2).
Applying Itô’s formula to![]() , we get
, we get
![]() (3.10)
(3.10)
Taking expectation in both side of (3.10) and noting that![]() , we have
, we have
![]() (3.11)
(3.11)
Using again Young’s inequality ![]() and assumption (H2), we obtain,
and assumption (H2), we obtain,
![]()
Choosing![]() , we have
, we have![]() , a.e., for all
, a.e., for all![]() . So, we have
. So, we have![]() , a.e., for all
, a.e., for all![]() .
.
On the other hand, since,
![]()
we have![]() , a.e., for all
, a.e., for all![]() . Then, we complete the proof.
. Then, we complete the proof.