Exactly Solvable Schrödinger Equation with Hypergeometric Wavefunctions ()
1. Introduction
Exactly and quasi-exactly solvable potential models are important in practically any field of theoretical quantum chemistry and physics for two principal reasons: first, they are useful to understand the behavior of quantum systems and second, can be used as a basis to study problems that can only be treated using perturbative and nonperturbative procedures. In spite of the above, the exactly solvable Schrödinger equations are rather scanty and in their research different analytical or operational approaches have been used. Also, the well known exactly- solvable Scarf, Eckart, Rosen-Morse I and II, Poschl-Teller I and II as well as Hulthén, Manning-Rosen and Woods-Saxon potentials, all they have, as common feature, hypergeometric wavefunctions. Similarly, the harmonic oscillator, Morse, Coulomb or Kratzer potential models have confluent hypergeometric solutions. Consequently, it becomes clear that the exact solution for the Schrödinger equation is reduced to the study of hypergeometric and/or confluent hypergeometric Differential Equations (DE). At this regard, many efforts have been conducted to find the intermapping between different solvable potentials [1] [2] with the aim to give a unified treatment of partner potentials [3] . For example, in the case of potentials with the hypergeometric wavefunctions, the hexagonal diagram proposed by Cooper et al. [4] is very useful to show how all the shape invariant potentials are inter-related. Also, it has been proposed a pre-potential approach to study of Eckart-type potentials [5] and a five-parameter exponential-type potential to unify the treatment of exactly solvable trigonometric potential models [6] -[8] . Furthermore, to find exactly solvable Schrödinger equations different methods based on Supersymetric Quantum Mechanics (SUSY-QM) [9] , Asymptotic Iteration Method (AIM) [10] and on the transformation of a Schrödinger equation into a hypergeometric-type DE by the Nikiforov-Uvarov (NU) [11] approach, have been used. In this work, we present a proposal that can be considered inverse to the NU method. However, instead of transforming a DE into a Schrödinger equation we consider the transformation of a general homogeneous linear second order DE to their canonical form. Obviously, the general DE has as particular cases the hypergeometric and confluent hypergeometric DE. For that reason, this work aims at finding solvable potentials with hypergeometric wavefunctions leaving the treatment of models with confluent hypergeometric solutions elsewhere [12] . Thus, the proposed approach to transform a general DE into a Schrödinger-like equation is given in next section by means of the canonical transformation method given in the Appendix. The application of the present proposal is given in Section 3, where we consider the hypergeometric DE by means of an auxiliary function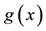 , defined in Section 2, that indicates the required transformation. That is, as will be shown, each possibility of
, defined in Section 2, that indicates the required transformation. That is, as will be shown, each possibility of 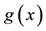 leads to different generalized potentials which are reduced to well known particular cases. Finally, in Section 4 we presents the concluding remarks emphasizing that our proposition is general for which can be directly applied to other important DE.
leads to different generalized potentials which are reduced to well known particular cases. Finally, in Section 4 we presents the concluding remarks emphasizing that our proposition is general for which can be directly applied to other important DE.
2. Transformation of a General DE into a Schrödinger-Like Equation
According to the proposition given in Equation (A5), the generalized canonical transformation of Equation (A1) becomes
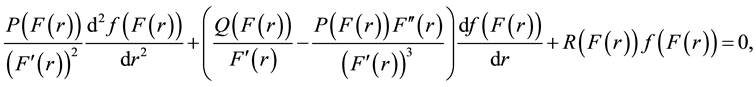 (1)
(1)
that can be rewritten as
 (2)
(2)
where
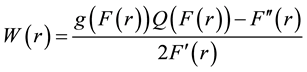 (3)
(3)
and
 (4)
(4)
that will be referred as auxiliary function hereafter. Consequently, from the above, the variable r is given by
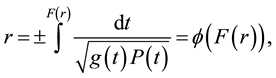 (5)
(5)
in such a way that Equation (A5) is feasible on condition to have the inverse function
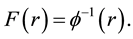 (6)
(6)
Furthermore, the general transform given in Equation (A6) can be rewritten as
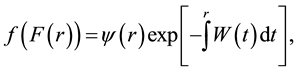 (7)
(7)
where we have used the auxiliary function and the fact that
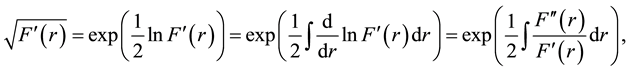 (8)
(8)
such that
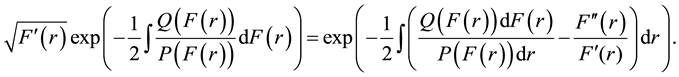 (9)
(9)
Consequently, by substituting Equation (7) into Equation (2), the generalized canonical form of Equation (A1) will be
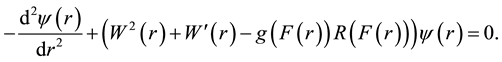 (10)
(10)
At this point, it should be noticed that above equation can be identified with a Schrödinger-like equation where the 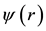 wavefunction can be obtained from Equation (7) on condition that coefficients
wavefunction can be obtained from Equation (7) on condition that coefficients ![]() and the solution
and the solution ![]() of Equation (A1) are known. Besides, the potential and energy spectra are given in general by
of Equation (A1) are known. Besides, the potential and energy spectra are given in general by
![]() (11)
(11)
being ![]() and
and ![]() a set of parameters that come from Equation (A1) and Equation (5) respectively.
a set of parameters that come from Equation (A1) and Equation (5) respectively.
In short, it is worth noting that ![]() is involved in the construction of potential
is involved in the construction of potential ![]() as well as in its corresponding solutions
as well as in its corresponding solutions ![]() given by Equation (7) through the function
given by Equation (7) through the function![]() . Also, according to Equation (4), there will be different transformations
. Also, according to Equation (4), there will be different transformations ![]() depending on the selection of the auxiliary function
depending on the selection of the auxiliary function ![]() which means the existence of various exactly-solvable potentials having the same wavefunctions of the former DE.
which means the existence of various exactly-solvable potentials having the same wavefunctions of the former DE.
3. Application to the Hypergeometric DE
To show the usefulness of the approach given in above section, in the search of exactly-solvable Schrödinger equations, let us apply the above results to the hypergeometric DE
![]() (12)
(12)
whose solution is [13]
![]() (13)
(13)
where ![]() is the Pochhammer symbol and parameters a and b are constant with
is the Pochhammer symbol and parameters a and b are constant with ![]() That is, in this case Equation (12) matches with Equation (A1) provided that
That is, in this case Equation (12) matches with Equation (A1) provided that ![]()
![]() and
and ![]()
As mentioned before, depending on the choice of![]() , or their corresponding auxiliary function
, or their corresponding auxiliary function![]() , our proposal leads to different exactly-solvable potentials. Consequently, the different options for
, our proposal leads to different exactly-solvable potentials. Consequently, the different options for ![]() can be obtained by Equation (3) given by
can be obtained by Equation (3) given by
![]() (14)
(14)
where we have used the explicit form of function![]() . So, in order to have at least one constant term associated to the eigenvalue
. So, in order to have at least one constant term associated to the eigenvalue ![]() of Equation (11), one option is to propose the following cases:
of Equation (11), one option is to propose the following cases:
![]()
![]() and
and ![]() So, by using Equation (4), the first option leads to
So, by using Equation (4), the first option leads to![]() , while the second implies
, while the second implies ![]() and the third option gives
and the third option gives ![]() Another possibility arises when
Another possibility arises when ![]() which means
which means
![]() since
since![]() . All these options are considered explicitly in what follows by using
. All these options are considered explicitly in what follows by using ![]() instead of
instead of![]() .
.
3.1. Generalized Potentials from g(x) Constant
Let us consider the identity
![]() (15)
(15)
to rewrite Equation (5) as
![]() (16)
(16)
with the purpose to use the integral [14]
![]() (17)
(17)
That is, the above integral and the choice of ![]() as constant given by
as constant given by
![]() (18)
(18)
allows to evaluate the integral of Equation (16) as
![]() (19)
(19)
Consequently, in this case the corresponding transformation is
![]() (20)
(20)
leading to the particular cases + and − specified by
![]() (21)
(21)
Thus, according to Equation (3), ![]() becomes
becomes
![]() (22)
(22)
which means
![]() (23)
(23)
and
![]() (24)
(24)
Thus, as mentioned before, the potential function ![]() and the eigenvalue
and the eigenvalue ![]() can be identified from Equation (11) as
can be identified from Equation (11) as
![]() (25)
(25)
leading to the corresponding generalized potentials ![]()
![]() (26)
(26)
with eigenvalues
![]() (27)
(27)
and eigenfunctions
![]() (28)
(28)
Also, in order to have physically acceptable wavefunctions, the original parameters a, b and c are redefined as
![]() (29)
(29)
with![]() , for which the potentials of Equation (26) become
, for which the potentials of Equation (26) become
![]() (30)
(30)
as well as their respective wavefunctions
![]() (31)
(31)
with energy spectra
![]() (32)
(32)
At this point, is important to notice that particular cases
![]() (33)
(33)
and
![]() (34)
(34)
are identified with the well known trigonometric ![]() and hyperbolic
and hyperbolic ![]() Scarf potentials having as eigenfunctions
Scarf potentials having as eigenfunctions
![]() (35)
(35)
and
![]() (36)
(36)
respectively, where ![]() is proportional to the
is proportional to the ![]() Jacobi polynomials. As a consequence the following question arises: From where comes the Posch-Teller potential? The answer to that question follows from the negative of
Jacobi polynomials. As a consequence the following question arises: From where comes the Posch-Teller potential? The answer to that question follows from the negative of ![]() given in Equation (18) but now along with the integral [14]
given in Equation (18) but now along with the integral [14]
![]() (37)
(37)
As can be proved, the plus (+) case leads to ![]() given in Equation (33). So, we are going to consider only the minus (−) case. That is, now Equation (16) gives rise to
given in Equation (33). So, we are going to consider only the minus (−) case. That is, now Equation (16) gives rise to
![]() (38)
(38)
which indicates that the corresponding transformation is
![]() (39)
(39)
Then, according to Equation (3), in this case ![]() will be
will be
![]() (40)
(40)
leading, from Equation (11), to the potential
![]() (41)
(41)
with eigenvalue
![]() (42)
(42)
and eigenfunction
![]() (43)
(43)
Finally, using the conditions for physically acceptable wavefunctions one obtains
![]() (44)
(44)
that leads to the potential
![]() (45)
(45)
with eigenfunctions
![]() (46)
(46)
and energy spectra
![]() (47)
(47)
that coincides with the well known Posch-Teller potential.
3.2. Generalized V(r) from g(x) Proportional to P(x)
Similarly to the cases considered in above section, the choice of
![]() (48)
(48)
can be worked by means of the integrals
![]() (49)
(49)
In fact, the use of Equation (49) lets write Equation (16) as
![]() (50)
(50)
leading to the corresponding transformation
![]() (51)
(51)
So, according to Equation (3)
![]() (52)
(52)
Thus, the potential function V(r) and the eigenvalue E can be identified from Equation (11) as
![]() (53)
(53)
to obtain the corresponding generalized potentials
![]() (54)
(54)
with eigenvalue
![]() (55)
(55)
and wavefunction
![]() (56)
(56)
As before, with the aim to have physically acceptable solutions it becomes necessary to redefine the original parameters a, b and c as follow
![]() (57)
(57)
with ![]() and
and ![]() This fact, leads to
This fact, leads to
![]() (58)
(58)
with their corresponding wavefunctions
![]() (59)
(59)
and energy spectra
![]() (60)
(60)
where
![]() (61)
(61)
At this point, is important to notice that potential ![]() given by
given by
![]() (62)
(62)
corresponds to the Rosen-Morse II hyperbolic potential and
![]() (63)
(63)
to the exactly solvable Eckart potential. Also, we want to point out that ![]() and
and ![]() are isospectral, accordingly to Equation (60), with wavefunctions
are isospectral, accordingly to Equation (60), with wavefunctions
![]() (64)
(64)
and
![]() (65)
(65)
respectively. Similarly to the cases analyzed in section III.1), the question is now; How can obtain the Rosen-Morse II trigonometric potential? To answer that question, we are going to use the same choice of ![]() but now proportional to the negative
but now proportional to the negative![]() . That is,
. That is,
![]() (66)
(66)
that together with Equation (49) in Equation (16) permit us to obtain
![]() (67)
(67)
Thus, with the aid of the identity
![]() (68)
(68)
the corresponding transformation is
![]() (69)
(69)
Consequently, according to Equation (3)
![]() (70)
(70)
such that
![]() (71)
(71)
leading to
![]() (72)
(72)
with eigenvalue
![]() (73)
(73)
and eigenfunction
![]() (74)
(74)
Similarly, to have physically acceptable wavefunctions the original parameters a, b and c are defined as
![]() (75)
(75)
where ![]() and p a new real parameter. Consequently, the potentials are corrected as
and p a new real parameter. Consequently, the potentials are corrected as
![]() (76)
(76)
with energy spectra
![]() (77)
(77)
and eigenfunctions
![]() (78)
(78)
where
![]() (79)
(79)
Specifically, the ![]() and
and ![]() are given by
are given by
![]() (80)
(80)
and
![]() (81)
(81)
with their respective wavefunctions
![]() (82)
(82)
and
![]() (83)
(83)
with the same energy spectra. At this point, we want to notice that potential ![]() corresponds, as expected, to the exactly solvable Rosen-Morse II trigonometric potential and
corresponds, as expected, to the exactly solvable Rosen-Morse II trigonometric potential and ![]() seems to be a new exactly solvable potential.
seems to be a new exactly solvable potential.
3.3. A class of Multiparameter Exponential-Type Potential
a). Option with![]() .
.
According to the third option for ![]() in this case one have
in this case one have
![]() (84)
(84)
In fact, by choosing the minus sign in Equation (5) and the down integral limit as a new parameter q, one obtains
![]() (85)
(85)
from which
![]() (86)
(86)
being ![]() an additional set of new parameters. In consequence, from Equation (3),
an additional set of new parameters. In consequence, from Equation (3), ![]() is given by
is given by
![]() (87)
(87)
leading, from Equation (11), to
![]() (88)
(88)
Thus, by defining
![]() (89)
(89)
and
![]() (90)
(90)
it becomes possible to identify the potential
![]() (91)
(91)
the eigenvalue
![]() (92)
(92)
and, from Equation (7), the eigenfunction
![]() (93)
(93)
In what follow, the parameters a,b and c will be calculated by considering the boundary conditions of the system in order to have physically acceptable wavefunctions. For example, by combining Equation (89) and Equation (90) one obtains
![]() (94)
(94)
with
![]() (95)
(95)
Thus, to have a node in ![]() is necessary to apply the condition
is necessary to apply the condition ![]() in Equation (93) with
in Equation (93) with ![]() the singular point of
the singular point of![]() , which is achieved if
, which is achieved if
![]() (96)
(96)
and
![]() (97)
(97)
On the other hand, by using Equation (94) in Equation (89) we obtain
![]() (98)
(98)
and
![]() (99)
(99)
Also, since the hypergeometric function of Equation (93) is an infinite series, the condition
![]() (100)
(100)
leads to a polynomial of n degree in the variable![]() . Besides,
. Besides, ![]() when
when ![]() indicates
indicates
that ![]() in the first exponent of Equation (93) which gives the number of states for the system
in the first exponent of Equation (93) which gives the number of states for the system
![]() (101)
(101)
These assumptions on the original parameters {a, b, c} assure that boundary conditions are fulfilled, leading to a physically acceptable wavefunction for the Schrödinger equation under consideration. Additionally, the energy spectra for the potential ![]() is obtained by using Equation (99) in Equation (92). That is
is obtained by using Equation (99) in Equation (92). That is
![]() (102)
(102)
with the corresponding wavefunctions
![]() (103)
(103)
It should be pointed out that in this case the potential V(r) has a minimum value
![]() (104)
(104)
in
![]() (105)
(105)
on condition that
![]() (106)
(106)
since in this case the argument of the logarithm function, given in Equation (105), is always positive. Consequently, Equations (104)-(106) ensures that potential V(r) is attractive with a infinite wall in![]() . In short, the potential given in Equation (91) is general and contains as particular cases exactly-solvable potentials for specific
. In short, the potential given in Equation (91) is general and contains as particular cases exactly-solvable potentials for specific
values of A, B, and C. For example, the choice of ![]()
![]() and
and ![]() lead to the exactly-solvable potential
lead to the exactly-solvable potential
![]() (107)
(107)
which is identified with the standard Hulthén potential with eigenvalues [15] [16]
![]() (108)
(108)
and eigenfunctions
![]() (109)
(109)
In a similar way, the selection ![]() and
and ![]() permit us to obtain the supersymmetric or generalized
permit us to obtain the supersymmetric or generalized
Hulthén potential already given by Morales et al., [17] . Also, the selection ![]()
![]()
![]()
![]() and
and ![]() gives rise to the Manning-Rosen potential [18]
gives rise to the Manning-Rosen potential [18]
![]() (110)
(110)
with energy spectra
![]() (111)
(111)
and wavefunctions
![]() (112)
(112)
Another important exactly-solvable exponential potential that have hypergeometric wavefunctions is the Woods-Saxon potential which has been worked using the Numerov method for the standard model [19] or by means of the Nikiforov-Uvarov procedure for the generalized case [20] . According to our results, one can show that the generalized Woods-Saxon potential is obtained as particular case of our proposal when choosing ![]()
![]()
![]() and
and![]() , where
, where![]() , namely [21] ,
, namely [21] ,
![]() (113)
(113)
However, due to the fact that the approach considered until now in this work is consistent with![]() , in the case
, in the case ![]() some conditions have to be considered affecting wavefunctions and energy spectra as well as coordinates. That is, for q negative, it must be necessary to change
some conditions have to be considered affecting wavefunctions and energy spectra as well as coordinates. That is, for q negative, it must be necessary to change ![]() in the transformation
in the transformation![]() , which means that r is no longer the radial variable. Also, the conditions on the original parameters a, b and c, given in Eqs.(94-99), will be corrected to
, which means that r is no longer the radial variable. Also, the conditions on the original parameters a, b and c, given in Eqs.(94-99), will be corrected to![]() , that is,
, that is,
![]() (114)
(114)
with
![]() (115)
(115)
and
![]() (116)
(116)
as well as
![]() (117)
(117)
Thus, by using Equation (114) and the negative of Equation (89) we obtain
![]() (118)
(118)
and
![]() (119)
(119)
with the condition ![]() for
for ![]() Thus, with these new parameters, the energy spectra and wavefunctions of the generalized Wood-Saxon potential are respectively
Thus, with these new parameters, the energy spectra and wavefunctions of the generalized Wood-Saxon potential are respectively
![]() (120)
(120)
and
![]() (121)
(121)
b). Option with![]() .
.
Similarly to the above case, in this new situation one have
![]() (122)
(122)
that leads to the transformation
![]() (123)
(123)
where ![]() and
and
![]() (124)
(124)
that, from Equation (3), lets write
![]() (125)
(125)
In this new situation, by considering the identities
![]() (126)
(126)
and
![]() (127)
(127)
as well as
![]() (128)
(128)
one find the multiparameter exponential-type potential
![]() (129)
(129)
with eigenvalue
![]() (130)
(130)
and eigenfunction
![]() (131)
(131)
At first glance, the potential of Equation (129) can be considered as a new one and however it should be noticed that it can be written as
![]() (132)
(132)
That is, the above ![]() has the same structure of potential given in Equation (91) which means that leads to similar results to those shown in Section 3.3.a). Also, thinking in molecular physics applications, the usefulness of the proposed exactly solvable multiparameter exponential-type potential given in Equation (91) is focused to obtain vibrational modes of diatomic molecules. At this regard, for the study of bound states solutions, one option is a redefinition of parameter B in order to include the centrifugal term and then to apply some of the
has the same structure of potential given in Equation (91) which means that leads to similar results to those shown in Section 3.3.a). Also, thinking in molecular physics applications, the usefulness of the proposed exactly solvable multiparameter exponential-type potential given in Equation (91) is focused to obtain vibrational modes of diatomic molecules. At this regard, for the study of bound states solutions, one option is a redefinition of parameter B in order to include the centrifugal term and then to apply some of the
approximation schemes proposed to![]() . For example, one can consider
. For example, one can consider ![]() and
and ![]() with
with ![]() and so on; see for example Falaye et al. [22] . Moreover, in the same way,
and so on; see for example Falaye et al. [22] . Moreover, in the same way,
other particular radial potentials can be derived from our proposal as, for example, the recently considered Manning-Rosen potential [23] which, as explained before, comes from Equation (91).
4. Concluding Remarks
In this paper, we present a method to obtain the general canonical form of second order differential equations on the field of theoretical physics. The procedure is similar to the method proposed by Levai [24] and however we consider a general DE to be converted into a Schrödinger-like equation. The algorithm we propose, makes use of an auxiliary function ![]() to find the needed transformation to obtain the exactly-solvable potentials with the same wavefunctions of the original DE. To show the usefulness of the proposed method, their application to the hypergeometric DE along with different forms of
to find the needed transformation to obtain the exactly-solvable potentials with the same wavefunctions of the original DE. To show the usefulness of the proposed method, their application to the hypergeometric DE along with different forms of ![]() is given explicitly. Thus, for each
is given explicitly. Thus, for each ![]() we found a generalized exactly-solvable potential from where well known potential models are obtained as particular cases. For example, the choice
we found a generalized exactly-solvable potential from where well known potential models are obtained as particular cases. For example, the choice ![]() gives place to the trigonometric and hyperbolic Scarf potentials as well as to the Posh-Teller potential, while the choice of
gives place to the trigonometric and hyperbolic Scarf potentials as well as to the Posh-Teller potential, while the choice of ![]() proportional to
proportional to ![]() leads to the Eckart and to the trigonometric and hyperbolic Rosen-Morse potentials. Similarly, with the choice of
leads to the Eckart and to the trigonometric and hyperbolic Rosen-Morse potentials. Similarly, with the choice of ![]() we obtain a generalized exactly-solvable multiparameter (
we obtain a generalized exactly-solvable multiparameter (![]() and k) exponential-type potential that contains, depending on the parameters selection, the particular cases of the Hulthén, Manning-Rosen and Wood-Saxon potentials, standard and generalized, among others. Besides, the proposed multiparameter exponential-type potential can be used in the search of bound state solutions of specific potential models of diatomic molecules in the frame of different approaches to the centrifual term [25] . In short, with the application given in this work we have shown that it is possible to give a unified treatment of exactly-solvable potentials having hypergeometric wavefunctions as common feature. Finally, we want to notice that the method is general for which can be applied to other DE as for example on the position-dependent mass Schrödinger equation. Furthermore, advantageously, our proposal avoids the use of specialized solution methods applied to particular potential models as has been usually done.
and k) exponential-type potential that contains, depending on the parameters selection, the particular cases of the Hulthén, Manning-Rosen and Wood-Saxon potentials, standard and generalized, among others. Besides, the proposed multiparameter exponential-type potential can be used in the search of bound state solutions of specific potential models of diatomic molecules in the frame of different approaches to the centrifual term [25] . In short, with the application given in this work we have shown that it is possible to give a unified treatment of exactly-solvable potentials having hypergeometric wavefunctions as common feature. Finally, we want to notice that the method is general for which can be applied to other DE as for example on the position-dependent mass Schrödinger equation. Furthermore, advantageously, our proposal avoids the use of specialized solution methods applied to particular potential models as has been usually done.
Acknowledgements
This work was partially supported by the projects UAM-A-CBI-2232004 and 009. JGR thanks to the Instituto Politécnico Nacional for the financial support given through the COFAA-IPN project SIP-200150019. JGM acknowledges to the IPN-ESFM, for the hospitality during his PhD studies in Science and Technology.
Appendix
The general second order DE
![]() (A1)
(A1)
is transformed into their standard canonical form [26]
![]() (A2)
(A2)
by means of the transformation
![]() (A3)
(A3)
i.e.
![]() (A4)
(A4)
where apostrophes indicates derivative with respect to the argument.
In order to have a generalized canonical transformation of Equation A1, we consider the variable change
![]() (A5)
(A5)
to obtain the general transformation [27]
![]() (A6)
(A6)
Consequently, one have
![]() (A7)
(A7)
being
![]() (A8)
(A8)
where
![]() (A9)
(A9)
It will be noticed that, for the particular case ![]() Equation (A8) reduces to the expression given in the square parenthesis of Equation (A4).
Equation (A8) reduces to the expression given in the square parenthesis of Equation (A4).
NOTES
*Corresponding author.