Travelling Wave Solutions of Kaup-Kupershmidt Equation Which Describes Pseudo Spherical Surfaces ()
1. Introduction
Many partial differential equations which continue to be investigated due to their role in mathematics and physics exhibit interrelationships with the geometry of surfaces, or submanifolds, immersed in a three-dimensional space [1] . In particular, it has been known for a while that there is a relationship between surfaces of a constant negative Gaussian curvature in Euclidean three-space, the Sine-Gordon Equation and Bäcklund transformations which are relevant to the given equation [2] . Moreover, the original Bäcklund transformation for the Sine-Gor- don Equation is also a simple geometric construction for pseudospherical surfaces [3] -[5] . It is well known that nonlinear complex physical phenomena are related to nonlinear partial differential equations (NLPDEs) which are involved in many fields from physics to biology, chemistry, mechanics, etc.
As mathematical models of the phenomena, the investigation of exact solutions to the NLPDEs reveals to be very important for the understanding of these physical problems. Many mathematicians and physicists have well understood this importance when they decided to pay special attention to the development of sophisticated methods for constructing exact solutions to the NLPDEs. Thus, a number of powerful methods have been presented.
We can cite the inverse scattering transform [6] , the Bäcklund and Darboux transform [7] - [10] , Hirota’s bilinear method [11] , the homogeneous balance method [12] , Jacobi elliptic function method [13] , the tanh method and extended tanh-function method [14] - [20] , F-expansion method [21] - [23] and so on. The notion of conservation laws is important in the study of nonlinear evolution equations (NLEEs) appearing in mathematical physics [24] .
Consider Kaup-Kupershmidt Equation,
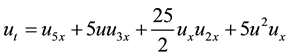(1)
where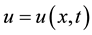 
is a function of two independent variables 
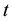and
.
2. Kaup-Kupershmidt Equation Which Describes Pseudo Spherical Surfaces
I recall the definition [25] -[28] of a differential equation (DE) that describes a pss. Let 
be a two dimensional differentiable manifold with coordinates
. A DE for a real function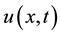 
describes a pss if it is a necessary and sufficient condition for the existence of differentiable functions
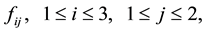(2)
depending on u and its derivatives such that the one-forms
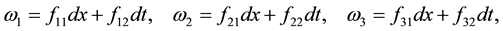(3)
satisfy the structure equations of a pss, i.e.,
(4)
I obtain that the Kaup-Kupershmidt Equation (1) describes pseudospherical surfaces, with associated one forms 
given by
(5)
As a consequence, each solution of the DE provides a local metric on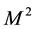
, whose Gaussian curvature is constant, equal to −1. Moreover, the above definition is equivalent to saying that DE for 
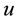is the integrability condition for the problem [19] [29] :
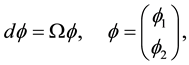(6)
where ![]()
denotes exterior differentiation, ![]()
is a column vector and the ![]()
matrix ![]()
is traceless
![]()
(7)
3. Exact Solution for Kaup-Kupershmidt Equation
With the rapid development of science and technology, the study kernel of modern science is changed from linear to nonlinear step by step. Many nonlinear science problems can simply and exactly be described by using the mathematical model of nonlinear equation. Up to now, many important physical nonlinear evolution equations are found, such as Sine-Gordon Equation, KdV Equations, Schrodinger Equation all possess solitary wave solutions. There exist many methods to seek for the solitary wave solutions, such as inverse scattering method, Hopf-Cole transformation, Miura transformations, Darboux transformation and Bäcklund transformation [7] -[10] , but solving nonlinear equations is still an important task [27] -[30] . In this paper, with the aid of Mathematica, a traveling wave solution for a class of Kaup-Kupershmidt Equation,
![]()
In order to obtain the soliton solution of (1), I will use the modified sine-cosine to develop traveling wave solutions to this equation. The modified sine-cosine method admits the use of solutions [30]
![]()
(8)
and
![]()
(9)
where ![]()
is the soliton amplitude, ![]()
is the width of the soliton, ![]()
is the soliton velocity and ![]()
is constant to be determined later, the unknown index ![]()
will be determined during the course of derivation of the solution of Equation (8). From Equation (8), I obtain
![]()
(10)
From Equation (9), I obtain
![]()
(11)
With the aid of Mathematica or Maple, from (8) and (10), we can get
![]()
(12)
Now, from Equation (12) equating the exponents n − 5 and 2n − 3 leads![]()
, which gives![]()
, such that ![]()
Also from Equation (12) equating the coefficients of like powers of![]()
, ![]()
and ![]()
to zero, I get
![]()
(13)
![]()
(14)
![]()
(15)
Solving the above system by the aid of Wu elimination method [31] , I obtain the three solutions
![]()
(16)
and
![]()
(17)
and
![]()
(18)
Then the soliton solutions of the Kaup-Kupershmidt Equation is given by
![]()
see Figure 1 and Figure 2 (19)
and
![]()
see Figure 3 and Figure 4 (20)
and
![]()
see Figure 5 and Figure 6 (21)
If setting![]()
, then the solutions (19) and (21) are given by
![]()
see Figure 7 and Figure 8 (22)
and
![]()
see Figure 9 and Figure 10 (23)
and
![]()
see Figure 11 and Figure 12 (24)
The double-kink solutions (19), (20), and (21) are characterized by the eigenvalue ![]()
(see Figures 1-6). The solutions (22), (23) and (24) are the single-soliton solutions (see Figures 7-12) corresponding to the eigenvalue![]()
.
4. Conclusions
The new types of exact traveling wave solution obtained in this paper for the Kaup-Kupershmidt Equation will
be of benefit to future studies.
The Soliton Equations play a central role in the field of integrable systems and also play a fundamental role in several other areas of mathematics and physics.
A soliton is a localized pulse-like nonlinear wave that possesses remarkable stability properties. Typically, problems that admit soliton solutions are in the form of evolution equations that describe how some variable or a set of variables evolves in time from a given state. The equations may take a variety of forms, for example, PDEs, differential difference equations, partial difference equations, integro-differential equations, as well as coupled ODEs of finite order.
In this paper, we considered the construction of exact solutions to Kaup-Kupershmidt Equation. I obtain travelling wave solutions for the above equation by using the modified sine-cosine method with the aid of Mathematica.
A travelling wave of permanent form has already been met; this is the solitary wave solution of the nonlinear evolution equation itself. Such a wave is a special solution of the governing equation which does not change its shape and propagates at constant speed.
The soliton phenomena of nonlinear evolution equations represent an important and well-established field of modern physics, mathematical physics and applied mathematics. Solitons are found in various areas of physics from hydrodynamics and plasma physics, nonlinear optics and solid state physics, to field theory and gravitation. NLEEs which describe soliton phenomena have a universal character.
Funding
This research is funded by the Deanship of Research and Graduate Studies in Zarqa University/Jordan.