Permanence and Globally Asymptotic Stability of Cooperative System Incorporating Harvesting ()
1. Introduction
Permanence, stability and periodic solution for LotkaVolterra models had been extensively investigated by many authors (see [1-8] and the references therein). Jorge Rebaza [1] had discussed the dynamic behaviors of predator-prey model with harvesting and refuge
(1)
he obtained that harvesting and refuge affected the stability of some coexistence equilibrium and periodic solutions of model (1), where 
was a continuous threshold policy harvesting function. Motivated by Jorge’s work, we consider the following cooperative system incorporating harvesting
(2)
where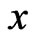 
and 
denote the densities of two populations at time
. The parameters 
are all positive constants.
Definition 1 [2] 
is called asymptotically 
- periodic function, if 
and it satisfies
, where 
is continuous periodic function with periodic 
and
.
We will discuss our problems in the region
where
.
2. Permanence of System
Definition 2 [2] If there are positive constants 
such that each positive solution 
of system (2) satisfies
Then system (2) is persistent. If the system is not persistent, then system (2) is called non-persistent.
Lemma 1 If
, then system (2) is persistent.
Proof. By the first equation of (2) and the comparison theorem, we get 
it implies that
.
For any 
there exists a
, as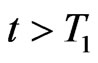
, it then follows
Similarly, we have
. By the discussion above, for any 
there exists a
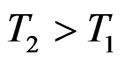, as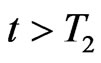
, it yields that 
On the other hand, we have
.
By the comparison theorem, and letting
, one gets that
.
By Definition 2, system (2) is persistent. □
3. Equilibrium Points and Stability
If
, then the equilibrium points of (2) are
where
(3)
.
The general Jacobian matrix of (2) is given by
.
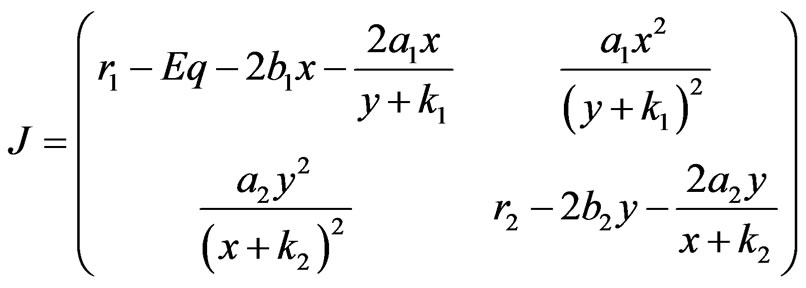The characteristic equation of system (2) at 
is
, this immediately indicates that
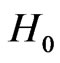is always unstable.
The characteristic equation of system (2) at 
is
, by the condition
, one then gets that 
is a saddle point.
The characteristic equation of system (2) at 
is
, we derive that 
is a saddle point.
The characteristic equation of system (2) at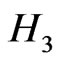 
takes the form
it is easy to check that 
, then 
, thus 
is locally asymptotically stable.
Theorem 1 If 
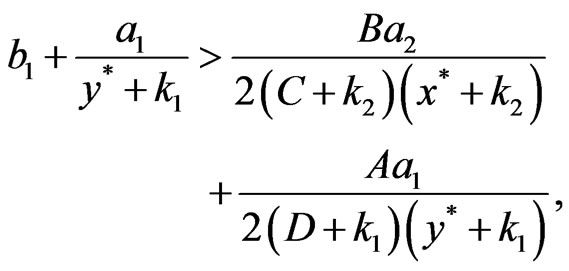
then the positive equilibrium point 
of system (2) is globally asymptotically stable, where 
can be found in Lemma 1.
Proof. Define a Lyapunov function
it then yields that
by the conditions of theorem 1, thus,
. The positive equilibrium point 
of system (2) is globally asymptotically stable.
4. Existence and Uniqueness of Solutions
Next, we will discuss a nonautonomous system
(4)
where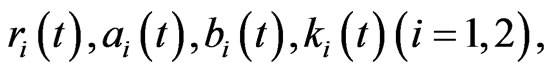 
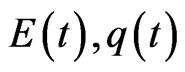are positive continuous bounded asymptotically periodic functions with period
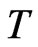. The initial data of (4) is given by
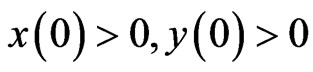. (5)
The solution of (4) with initial data (5) is denoted by
, 
,
.
For a continuous function 
defined on 
define
.
Definition 3 [2] If there exists a
, for any
, 
, there exists a
such that 
for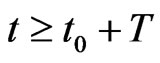
, then the solution
is called ultimately bounded.
Let us consider the following asymptotically periodic system
(6)
where
. Set
,
In order to discuss the existence and uniqueness of asymptotically periodic solution of system (6), we can consider the adjoint system
(7)
Lemma 2 If
and
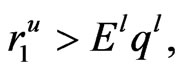
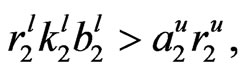
then the solution of system (4) is ultimately boundedness.
Proof. By the first equation of system (4) and the comparison theorem, one gets that
it then implies that
.
Similarly, we have
.
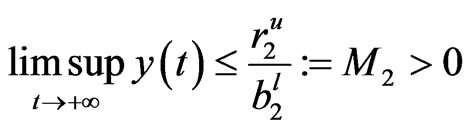By the same discussion, one thus gets that
,
Letting
, we have
.
By the Definition 3, the solution of system (4) is ultimately bounded. □
Lemma 3 [2] If 
satisfies the following conditions:
1)
, where 
and 
are continuously positively increasing functions;
2)
where 
is a constant;
3) there exists a continuous non-increasing function
, such that for s > 0,
. And as
,
it then follows that
where 
is a constant; furthermore, system (6) has a solution 
for 
and satisfies
.
Then system (6) has a unique asymptotically periodic solution, which is uniformly asymptotically stable.
Theorem 2 If conditions
and
hold, the conditions of Lemma 2 are satisfied, then system (4) has a unique asymptotically periodic solution, which is uniformly asymptotically stable.
Proof. By Lemma 2, the solutions of system (4) is ultimately bounded. We consider the adjoint system
(8)
Let
and 
be the solution of (8). By the fact
where 
lies between 
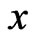and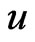
, 
lies between 
and
, it then follows
(9)
Define Lyapunov function
, taking
By suing of the inequality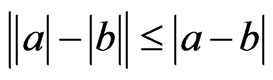
, it is easy to check that 1) and 2) of Lemma 3 are valid. Computing the derivative of 
along the solution of system (8), by (9) and
, we get that
taking
, it yields
, then, system (4) has a unique positive asymptotically periodic solution, which is uniformly asymptotically stable. □
5. Examples and Numerical Simulations
Now, let us consider a autonomous cooperative system incorporating harvesting
, (10)
it is easy to check that
,
,
, 
,
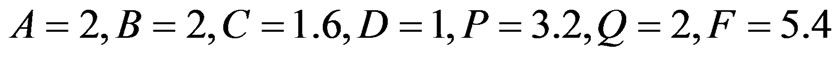the conditions of Theorem 1 are valid, then the positive equilibrium point 
of system (2) is globally asymptotically stable in Figures 1 and 2.
6. Conclusions
By analyzing the characteristic roots of a kind of cooperative models (2) incorporating harvesting, the stability of positive equilibrium point 
to model (2) is obtained by constructing a suitable Lyapunov function. Our results have shown that the harvesting coefficient 
affects the stability and the existence of equilibrium point to model (2).
The related non-autonomous asymptotically periodic cooperative model (4) has been discussed later. Under some conditions, which also depend on model parameters (see Theorem 2), model (4) has a unique asymptotically periodic solution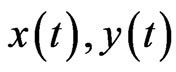
, which is uniformly
Figure 1. Positive equilibrium point 
of (2) is globally asymptotically stable.
Figure 2. Solution of (2) is uniformly asymptotically stable.
asymptotically stable. Example model (10) shows the effectiveness of our results.
7. Acknowledgements
Our work is supported by Natural Science Foundation of China (11201075), the Natural Science Foundation of Fujian Province of China (2010J01005).