Overlapping Nonmatching Grid Method for the Ergodic Control Quasi Variational Inequalities ()
1. Introduction
The Schwarz alternating method can be used to solve elliptic boundary value problems on domains which consist of two or more overlapping sub domains.
The solution is approximated by an infinite sequence of functions which results from solving a sequence of elliptic boundary value problems in each of the sub domain.
In this paper, we are interested in the error analysis in the maximum norm for the obstacle problem in the context of overlapping nonmatching grids: we consider a domain Ω which is the union of two overlapping subdoains where each sub domain has its own triangulation. This kind of discretizations is very interesting as they can be applied to solving many practical problems which cannot be handled by global discretizations. They are earning particular attention of computational experts and engineers as they allow the choice of different mesh sizes and different orders of approximate polynomials in different sub domains according to the different properties of the solution and different requirements of the practical problems.
We study a new approach for the finite element approximation for the ergodic problem where the obstacle is related to a solution. We consider a domain which the union of two overlapping sub-domains where each sub domain has its own generated triangulation. The grid points on the sub-domain boundaries need not much the grid points from the other sub-domains. Under a discrete maximum principle, we show that the discretization on each sub-domain converges quasi-optimally in the  norm.
norm.
In the first section we study the Schwarz method for the ergodic control Quasi-variational inequalities; we state the continuous alternating Schwarz sequence for quasivariational inequalities, and define their respective finite element counterparts in the context of overlapping grids.
In Section 2, we give a simple proof for the main result concerning error estimates in the  norm for the problem studied, taking into account the combination of Jinping Zeng & Shuzi Zhou [2] geometrical convergence and P. L. Lions, B. Perthame [3] quasi-variational inequalities and ergodic impulse control.
norm for the problem studied, taking into account the combination of Jinping Zeng & Shuzi Zhou [2] geometrical convergence and P. L. Lions, B. Perthame [3] quasi-variational inequalities and ergodic impulse control.
2. Schwarz Method for the Ergodic Control Quasi-Variational Inequalities
We begin by down a classical results related to ergodic control quasi-variational inequalities [1-18].
It is well known that impulse control problems for reflected diffusion process may be solved by considering the solution of quasi variational inequalities (QVI) (see Bensoussan [4], A. Bensoussan and J. L. Lions [5]). A typical example is the following:
 (1)
(1)
where Ω is given bounded smooth open set in ,
,  , f is given function, the cost function
, f is given function, the cost function  represents the obstacle of impulse control defined by:
represents the obstacle of impulse control defined by:
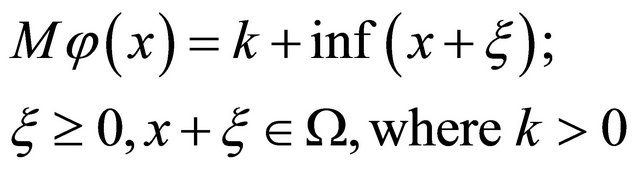 (2)
(2)
where k is a positive number, M is an operator defined on  and assumed to map into itself, that is,
and assumed to map into itself, that is,
 whenever
whenever 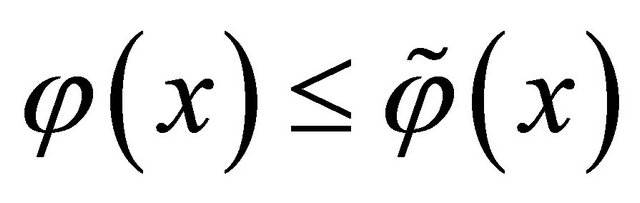 (3)
(3)
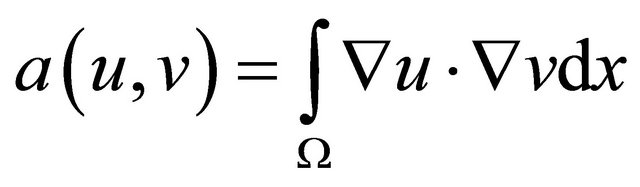 ; and (.,.) denotes the inner product on Ω.
; and (.,.) denotes the inner product on Ω.
It has been proved that the long run average cost for this problem solves the ergodic QVI. More precisely, denoting by:
 and
and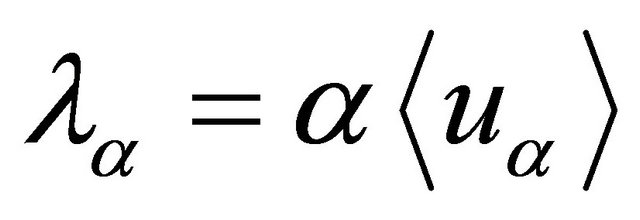 .
.
P. L. Lions and B. Perthame [3] proved that the solution  of the QVI :
of the QVI :

(4)
converges to the solution of the ergodic control QVI :
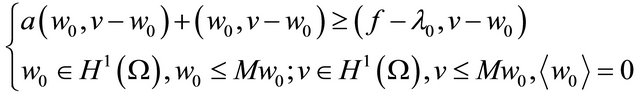
(5)
As stated in the following theorem.
Theorem 1 [1]: As 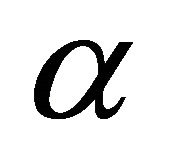 goes to
goes to ,
, converges uniformly in
converges uniformly in  to some constant
to some constant , and
, and  converges uniformly in
converges uniformly in  and strongly in
and strongly in  to
to . Moreover
. Moreover 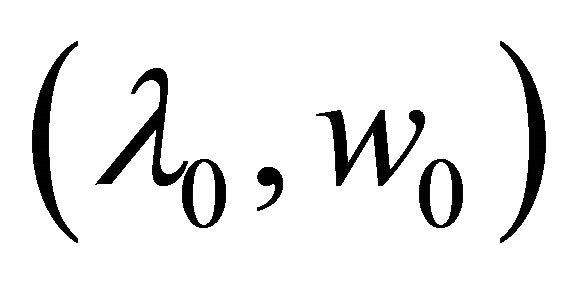 is the unique solution of the quasi variational inequality of the ergodic control problem (5).
is the unique solution of the quasi variational inequality of the ergodic control problem (5).
Problem Position
Let  be fixed in the open interval
be fixed in the open interval  and set. Then, one can easily see that problem (1) is equivalent to the following QVI:
and set. Then, one can easily see that problem (1) is equivalent to the following QVI:
 (6)
(6)
where
 , and
, and 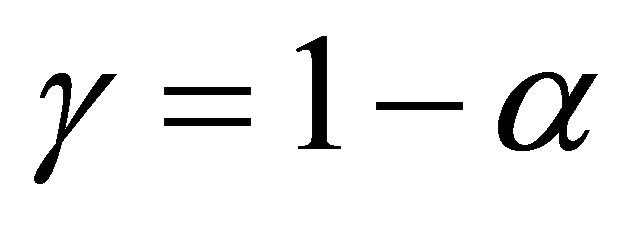 (7)
(7)
Thanks to [5], (1) or (6) has a unique solution. Also, notice that, as the bilinear form (7) is independent of , the left hand side of (6) is independent of
, the left hand side of (6) is independent of  too.
too.
3. The Schwarz Method for the Obstacle
We decompose  into two overlapping polygonal sub domains
into two overlapping polygonal sub domains  and
and , such that
, such that
 (8)
(8)
and  satisfies the local regularity condition
satisfies the local regularity condition
 (9)
(9)
We denote by  the boundary condition of
the boundary condition of  and
and  the intersection of
the intersection of  and
and  is assumed to be empty.
is assumed to be empty.
3.1. The Schwarz Sequences for Problem (4)
We denote by:
 and
and  (10)
(10)
Choosing  such that
such that  the unique solution of:
the unique solution of:
 (11)
(11)
We respectively define 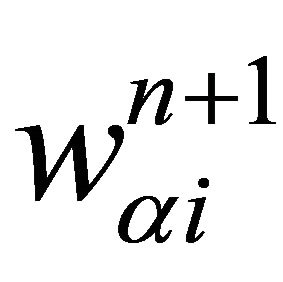 the alternating Schwarz sequences on
the alternating Schwarz sequences on  such that:
such that:

(12)
where .
.
3.2. The Continuous Schwarz Sequences for Principal Problem
We consider the following problem:
 (13)
(13)
Choosing , solution of:
, solution of:
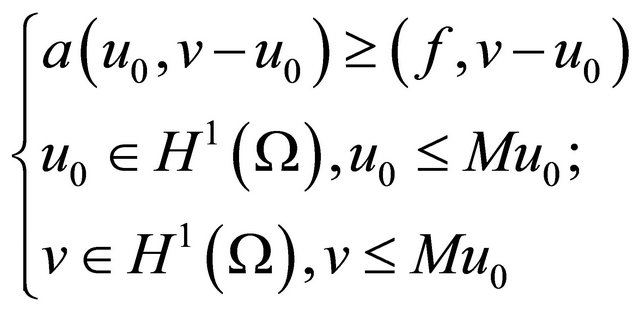 (14)
(14)
We respectively define the alternating Schwarz sequences  on
on  such that:
such that:  and;
and;
 (15)
(15)
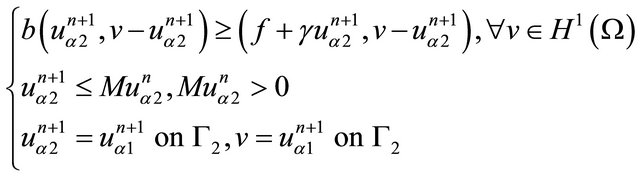 (16)
(16)
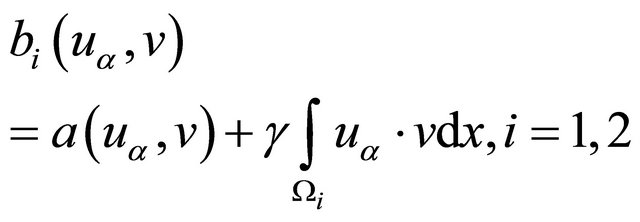 (17)
(17)
Lemma 1 [3]: for each 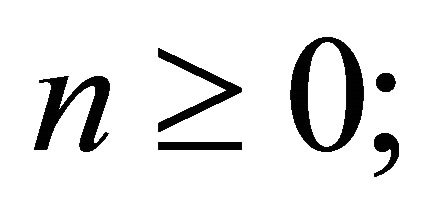
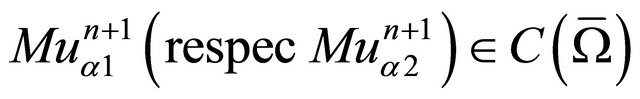
4. The Discret Problem
We suppose for simplicity that  is polyhedral. Let
is polyhedral. Let  be a regular, quasi uniform triangulation of
be a regular, quasi uniform triangulation of  into nsimplexes of diameter less than h.
into nsimplexes of diameter less than h.
We denote by  the standard piecewise linear finite element espace, we consider the discrete variational inequality:
the standard piecewise linear finite element espace, we consider the discrete variational inequality:
 (18)
(18)
Thanks to ([6]), QVI (18) has a unique solution.
4.1. The Discrete Maximum Principle [7]
We assume that the matrix A with generic coefficient is a M-matrix.
 (19)
(19)
As for the continuous problem, it is easy to see that , the solution of (18), is also solution to the following (QVI):
, the solution of (18), is also solution to the following (QVI):
 (20)
(20)
Theorem 2 [1]: Let  and
and  (the discrete solution). Then, there exists a constant independent of
(the discrete solution). Then, there exists a constant independent of  the both and h such that
the both and h such that
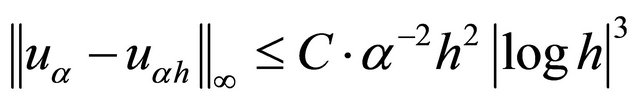
Theorem 3 [1]: Under conditions of theorem 1 and 2, we have
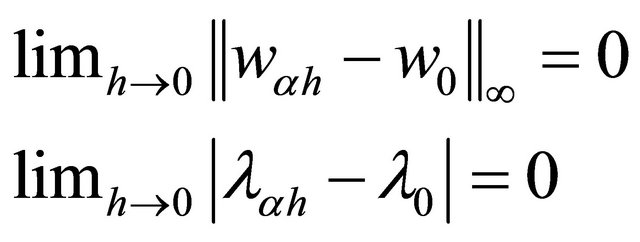
Note that  and
and  is the finite element approximation of
is the finite element approximation of 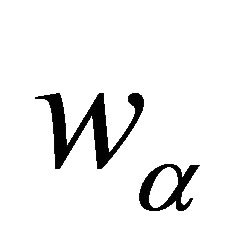 and
and 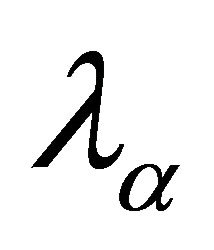 respectively.
respectively.
4.2. The Discrete Schwarz Sequences
Let  be the space of continuous piecewise linear function on
be the space of continuous piecewise linear function on  which vanish on
which vanish on .
.
For , we define
, we define

where  denotes the interpolation operator on
denotes the interpolation operator on .
.
For . Let
. Let 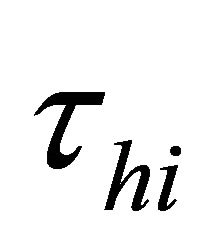 be a standard regular finite element triangulation in
be a standard regular finite element triangulation in ,
,  being the mesh size.
being the mesh size.
We suppose that the two triangulations are mutually independent on  a triangle belonging to one triangulation does not necessarily belong to the other.
a triangle belonging to one triangulation does not necessarily belong to the other.
Choosing , such that
, such that  is a solution of the following inequation:
is a solution of the following inequation:
 (21)
(21)
We define the alternating Schwarz sequences 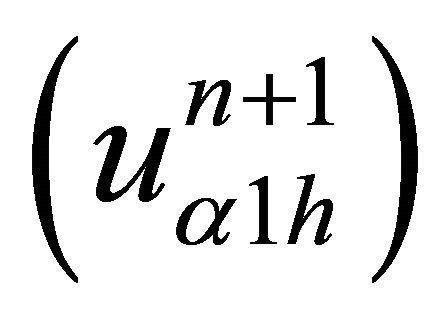 on
on  such that:
such that:
 (22)
(22)
And 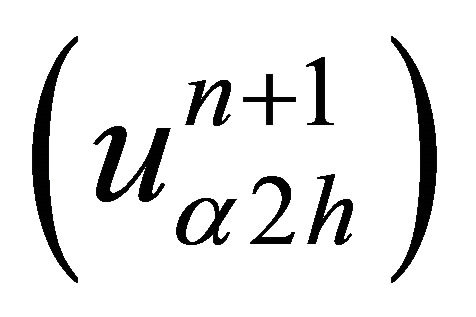 on
on  such that:
such that:
 (23)
(23)
Notation: We will adapt the following notations:
 (24)
(24)
5.  -Error Analysis
-Error Analysis
Lemma 2 [8]:  is M-matrix such that
is M-matrix such that

then exists constants 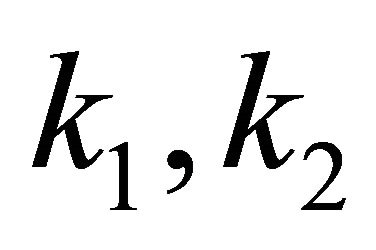 such that
such that
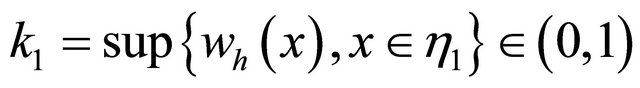
and 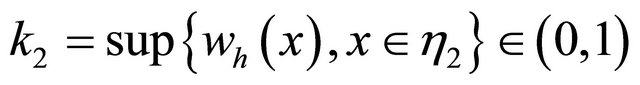
 (25)
(25)
Theorem 4 [8]:  produced by Schwarz alternating method converges geometrically to
produced by Schwarz alternating method converges geometrically to  the solution of obstacle problem, more precisely, there exist
the solution of obstacle problem, more precisely, there exist  which depend only respectively of
which depend only respectively of  and
and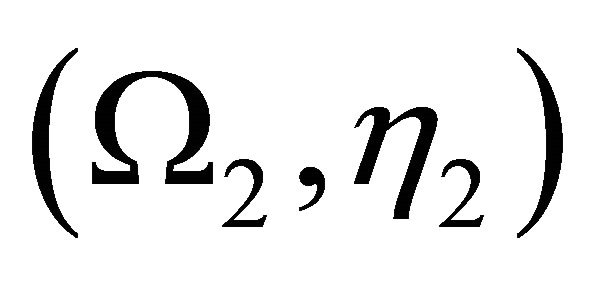 , such that
, such that
 (26)
(26)
Theorem 5: Let  Then, there exist two constants C and
Then, there exist two constants C and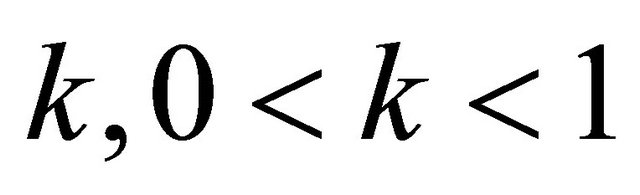 , independent of both h and n such that:
, independent of both h and n such that:
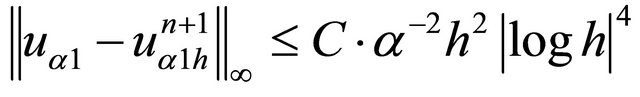
and
 .
.
Proof:


We obtain
 .
.
The case i = 2 is similar.
Theorem 6: Let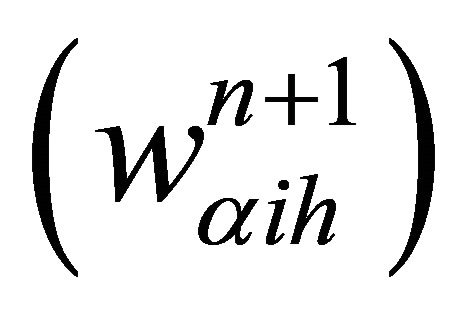 and
and , the discretes alternating Schwarz sequences, we have:
, the discretes alternating Schwarz sequences, we have:

Proof:
1)

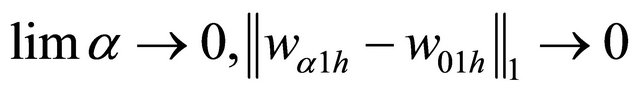
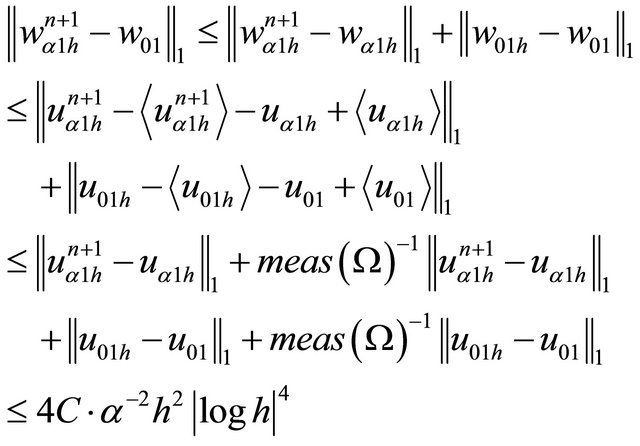
For:  and
and .
.
The case i = 2 is similar.
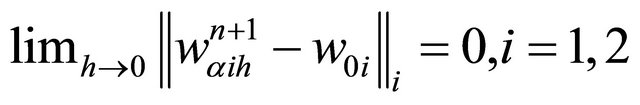 .
.
2)

Hence : for
: for 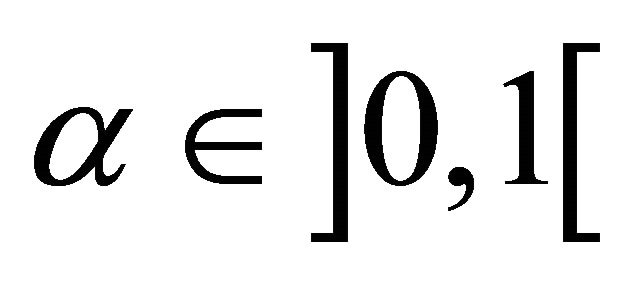
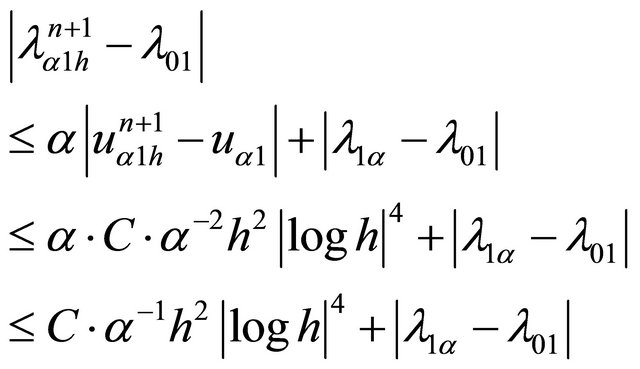
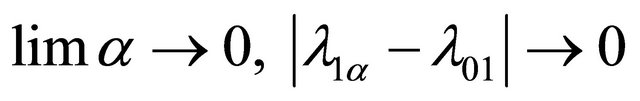 , For:
, For:  and
and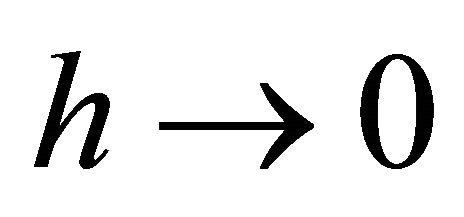 .
.
The case i = 2 is similar.
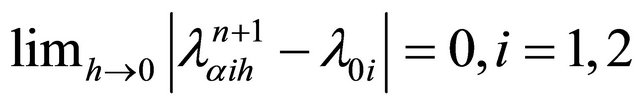 .
.