Common Fixed Point Theorems of Multi-Valued Maps in Ultra Metric Space ()
1. Introduction
Roovij in [1] introduced the concept of ultra metric space. Later, C. Petalas, F. Vidalis [2] and Ljiljana Gajic [3] studied fixed point theorems of contractive type maps on a spherically complete ultra metric spaces which are generalizations of the Banach fixed point theorems. In [4] K. P. R. Rao, G. N. V. Kishore and T. Ranga Rao obtained two coincidence point theorems for three or four self maps in ultra metric space.
J. Kubiaczyk and A. N. Mostafa [5] extend the fixed point theorems from the single-valued maps to the setvalued contractive maps. Then Gajic [6] gave some generalizations of the result of [3]. Again, Rao [7] proved some common fixed point theorems for a pair of maps of Jungck type on a spherically complete ultra metric space.
In this article, we are going to establish some results on coincidence and common fixed point for two pair of multi-valued and single-valued maps in ultra metric spaces.
2. Basic Concept
First we introducing a notation.
Let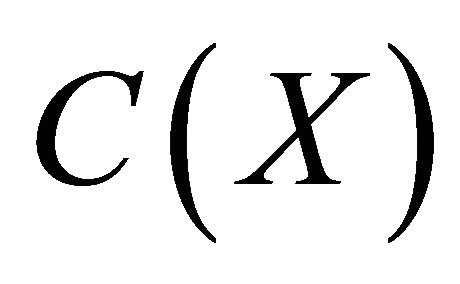 
denote the class of all non empty compact subsets of
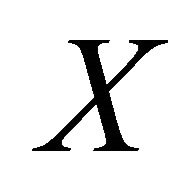. For
, the Hausdorff metric is defined as
where
.
The following definitions will be used later.
Definition 2.1 ([1]) Let
be a metric space. If the metric 
satisfies strong triangle inequality
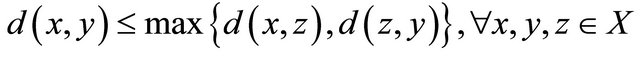
Then 
is called an ultra metric on 
and 
is called an ultra metric space.
Example. Let
,
, then
is a ultra metric space.
Definition 2.2 ([1]) An ultra metric space is said to be spherically complete if every shrinking collection of balls in 
has a non empty intersection.
Definition 2.3 An element
is said to be a coincidence point of 
and 
if
. We denote
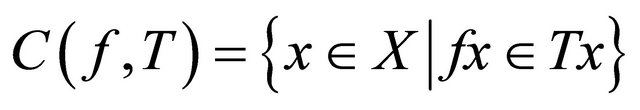
the set of coincidence points of 
and
.
Definition 2.4 ([7]) Let 
be an ultra metric space, 
and
. 
and 
are said to be coincidentally commuting at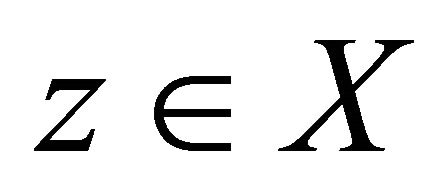 
if 
implies
.
Definition 2.5 ([8]) An element 
is a common fixed point of 
and 
if 
.
3. Main Results
The following results are the main result of this paper.
Theorem 3.1 Let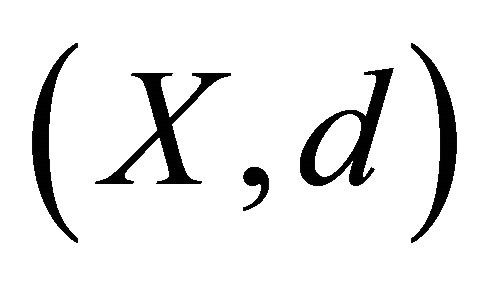 
be an ultra metric space. Let 
be a pair of multi-valued maps and 
a pair of single-valued maps satisfying
(a) 
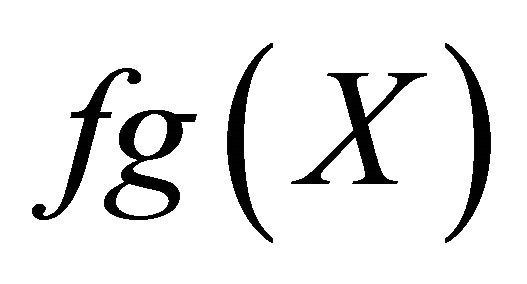is spherically complete;
(b)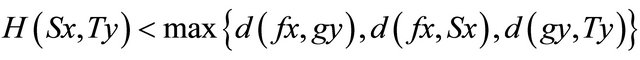 
for all
, with
;
(c) 
;
(d)
.
Then there exist point 
and 
in
, such that
.
Proof. Let
denote the closed sphere with centered
and radius
.
Let
be the collection of all the spheres for all
.
Then the relation
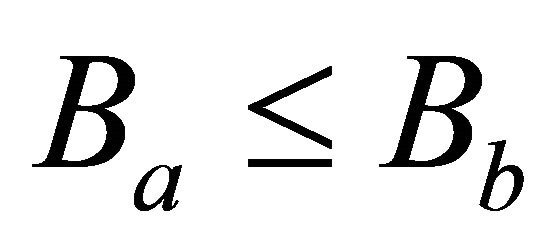if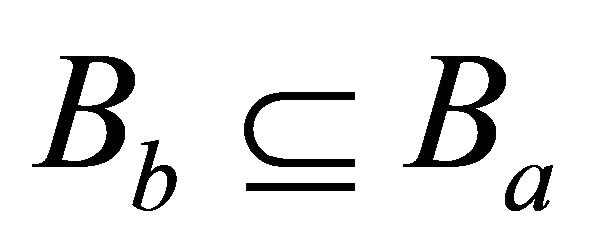 
is a partial order on
.
Consider a totally ordered sub family 
of
.
Since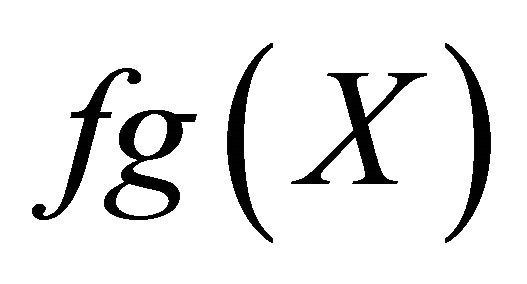 
is spherically complete, we have
Let 
where 
and
.
Then
. Hence
(1)
If 
then
. Assume that
.
Let
, then
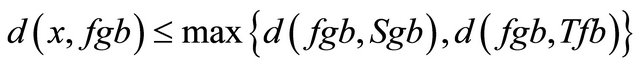
Since
is nonempty compact set, then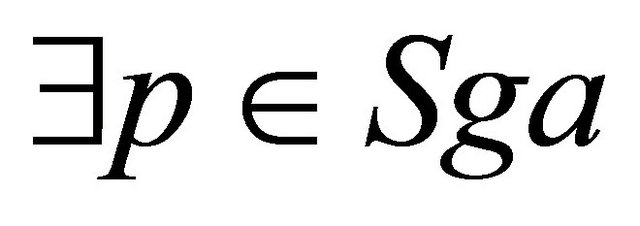
such that
;
is a nonempty compact set, then 
such that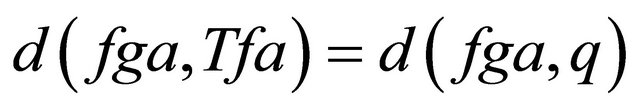
.
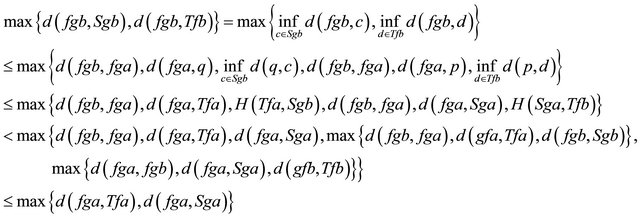
from (a) (b) and Equation (1)
Now
So
, we have just proved that 
for every
. Thus 
is an upper bound in 
for the family 
and hence by Zorn’s Lemma, there is a maximal element in
, say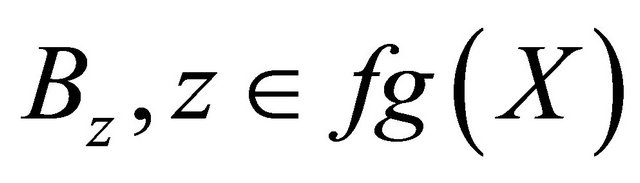
. There exists 
such that
.
Suppose
.
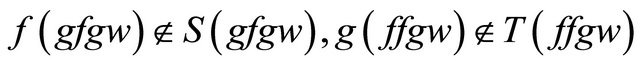Since 
are nonempty compact sets, then 
such that
(2)
(3)
From (b), (c) and Equation (2), we have
(4)
(5)
From (b), (c) and Equations (2)-(5)
(6)
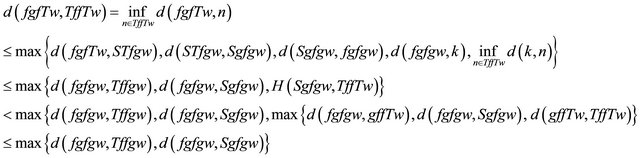(7)
From Equation (4) and Equation (6) we have
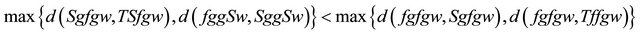(8)
From Equation (5) and Equation (7) we have
(9)
If
Then from Equation (8),
. Hence
. It is a contradiction to the maximality of 
in
, since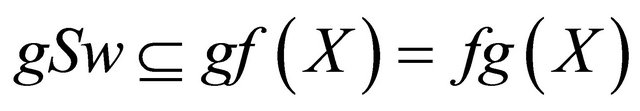 
If
Then from Equation (9),
. Hence
.It is a contradiction to the maximality of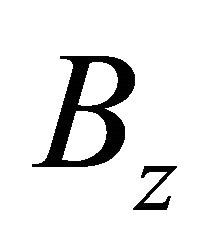 
in
, since
.
So
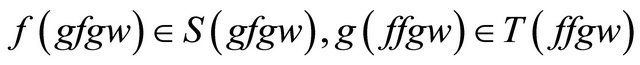(10)
In addition,
.
Using (b), (c) and Equation (10), we obtain
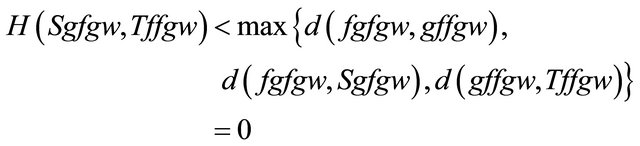
Hence
.
Then the proof is completed.
Theorem 3.2 Let 
be an ultra metric space. Let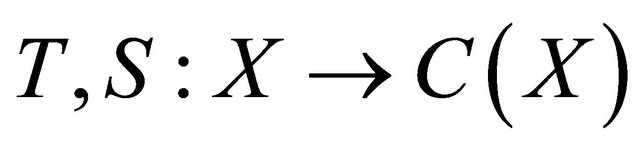 
be a pair of multi-valued maps and 
be a single-valued maps satisfying
(a) 
is spherically complete;
(b)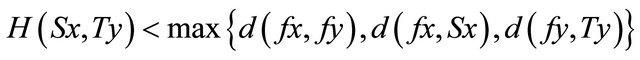 
for all
,with
;
(c)
;
(d)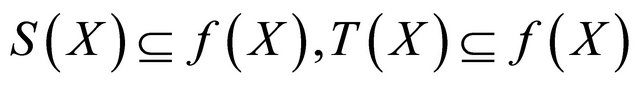
.
Then 
and 
have a coincidence point in
.
Moreover, if 
and
, 
and 
are coincidentally commuting at 
and
, then 
and 
have a common fixed point in
.
Proof. If 
in Theorem 2.1, we obtain that there exist points 
and 
in 
such that
.
As
, 
and 
ipipare coincidentally commuting at 
and
.
Write
, then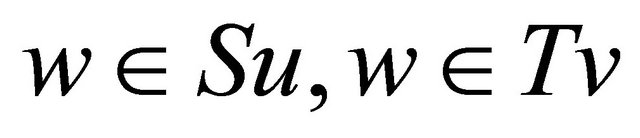
.
Then we have
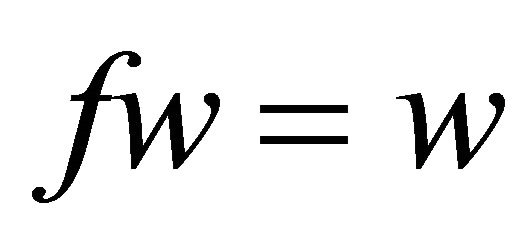
and
.
Now, since also
, 
and 
are coincidentally commuting at 
and
, so we obtain
.
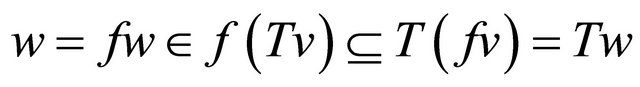Thus, we have proved that
, that is, 
is a common fixed point of 
and
.
Corollary 3.3 Let 
be a spherically complete ultra metric space. Let 
be a pair of multi-valued maps satisfying
(a) 
for all
,with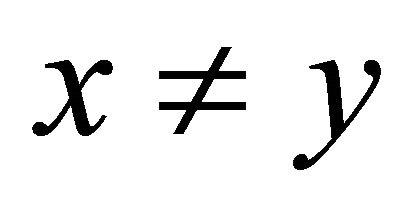
;
(b)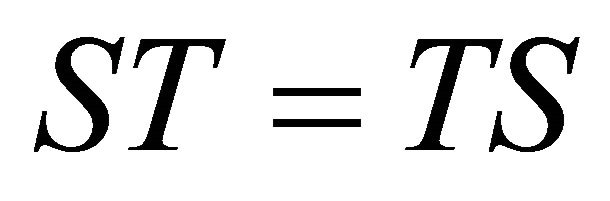
.
Then, there exists a point
in
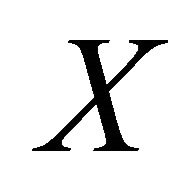such that 
and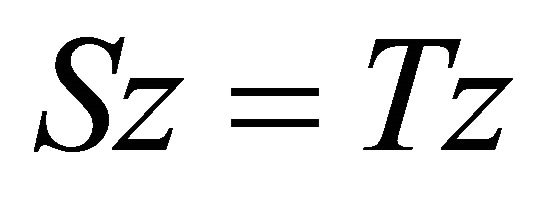
.
Remark 1 If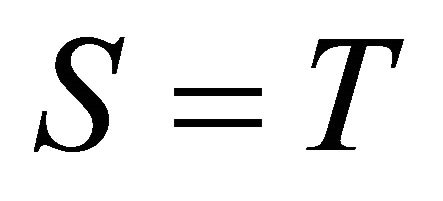 
in Corollary 3.3, then we obtain the Theorem of Ljiljana Gajic [6].
Remark 2 If in Theorem 3.1, 
, we obtain Theorem 9 of K. P. R. Rao at [7].
Remark 3 If
and
in Theorem 3.1 are single-valued maps, then: 1) we obtain the results of K. P. R. Rao [4]; 2)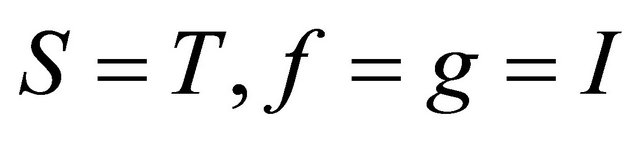
, we obtain the result of Ljiljana Gajic [3]; 3)
, then, we obtain Theorem 4 of K. P. R. Rao at [7].
4. Conclusion
In this paper, we get coincidence point theorems and common fixed point theorems for two pair of multi-valued and single-valued maps satisfying different contractive conditions on spherically complete ultra metric space, which is generalized results of [3-7].
5. Acknowledgements
Foundation item: Science and Technology Foundation of Educational Committee of Tianjin (11026177).
NOTES