Applied Mathematics
Vol.06 No.10(2015), Article ID:59963,17 pages
10.4236/am.2015.610159
Random Attractors for Stochastic Reaction-Diffusion Equations with Distribution Derivatives on Unbounded Domains
Eshag Mohamed Ahmed, Ali Dafallah Abdelmajid, Ling Xu, Qiaozhen Ma*
College of Mathematics and Statistics, Northwest Normal University, Lanzhou, China
Email: ahmedesag@gmail.com, majid_dafallah@yahoo.com, 13893414055@163.com, *maqzh@nwnu.edu.cn
Copyright © 2015 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 10 August 2015; accepted 22 September 2015; published 25 September 2015
ABSTRACT
In this paper, we prove the existence of random attractors for a stochastic reaction-diffusion equation with distribution derivatives on unbounded domains. The nonlinearity is dissipative for large values of the state and the stochastic nature of the equation appears spatially distributed temporal white noise. The stochastic reaction-diffusion equation is recast as a continuous random dynamical system and asymptotic compactness for this demonstrated by using uniform estimates far-field values of solutions. The results are new and appear to be optimal.
Keywords:
Stochastic Reaction-Diffusion Equation, Random Attractors, Distribution Derivatives, Asymptotic Compactness, Unbounded Domain

1. Introduction
The understanding of the asymptotic behavior of dynamical system is one of the most important problems of modern mathematical physics; one way to attack the problem for dissipative deterministic dynamical systems is to consider its global attractors. This is an invariant set that attracts all the trajectories of the system. Its geometry can be very complicated and reflects the complexity of the long-time dynamical of the systems. In this paper we investigate the asymptotic behavior of solutions to the following stochastic reaction-diffusion equations with distribution derivatives and additive noise defined in the space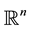 :
:
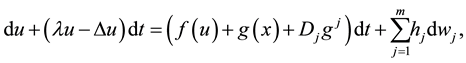 (1.1)
(1.1)
with initial data
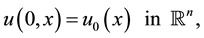 (1.2)
(1.2)
where 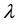 is a positive constant;
is a positive constant; 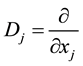 is distribution derivatives;
is distribution derivatives;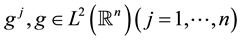 ; f is a
; f is a
nonlinear function satisfying certain dissipative conditions; hj is given functions defined on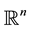 ; and
; and 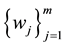 is independent two sided real-valued wiener processes on probability space which will be specified later.
is independent two sided real-valued wiener processes on probability space which will be specified later.
Stochastic differential equations of this type arise from many physical systems when random spatio-temporal forcing is taken into account. In order to capture the essential dynamics of random systems with wide fluctuations, the concept of pullback random attractors was introduced in [1] , being an extension to stochastic systems of the theory of attractors for deterministic equations found in [2] - [5] , for instance. The existence of such random attractors has been studied for stochastic PDE on bounded domains; see, e.g. [6] [7] , and for stochastic PDE on unbounded domains, see, e.g. [8] [9] , and the references therein. In the present paper, we prove the existence of such a random attractor for stochastic reaction-diffusion Equation (1.1) defined in 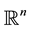 which is not founded.
which is not founded.
Notice that the unboundedness of domain introduces a major difficulty for proving the existence of an attractor because Sobolev embedding theorem is no longer compact and so the asymptotic compactness of solutions cannot be obtained by the standard method. In the case of deterministic equations, this difficulty can be overcome by the energy equation approach, introduced by Ball in [10] and then employed by several authors to prove the asymptotic compactness of deterministic equations in unbounded domains. This idea was developed in [5] to prove asymptotic compactness for the deterministic version of (1.1) on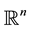 . In this paper, we provide uniform estimates on the far-field values of solutions to circumvent the difficulty caused by the unboundedness of the domains. The main contribution of this paper is to extend the method of using tail estimates of the case stochastic dissipative PDEs and prove the existence of random attractor for the stochastic reaction-diffusion equation with distribution derivatives on the unbounded domain
. In this paper, we provide uniform estimates on the far-field values of solutions to circumvent the difficulty caused by the unboundedness of the domains. The main contribution of this paper is to extend the method of using tail estimates of the case stochastic dissipative PDEs and prove the existence of random attractor for the stochastic reaction-diffusion equation with distribution derivatives on the unbounded domain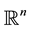 .
.
The paper is organized as follows. In Section 2, we recall some preliminaries and abstract results on the existence of a pullback random attractor for random dynamical systems. In Section 3, we transform (1.1) into a continuous random dynamical system. Section 4 is devoted to obtain the uniform estimates of solution as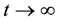 . These estimates are necessary for proving the existence of bounded absorbing sets and the asymptotic compactness of the equation. In Section 5, we first establish the asymptotic compactness of the solution operator by giving uniform estimates on the tails of solutions, and then prove the estimates of a random attractor.
. These estimates are necessary for proving the existence of bounded absorbing sets and the asymptotic compactness of the equation. In Section 5, we first establish the asymptotic compactness of the solution operator by giving uniform estimates on the tails of solutions, and then prove the estimates of a random attractor.
We denote by  and
and  the norm and the inner product in
the norm and the inner product in 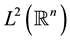 and use
and use 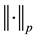 to denote the norm in
to denote the norm in


2. Preliminaries and Abstract Results
As mentioned in the introduction, our main purpose is to prove the existence of a random attractor. For that matter, first, we will recapitulate basic concepts related to random attractors for stochastic dynamical systems. The reader is referred to [6] [11] -[13] for more details. Let 

Definition 2.1. 









Definition 2.2. A continuous random dynamical system (RDS) on X over a metric dynamical system 
which is








Definition 2.3. A random bounded set 


where
Definition 2.4. Let D be a collection of random subsets of X and

called a random absorbing set for 



Definition 2.5. Let D be a collection of random subsets of X. Then 
ly compact in X if for P-a.e


and 

Definition 2.6. Let D be a collection of random sunsets of X. Then a random set 






(3) 

where d is the Hausdorff semi-metric given by 







Definition 2.7. Let D be an inclusion-closed collection of random subsets of X and 






In this paper, we will take D as the collection of all tempered random subsets of 

3. The Reaction-Diffusion Equation on Rn with Distribution Derivatives and Additive Noise

with initial condition

where 



are distribution derivative, 
space which will be specified below, and 



for 





In the sequel, we consider the probability space 





Define the time shift by
Then 
We now associate a continuous random dynamical system with the stochastic reaction-diffusion equation over


The solution of (3.6) is given by
Note that the random variable 



where 

Then it follows form (3.7), (3.8) that, for P-a.e.

Putting 
The existence and uniqueness of solutions to the stochastic partial differential Equation (3.1) with initial condition (3.2) which can be obtained by standard Fatou-Galerkin methods. To show that problem (3.1), (3.2) generates a random system, we let 


By a Galerkin method, one can show that if f satisfies (3.3)-(3.5), then in the case of a bounded domain with Dirichlet boundary conditions, for P-a.e.

with 





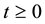

Then the process u is the solution of problem (3.1), (3.2), we now define a mapping 

Then 



4. Uniform Estimates of Solutions
In this section, we drive uniform estimates on the solutions of (3.1), (3.2) defined on 


We always assume that D is the collection of all tempered subsets of 


Lemma 4.1. Assume that gj, 





there is 
Proof. We first derive uniform estimates on 




For the nonlinear term, by (3.3)-(3.5) we obtain

on the other hand, the next two terms on the right-hand side of (4.1) are bounded by

the last term on the right-hand side of (4.1) is bounded by

where 

Then it follows from (4.1)-(4.4) that

Note that 


By (3.9), we find that for P-a.e,

it follows from (4.5), (4.6) that, all

which implies that for all

Let


By replacing 


Note that
So from (4.11) we get that, for all

By assumption 

therefore, if

which along with (4.12) shows that, for all

Given
Then


Which completes the Proof. ,
We next drive uniform estimates for u in 

Lemma 4.2. Assume that 


Then for every 







where C is a positive deterministic constant independent of 

Proof. First, replacing t by 


Multiply the above by 
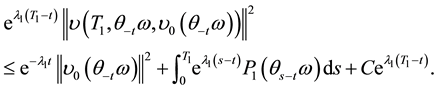
By (4.7), the second term on the right-hand side of (4.16) satisfies

From (4.16), (4.17) it follows that

By (4.8) we find that, for

Dropping the first term on the left-hand side of (4.19) and replacing 


By (4.7), the second term on the right-hand side of (4.20) satisfies, for all

Then, using (4.20) and (4.21), it follows from (4.20) that
This completes the proof. ,
Lemma 4.3. Assume that gj, 


Then for P-a.e





where C is a positive deterministic constant and 
Proof. First replacing t by t + 1 and then replacing 

Note that 


Since 


which along with (4.23) shows that, for all

Then from (4.10) using the same steps of last process applying on (4.15), we get that

The above uniform estimates is a special case lemma 4.2, then the lemma follows from (4.24)-(4.25). ,.
Lemma 4.4. Assume that gj, 


Then for P-a.e



where C is a positive deterministic constant and 
Proof. Let 



By (3.9) we obtain

Now integration (4.26) with respect to s over (t, t + 1), by lemma 4.3 and inequality (4.27), we have

Then the lemma follows from (4.28). ,
Lemma 4.5. Assume that gj, 





where C is a positive deterministic constant and 
Proof. Taking the inner product of (3.10) with 


We estimates the first term in the right-hand side of (4.29) by (3.3), (3.4) we have

On the other hand, the second term on the right-hand side of (4.29) is bounded by

The last term on the right-hand side of (4.29) is bounded by

By (4.29)-(4.32) we get that

Let

Since 



which along with (3.9) shows that

By (4.33), (4.34) we have

Let 


Now integrating the above equation with respect to s over (t, t + 1), we find that
Replacing 


By lemma 4.3 and 4.4, it follows from (4.37) and (4.35) that, for all

Then by 4.38 and 3.9, we have, for all
which completes the proof. ,
Lemma 4.6. Assume that gj, 


Then for every 





Proof. Choose a smooth function 



Then there exists a constant C such that 





We now estimate the terms in (4.39) as follows, first we have

Note that the second term on the right-hand side of (4.40) is bounded by

By (4.40), (4.41), we find that

From (4.39) the first term on the right-hand side, we have

By (3.3), the first term on the right-hand side of (4.43) is bounded by

By (3.4), the second term on the right-hand side of (4.43) is bounded by

Then it follows from (4.43)-(4.45) we have that

For the second term on the right-hand side of (4.39) we have

For the last term on the right-hand side of (4.39), we have that

Finally, by (4.39), (4.42) and (4.47) (4.48), we have that

Note that (4.49) implies that

By lemma 4.1 and 4.5, there is 


Now integrating (4.50) over 

Replacing 



In what follows, we estimate the terms in (4.53). First replacing t by 



where we have used (4.7). By (4.54), we find that, given


By lemma 4.2, there is 
And hence, there is 



First replacing t by s and then replacing 

This implies that there exist 




Then the second term on the right-hand side of (4.53), there exist 




Note that



For the five term on the right-hand side of (4.53), we have

Note that 


that for all 


where 


Let 



which shows that for all 
This completes the proof. ,
Lemma 4.7. Assume that gj, 


Then for every 



Proof. Let 



then by (4.62) and lemma 4.6, we get that, for all 
which completes the proof. ,
5. Random Attractors
In this section, we prove the existence of a D-random attractor for the random dynamical system 



Lemma 5.1. Assume that gj, 
pullback asymptotically compact in

has a convergent subsequence in 


Proof. Let 



Hence, there is 

Next, we prove the weak convergence of (5.1) is actually strong convergence. Given




Since





On the other hand, by lemma 4.1 and 4.5, there 


Let 

Denote by 
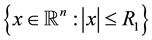

which shows that for the given



Note that


let 


which shows that
as wanted. ,
Now we are in a position to present our main result: the existence of a D-random attractor for 
Theorem 5.2. Assume that



Proof. Notice that 

asymptotically compact in 

Foundation Term
This work was supported by the NSFC (11101334).
Cite this paper
Eshag MohamedAhmed,Ali DafallahAbdelmajid,LingXu,QiaozhenMa, (2015) Random Attractors for Stochastic Reaction-Diffusion Equations with Distribution Derivatives on Unbounded Domains. Applied Mathematics,06,1790-1807. doi: 10.4236/am.2015.610159
References
- 1. Flandoli, F. and Schmalfuβ, B. (1996) Random Attractors for the 3D Stochastic Navier-Stokes Equation with Multiplicative Noise. Stochastics and Stochastic Reports, 59, 21-45.
http://dx.doi.org/10.1080/17442509608834083 - 2. Antoci, F. and Prizzi, M. (2001) Reaction-Diffusion Equations on Unbounded Thin Domains. Topological Methods in Nonlinear Analysis, 18, 283-302.
- 3. Hale, J.K. (1988) Asymptotic Behavior of Dissipative Systems. American Mathematical Society, Providence.
- 4. Robinson, J.C. (2001) Infinite-Dimensional Dynamical Systems. Cambridge University Press, Cambridge.
http://dx.doi.org/10.1007/978-94-010-0732-0 - 5. Wang, B. (1999) Attractors for Reaction-Diffusion Equations in Unbounded Domains. Physica D, 128, 41-52.
http://dx.doi.org/10.1016/S0167-2789(98)00304-2 - 6. Crauel, H., Debussche, A. and Flandoli, F. (1997) Random Attractors. Journal of Dynamics and Differential Equations, 9, 307-341.
http://dx.doi.org/10.1007/BF02219225 - 7. Caraballo, T., Langa, J.A. and Robinson, J.C. (2001) A Stochastic Pitchfork Bifurcation in a Reaction-Diffusion Equation. Proceedings of the Royal Society A, 457, 2041-2061.
http://dx.doi.org/10.1098/rspa.2001.0819 - 8. Bates, P.W., Lu, K.N. and Wang, B.X. (2009) Random Attractors for Stochastic Reaction-Diffusion Equations on Unbounded Domains. Journal of Differential Equations, 246, 845-869.
http://dx.doi.org/10.1016/j.jde.2008.05.017 - 9. Rosa, R. (1998) The Global Attractor for the 2D Navier-Stokes Flow on Some Unbounded Domains. Nonlinear Analysis, 32, 71-85.
http://dx.doi.org/10.1016/S0362-546X(97)00453-7 - 10. Ball, J.M. (2004) Global Attractors for Damped Semilinear Wave Equations. Discrete and Continuous Dynamical Systems, 10, 31-52.
http://dx.doi.org/10.3934/dcds.2004.10.31 - 11. Arnold, L. (1998) Random Dynamical Systems. Springer-Verlag, Berlin.
http://dx.doi.org/10.1007/978-3-662-12878-7 - 12. Crauel, H., Debussche, A. and Flandoli, F. (1997) Random Attractors. Journal of Dynamics and Differential Equations 9, 307-341.
http://dx.doi.org/10.1007/BF02219225 - 13. Crauel, H. and Flandoli, F. (1994) Attractors for Random Dynamical Systems. Probability Theory and Related Fields, 100, 365-393.
http://dx.doi.org/10.1007/BF01193705
NOTES
*Corresponding author.
































































