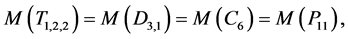Applied Mathematics
Vol.07 No.09(2016), Article ID:66814,7 pages
10.4236/am.2016.79082
The Matching Equivalence Graphs with the Maximum Matching Root Less than or Equal to 2
Haicheng Ma, Yinkui Li
Department of Mathematics, Qinghai University for Nationalities, Xining, China

Copyright © 2016 by authors and Scientific Research Publishing Inc.
This work is licensed under the Creative Commons Attribution International License (CC BY).
http://creativecommons.org/licenses/by/4.0/



Received 23 March 2016; accepted 24 May 2016; published 27 May 2016
ABSTRACT
In the paper, we give a necessary and sufficient condition of matching equivalence of two graphs with the maximum matching root less than or equal to 2.
Keywords:
Matching Polynomial, Matching-Equivalent, Matching Unique

1. Introduction
Let G be a finite simple graph with vertex set 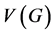 and edge set
and edge set . A spanning subgraph H is called a matching of G, if every connected component of H is isolated edge or isolated vertex. k-matching of G is a matching with k edges. A matching polynomial of G is defined as
. A spanning subgraph H is called a matching of G, if every connected component of H is isolated edge or isolated vertex. k-matching of G is a matching with k edges. A matching polynomial of G is defined as
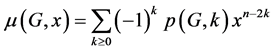
where 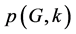 is the number of k-matchings of G.
is the number of k-matchings of G.
Two graphs G and H are called matching-equivalent if , and denoted by
, and denoted by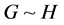 . A graph G is called matching unique if
. A graph G is called matching unique if 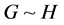 implies that
implies that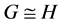 . The union of two graphs G and H, denoted by
. The union of two graphs G and H, denoted by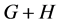 , is the graph with vertex set
, is the graph with vertex set 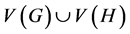 and edge set
and edge set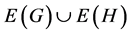 .
. 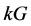 denotes the union of k graphs G.
denotes the union of k graphs G.
More than 30 years ago E. J. Farrell in [1] introduced the concept of matching polynomials. Latterly, Godsil and Gutman in [2] gave another definition. Here we use the definition given by Godsil. Form then on, the research on the properties of matching polynomials has largely been done (see [3] - [13] ). But the research on matching-equivalent of graphs is few. In this paper, we give a necessary and sufficient condition of matching equivalence of two graphs with the maximum matching root less than or equal to 2.
Throughout the paper, by 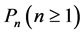 and
and , respectively, denote the path and the cycle with n vertices.
, respectively, denote the path and the cycle with n vertices. 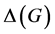 denotes the maximum degree of graph G. By
denotes the maximum degree of graph G. By 













2. Graphs with the Maximum Matching Root Less than or Equal to 2
Let G be a graph with order n. Since the roots of 






Lemma 2.1. [7] Let G be a graph with k components
Lemma 2.2. [7] Let G be a forest. Then
Lemma 2.3. [7] Let G be a connected graph and
1) 


2) 


Definition 2.1. Let G be a connected graph with a vertex u. The path-tree 
Clearly, if G is a tree, then the path tree
Lemma 2.4. [7] Let u be a vertex in the graph G and 
and 
Lemma 2.5. Let G is a connected graph and


Proof. By Lemmas 2.2 and 2.4, we have





□Lemma 2.6. [14] Let T be a tree. Then
1) 

2) 

Theorem 2.1. Let G be a connected graph. Then
Figure 1. The graphs 

1) 

2) 

Proof. (1) Since the path-tree of 



Necessity:
Case 1. If G is a tree.
Clearly,

Case 2. If G isn’t a tree.
By Lemma 2.5 and 2.6, the path-tree respect to an arbitrary vertex u of G is


Subcase 2.1. If






Subcase 2.2. If
Since G is connected and isn't a tree, then G is

(2) Since the path-tree of 



Necessity:
Case 1. If G is a tree.
Clearly,

Case 2. If G isn’t a tree.
By Lemma 2.5, the path-tree respect to an arbitrary vertex u of G is


Subcase 2.1. If
Let u is a 4 degree vertex of G. Since


Subcase 2.2. If 

It is clear that the number of 3 degree vertex of path-tree 

Subcase 2.3. If 




Subcase 2.4. If 

It is clear that 






By Theorem 2.1 and Lemma 2.1, we can easily obtain the following Theorem 2.2:
Theorem 2.2. Let G be a graph. Then
1) 

2) 



Figure 2. Four connected graphs with 

3. Sufficient and Necessary Condition for Matching Equivalence of Graphs
In this section, the sufficient and necessary condition for matching equivalence of graphs with the maximum matching root less than or equal to 2 is determined. Firstly, we give some lemmas as follows:
Lemma 3.1. [7] Let G be a connected graph and
where 
Lemma 3.2. 1)
2)
3)
4)
5)
6)
7)
8)
9)
10)
11)
12)
13)
Proof. (1) Let the vertices sequence of path 




(2) Let v be the 3 degree vertex and u be a such pendant vertex of 


(3)-(12) The results (3)-(12) can easily obtained by the following equalities.
(13) By Lemma 3.1, 


Now, by using mathematical induction to prove (13). Firstly, By (8) and
(13) holds for

Hence (13) holds for
Lemma 3.3. 1)
2)
3)
4)
5)
6)
Proof. Clearly, by Lemma 2.3, we obtain Lemma 3.3(1) immediately. And comparing with the maximum root of two sides of equalities in Lemma 3.2, other results in Lemma 3.3 is also obvious. □
Definition 3.1. Let G and 
where 


Note that some 










By Lemma 3.2, the following representations are also obvious.
Lemma 3.4. 1) 
3) 
5) 
7) 
9) 
11) 

Lemma 3.5. If
and the non-vanishing coefficient




Proof. Since


where 

Now by transposition terms from side to side of Equations (1) to (2) such that the coefficients of 


where


Compare with the maximum root and its multiplicity of graphs in two sides of (2), we shall get
Repeat this proceeding, we shall get 

Furthermore, assume that G be represented as a linear combination

and 

In fact, assume that


where 



it is a contradiction. Thus 
compare with the maximum root of 


Lemma 3.6. If



Proof. Since


By transposition terms and comparing with the multiplicity of root 2, we have 

Furthermore, we can obtain 


By Lemmas 3.5, 3.6 and Definition 3.1 we immediately get. □
Theorem 3.1. Let 
1) If

2) If


Acknowledgements
This work is supported by National Natural Science Foundation of China (11561056), National Natural Science Foundation of Qinghai Provence (2011-Z-911), and Scientific Research Fund of Qinghai University for Nationalities (2015G02).
Cite this paper
Haicheng Ma,Yinkui Li, (2016) The Matching Equivalence Graphs with the Maximum Matching Root Less than or Equal to 2. Applied Mathematics,07,920-926. doi: 10.4236/am.2016.79082
References
- 1. Farrell, E.J. (1979) An Introduction to Matching Polynomial. Journal of Combinatorial Theory, 27, 75-86.
http://dx.doi.org/10.1016/0095-8956(79)90070-4 - 2. Godsil, C.D. and Gutman, I. (1981) On the Theory of the Matching Polynomials. Journal of Graph Theory, 5, 79-87.
http://dx.doi.org/10.1002/jgt.3190050203 - 3. Beezer, R.A. and Farrell, E.J. (1995) The Matching Polynomials of a Regular Graph. Discrete Mathematics, 137, 7-8.
http://dx.doi.org/10.1016/0012-365X(93)E0125-N - 4. Farrell, E.J. and Whitehead Jr., E.G. (1992) Connections between the Matching and Chromatic Polynomials. International Journal of Mathematics and Mathematical Sciences, 15, 757-766.
http://dx.doi.org/10.1155/S016117129200098X - 5. Farrell, E.J. and Guo, J.M. (1993) On the Characterizing Properties of Matching Polynomials. Vishwa International Journal of Graph Theory, 2, 55-62.
- 6. Farrell, E.J., Guo, J.M. and Constantine, G.M. (1991) On Matching Coefficents. Discrete Mathematics, 89, 203-210.
http://dx.doi.org/10.1016/0012-365X(91)90369-D - 7. Godsil, C.D. (1993) Algebraic Combinatorics. Chapman and Hall, New York.
- 8. Godsil, C.D. (1981) Hermite Polynomiala and a Duality Relation for Matching Polynomials. Combinnatorica, 1, 257- 262.
http://dx.doi.org/10.1007/BF02579331 - 9. Godsil, C.D. and Gutman, I. (1981) Some Remarks on the Matching Polynomial and Its Zeros. Croatica Chemica Acta, 54, 53-59.
- 10. Heilmann, O.J. and Lieb, E.H. (1972) Theory of Monomer-Dimer Systems. Communications in Mathematical Physics, 25, 190-232.
http://dx.doi.org/10.1007/BF01877590 - 11. Ma, H.C. and Xia, H. (2001) The Graph with Matching Polynomials Maximum Roots of no Excess 2. Journal of Jilin Institute of Chemical Technology, 18, 67-68. (In Chinese)
- 12. Ma, H.C. (2000) On the Matching Equivalent Classes of Two Kind of Graphs. Journal of Mathematical Study, 33, 218- 222. (In Chinese)
- 13. Ma, H.C. (2002) The Matching Equivalent Classes of Graphs of I Shape. Journal of Mathematical Study, 35, 65-71. (In Chinese)
- 14. Cvetkovic, D.M., Doob, M. and Sachs, H. (1995) Spectra of Graphs. 3rd Edition, Johann Abrosius Barth Verlag.