The Pancharatnam Phase of a Three-Level Atom Coupled to Two Systems of N-Two Level Atoms ()
Received 7 February 2016; accepted 25 March 2016; published 30 March 2016

1. Introduction
The use of statistical mechanics is fundamental to the concepts of quantum optics: Light is described in terms of field operators for creation and annihilation of photons [1] - [7] . Features of quantum optics are mainly based on three different types of interaction, namely, field-field, atom-atom, atom-field interaction. Each one of these interactions represents a certain type of physical phenomena [8] - [13] . For example, Hichem Eleuch and Raouf Bennaceur studied the interaction between a three-level system in the lambda configuration with two resonant electromagnetic fields, through which the motion of a pair of solitons propagating through an absorbing three- level system in the lambda configuration was analyzed [14] .
In addition, based on Dicke’s superradiance, Eyob A. Sete et al. studied the collective spontaneous emission from an ensemble of N identical two-level atoms prepared by absorption of a single photon―a.k.a. single photon Dicke superradiance [15] .
In the present communication we are concerned with the type of atom-atom (spin-spin) interaction, the interaction between a three-level atom and two systems of N-two level atoms. The time evolution of dynamical systems has attracted considerable attention over the past several decades because of its various applications. An important aspect in this regard is the quantum phase associated with the evolution of these states in certain circumstances [16] .
In recent years much attention has paid to the quantum phases [17] such as the Pancharatnam phase [18] - [22] and the geometric phase [23] [24] . The concept of geometric phase naturally arises for polarized light in optics. Simple quantum gates using geometric phase have been demonstrated experimentally in the nuclear magnetic resonance setup [25] . In the fifties, Pancharatnam [26] came up with a rigorous prescription for the phase acquired in a completely general evolution of a system. Pancharatnam discovered that when the polarization state of a beam of light was taken around a closed circuit in the state space, namely the Poincar. Sphere, it acquires an extra phase which is equal to half the solid angle subtended by the circuit at the origin of the sphere [27] . In 1956, Pancharatnam [26] studied how the phase of polarized light changed after a cyclic evolution of its polarization [28] . In 1984 Berry addressed a quantum system undergoing a unitary and cyclic evolution under the action of a time-dependent Hamiltonian [29] . The process was supposed to be adiabatic, meaning that the time scale of the system’s evolution was much shorter than the time scale of the changing Hamiltonian [30] .
The Pancharatnam phase or most commonly Berry phase is a phase difference acquired over the course of a cycle when a system is subjected to cyclic adiabatic processes, which results from the geometrical properties of the parameter space of the Hamiltonian. The Pancharatnam phase is very important in the propagation of a light beam where its polarization state is changing periodically [18] . Hence, we study the Pancharatnam phase of a three-level atom coupled to two systems of N-two level atoms as an application.
This paper is organized as follows: in section 2, we will describe the Hamiltonian of the system of interest, and obtain the explicit analytical solution of the model describing the interaction between a three-level atom and two systems of N-two level atoms. The case discussed in this paper is considered to be the generalization of the atom-atom interaction, and most of the previous papers which handled this interaction are, for the most part, considered to be a special case of our case. In section 3, different cases are studied to demonstrate the effects due to both the quantum numbers m1, m2 and the coupling parameters between spins λ1, λ2 on the atomic inversion  of the model. By analytical calculations in section 4, we examine the influence of the quantum numbers m1, m2 and the coupling parameters between spins λ1, λ2 on the Pancharatnam phase
of the model. By analytical calculations in section 4, we examine the influence of the quantum numbers m1, m2 and the coupling parameters between spins λ1, λ2 on the Pancharatnam phase 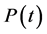 of the model. In section 5, we discuss the second-order correlation function where the examination of the second-order correlation function leads to better understanding for the nonclassical behavior of the system. Finally, section 6 presents the conclusions and an outlook.
of the model. In section 5, we discuss the second-order correlation function where the examination of the second-order correlation function leads to better understanding for the nonclassical behavior of the system. Finally, section 6 presents the conclusions and an outlook.
2. The Model
The Hamiltonian of our model describes the interaction between a three-level atom coupled to two systems of N-two level atoms. In this case the Hamiltonian of the whole system can be written in the form:
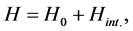 (1)
(1)
where,
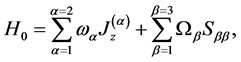 (2)
(2)
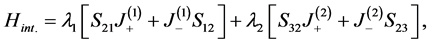 (3)
(3)
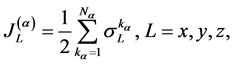 (4)
(4)
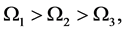 (5)
(5)
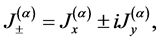 (6)
(6)
where 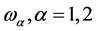 is the strength of the field (the two systems of N-two level atoms). The operators
is the strength of the field (the two systems of N-two level atoms). The operators 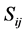 satisfy the commutation relation
satisfy the commutation relation
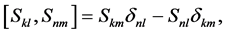 (7)
(7)
while  and
and 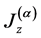 are the collective angular momentum operators for N-two level atoms, which satisfy the relations
are the collective angular momentum operators for N-two level atoms, which satisfy the relations
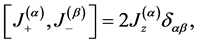 (8)
(8)
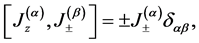 (9)
(9)
and
![]() (10)
(10)
with the operator ![]() is the usual Pauli matrices.
is the usual Pauli matrices.
We define
![]() (11)
(11)
![]() (12)
(12)
![]() (13)
(13)
![]() (14)
(14)
Let
![]() (15)
(15)
From Schrödinger equation
![]() (16)
(16)
we get from Equations (1), (15)
![]() (17)
(17)
![]() (18)
(18)
![]() (19)
(19)
where,
![]() (20)
(20)
![]() (21)
(21)
![]() (22)
(22)
![]() (23)
(23)
where ![]() is the coupling parameters between spins.
is the coupling parameters between spins.
Define
![]() (24)
(24)
by substituting from Equation (24) in Equations (17)-(19) we get the following equations:
![]() (25)
(25)
![]() (26)
(26)
![]() (27)
(27)
from Figure 1 we set
![]() (28)
(28)
So, we can write the Equations (25)-(27) as the following:
![]() (29)
(29)
![]() (30)
(30)
![]() (31)
(31)
We solve the Equations (29)-(31) analytically, we get:
![]() (32)
(32)
where,
![]() (33)
(33)
![]() (34)
(34)
![]()
Figure 1. Scheme of the interaction between a three-level atom coupled to two systems of N-two level atoms.
![]() (35)
(35)
similarly
![]() (36)
(36)
![]() (37)
(37)
and
![]() (38)
(38)
![]() (39)
(39)
So from Equation (24) we get ![]() and
and ![]()
As applications to the solution of our case, a three-level atom coupled to two systems of N-two level atoms, we calculate the atomic inversion, the Pancharatnam phase and the correlation functions.
3. The Atomic Inversion
The atomic population inversion![]() , can be considered as one of the simplest important quantities, it is defined as “the difference between the probabilities of finding the atom in their exited states and in its ground state”.
, can be considered as one of the simplest important quantities, it is defined as “the difference between the probabilities of finding the atom in their exited states and in its ground state”.
In Figures 2(a)-(c), we consider (Δ = 0, λ1 = λ2 = 1 and j1 = 30, j2 = 20). We investigate the effect of the
quantum numbers m1, m2 on the atomic inversion. In Figure 2(a), the initial state is![]() , the atomic
, the atomic
inversion (m1 = m2 = 1) has regular and periodic oscillations. It starts from its maximum value, ![]() , then it decreases until it reaches its minimum value,
, then it decreases until it reaches its minimum value,![]() . We observe, when the quantum numbers m1, m2 increase (m1 = m2 = 18), the phase of periodic oscillations gradually decreases, until it reaches zero (m1 = m2 = 20) (straight line), but the minimum value increases until it equalizes the maximum value (
. We observe, when the quantum numbers m1, m2 increase (m1 = m2 = 18), the phase of periodic oscillations gradually decreases, until it reaches zero (m1 = m2 = 20) (straight line), but the minimum value increases until it equalizes the maximum value (![]() )
)
(straight line). In Figure 2(b), the initial state is![]() , the atomic inversion has regular
, the atomic inversion has regular
and periodic oscillations. It starts from its maximum value, ![]() , then it decreases until it reaches its minimum value. We observe, when the quantum numbers m1, m2 increases, the number of periodic oscillations and the phase of periodic oscillations gradually decrease, but the minimum value increases remarkably. In Figure
, then it decreases until it reaches its minimum value. We observe, when the quantum numbers m1, m2 increases, the number of periodic oscillations and the phase of periodic oscillations gradually decrease, but the minimum value increases remarkably. In Figure
2(c), the initial state is![]() , the atomic inversion has regular and periodic oscillations.
, the atomic inversion has regular and periodic oscillations.
It starts from its maximum value, ![]() , then it decreases until it reaches its minimum value
, then it decreases until it reaches its minimum value![]() . The atomic inversion (m1 = m2 = 1) has small oscillations in the middle of the apexes of the regular and periodic oscillations. When the quantum numbers m1, m2 increase, the number of periodic oscillations decreases and the small oscillations disappear gradually. In Figure 3, we consider (Δ = 0, m1 = m2 = 1, j1 = 30, j2 = 20 and the initial state is
. The atomic inversion (m1 = m2 = 1) has small oscillations in the middle of the apexes of the regular and periodic oscillations. When the quantum numbers m1, m2 increase, the number of periodic oscillations decreases and the small oscillations disappear gradually. In Figure 3, we consider (Δ = 0, m1 = m2 = 1, j1 = 30, j2 = 20 and the initial state is![]() ). We investigate the effect of the coupling parameters between spins λ1, λ2 on the
). We investigate the effect of the coupling parameters between spins λ1, λ2 on the
![]()
Figure 3. Figure of the case in which Δ = 0, m1 = m2 = 1, j1 = 30, j2 = 20 and the initial state is![]() , where the dashed, bold solid, gray solid curves correspond, respectively, to λ1 = λ2 = 1, 0.5, 0.25.
, where the dashed, bold solid, gray solid curves correspond, respectively, to λ1 = λ2 = 1, 0.5, 0.25.
atomic inversion. The atomic inversion has regular and periodic oscillations. It starts from its maximum value, ![]() , then it decreases until it reaches its minimum value,
, then it decreases until it reaches its minimum value,![]() . We observe, there is constant interval at the maximum value. It increases remarkably when the coupling parameters between spins λ1, λ2 decrease and the number of periodic oscillations gradually decreases.
. We observe, there is constant interval at the maximum value. It increases remarkably when the coupling parameters between spins λ1, λ2 decrease and the number of periodic oscillations gradually decreases.
4. The Pancharatnam Phase
The total phase both dynamic and geometric phase parts for an arbitrary quantum evolution of a system from a state at t = 0 to a final state at time t. Without invoking the fact that any initial state vector ![]() and the final state vector
and the final state vector ![]() correspond to different rays, we use the Pancharatnam phase approach of defining the phase between them. The Pancharatnam phase
correspond to different rays, we use the Pancharatnam phase approach of defining the phase between them. The Pancharatnam phase ![]() between the vectors
between the vectors ![]() and
and ![]() is given by [31]
is given by [31]
![]() (40)
(40)
In Figure 4, we consider (Δ = 0, λ1 = λ2 = 1 and j1 = 30, j2 = 20 and the initial state is![]() ). We investigate the effect of the quantum numbers m1, m2 on the Pancharatnam phase. It has regular and periodic straight lines following the shape of the letter N. It starts from zero then decreases until it reaches its minimum value, P = −3, then it increases until it reaches its maximum value, P = 3. We note that there is a regular repeat in the behavior of the Pancharatnam phase. We observe, when the quantum numbers m1, m2 increase, each period of the Pancharatnam phase has widen on the time axe and the overall number of periods decreases. In Figure 5, we consider (Δ = 0, m1 = m2 = 1, j1 = 30, j2 = 20 and the initial state is
). We investigate the effect of the quantum numbers m1, m2 on the Pancharatnam phase. It has regular and periodic straight lines following the shape of the letter N. It starts from zero then decreases until it reaches its minimum value, P = −3, then it increases until it reaches its maximum value, P = 3. We note that there is a regular repeat in the behavior of the Pancharatnam phase. We observe, when the quantum numbers m1, m2 increase, each period of the Pancharatnam phase has widen on the time axe and the overall number of periods decreases. In Figure 5, we consider (Δ = 0, m1 = m2 = 1, j1 = 30, j2 = 20 and the initial state is![]() ). We investigate the effect of the coupling parameters between spins λ1, λ2 on the Pancharatnam phase. It has regular and periodic straight lines following the shape of the letter N. It starts from zero then decreases until it reaches its minimum value, P = −3, then it increases until it reaches its maximum value, P = 3. We note that there is a regular repeat in the behavior of the Pancharatnam phase. We observe, when the coupling parameters between spins λ1, λ2 decrease, each period of the Pancharatnam phase has widen on the time axe and the overall number of periods decreases remarkably. Finally, comparing the change of the Pancharatnam phase in Figure 4 and Figure 5, we observe that the effect of the quantum numbers m1, m2 is larger than the effect of the coupling parameters between spins λ1, λ2 in respect to the overall number of periods.
). We investigate the effect of the coupling parameters between spins λ1, λ2 on the Pancharatnam phase. It has regular and periodic straight lines following the shape of the letter N. It starts from zero then decreases until it reaches its minimum value, P = −3, then it increases until it reaches its maximum value, P = 3. We note that there is a regular repeat in the behavior of the Pancharatnam phase. We observe, when the coupling parameters between spins λ1, λ2 decrease, each period of the Pancharatnam phase has widen on the time axe and the overall number of periods decreases remarkably. Finally, comparing the change of the Pancharatnam phase in Figure 4 and Figure 5, we observe that the effect of the quantum numbers m1, m2 is larger than the effect of the coupling parameters between spins λ1, λ2 in respect to the overall number of periods.
The Correlation Function
In this section, we discuss the behavior of the second-order correlation function where the examination of the correlation function is usually used to discuss the correlated or uncorrelated behavior from which we can distinguish between classical and nonclassical behavior. The normalized second-order correlation function is defined by [13] [32]
![]() (41)
(41)
![]()
Figure 4. The evolution of the Pancharatnam phase as function of the scaled time t. Δ = 0, λ1 = λ2 = 1 and j1 = 30, j2 = 20 and the initial state is![]() , where the dashed, bold solid, gray solid curves correspond, respectively, to m1 = m2 = 1, 18, 20.
, where the dashed, bold solid, gray solid curves correspond, respectively, to m1 = m2 = 1, 18, 20.
![]()
Figure 5. Figure of the case in which Δ = 0, m1 = m2 = 1, j1 = 30, j2 = 20 and the initial state is![]() , where the dashed, bold solid, gray solid curves correspond, respectively, to λ1 = λ2 = 1, 0.5, 0.2.
, where the dashed, bold solid, gray solid curves correspond, respectively, to λ1 = λ2 = 1, 0.5, 0.2.
To discuss the behavior of the correlation function, we have to calculate the expectation value of the quantity ![]() and
and ![]() which can be obtained as:
which can be obtained as:
We know that
![]() (42)
(42)
![]() (43)
(43)
So
![]() (44)
(44)
![]() (45)
(45)
we get from Equations (15) (41)
![]() (46)
(46)
![]() (47)
(47)
where
![]() (48)
(48)
![]() (49)
(49)
In Figure 6, we consider (Δ = 0, λ1 = λ2 = 1, ![]() and the initial state is
and the initial state is![]() ). We investi-
). We investi-
gate the effect of the quantum numbers m1, m2 on the correlation functions ![]() and
and ![]() for the systems 1 and
for the systems 1 and
2 respectively. When![]() ,
, ![]() , at any time, so in this case the functions show uncorrelated behavior. When
, at any time, so in this case the functions show uncorrelated behavior. When![]() , the correlation functions
, the correlation functions![]() ,
, ![]() have regular and periodic oscillations and
have regular and periodic oscillations and![]() , so in this case also the functions show uncorrelated behavior. When
, so in this case also the functions show uncorrelated behavior. When![]() , the correlation
, the correlation
functions![]() ,
, ![]() have regular and periodic oscillations, but in this case the functions sometimes show uncorrelated behavior (
have regular and periodic oscillations, but in this case the functions sometimes show uncorrelated behavior (![]() ) and sometimes show correlated behavior (
) and sometimes show correlated behavior (![]() ) periodically. When
) periodically. When
![]() ,
, ![]() , at any time, so in this case the functions show correlated behavior. In Figure 7, we consider (Δ = 0,
, at any time, so in this case the functions show correlated behavior. In Figure 7, we consider (Δ = 0, ![]() ,
, ![]() and the initial state is
and the initial state is![]() ). We investigate the effect of
). We investigate the effect of
the coupling parameters between spins λ1, λ2 on the correlation functions ![]() and
and ![]() for the systems 1 and 2 respectively. The correlation functions
for the systems 1 and 2 respectively. The correlation functions![]() ,
, ![]() have regular and periodic oscillations, the functions sometimes show uncorrelated behavior (
have regular and periodic oscillations, the functions sometimes show uncorrelated behavior (![]() ) and sometimes show correlated behavior (
) and sometimes show correlated behavior (![]() ) periodically. When the coupling parameters between spins λ1, λ2 increase the number of periodic oscillations increases.
) periodically. When the coupling parameters between spins λ1, λ2 increase the number of periodic oscillations increases.
5. Conclusions
In this paper, we analytically solved the model that described the interaction between a three-level atom coupled to two systems of N-two level atoms. We calculated the atomic inversion and the Pancharatnam phase for some special cases of the initial states![]() , and special values of the quantum numbers
, and special values of the quantum numbers![]() , the coupling parameters between spins λ1, λ2. The atomic inversion has regular and periodic oscillations. We observe, at the initial state
, the coupling parameters between spins λ1, λ2. The atomic inversion has regular and periodic oscillations. We observe, at the initial state![]() , when the quantum numbers m1, m2 increase, the phase of periodic oscillations gradually decreases, until the phase of periodic oscillations reaches zero (straight line), but the minimum value increases until it equalizes the maximum value
, when the quantum numbers m1, m2 increase, the phase of periodic oscillations gradually decreases, until the phase of periodic oscillations reaches zero (straight line), but the minimum value increases until it equalizes the maximum value ![]() (straight line). At the initial state
(straight line). At the initial state
![]() , the atomic inversion has small oscillations in the middle of the apexes of the
, the atomic inversion has small oscillations in the middle of the apexes of the
oscillations when the quantum numbers m1, m2 increase, the number of periodic oscillations decreases and the small oscillations disappear gradually. We observe that there is constant interval at the maximum value. It increases remarkably when the coupling parameters between spins λ1, λ2 decrease and the number of periodic oscillations gradually decreases. The Pancharatnam phase has regular and periodic straight lines following the shape of the letter N. We note that there is a regular repeat in the behavior of the Pancharatnam phase. When the quantum numbers m1, m2 increase and the coupling parameters between spins λ1, λ2 decrease, each period of the Pancharatnam phase widens on the time axe and the overall number of periods decreases. Comparing the change of the Pancharatnam phase in Figure 4 and Figure 5, we observe that the effect of the quantum numbers m1, m2 is larger than the effect of the coupling parameters between spins λ1, λ2 in respect to the overall number of periods. Finally, we discuss the second-order correlation function where the examination of the second-order correlation function leads to better understanding for the nonclassical behavior of the system. When the quantum numbers m1, m2 increase the correlated behavior shows remarkably.
The model presented in this paper can further be applied to two-two level atom or two qubits where in this case j = 1. This can make contribution to more understanding and possible applications in the field of quantum optics as well as solid-state physics. In addition, the atom-atom (i.e. spin-spin) interaction is a promising candidate for implementing the quantum computer, which accordingly can be connected vitally to the demonstration of spin dynamics in semiconductor structures [33] .
Acknowledgements
I would like to express my deep gratitude to Professors Abdel-Shafy F. Obada, Mohamed M. A. Ahmed, Department of Mathematics, Faculty of Science, Al-Azhar University and Professor Mahmoud Abdel-Aty, Zewail City of Science and Technology, Giza, Egypt, for their support, care, their useful suggestions, useful discussion and for their continuous help and guidance.