The Optical Properties of Gravity

Bernard Lavenda

Pisgat Shoresh, Apt 634 908600O Shoresh, Israel.

DOI: 10.4236/jmp.2017.85051                

Abstract

The resemblance between the equation for a characteristic hypersurface through which wavefronts of light rays pass and optical metrics of general relativity has long been known. Discontinuities in the hypersurface are due to refraction involving Snell’s law, as opposed to discontinuities in time that would involve the Doppler effect. The presence of a static gravitational potential in the metric coefficients is accounted by an index of refraction that is entirely dependent on the space coordinates. The two-time Einstein metric must be reinterpreted as a two-space scale metric because of the two different speeds of light. It is shown that the Schwarzschild metric is incompatible with the laws of classical physics. Gravitational waves are identified with the transverse-trans-verse plane wave solutions to Einstein’s equations in vacuum, which propagate at the speed of light. Yet, when energy loss is evaluated, his equations acquire, surprisingly, a source term. Poynting’s vector, which is not a true vector, is defined in terms of the pseudo-gravitational tensor, and hence energy is neither localizable nor conserved. The solutions to the equations of motion are geodesics and, by definition, do not radiate. A finite speed of propagation implies that gravitational waves should aberrate, like their electromagnetic wave counterparts, and if they do not aberrate they cannot radiate.

Keywords

Gravitational Red-Shifts, Optical Metrics, Gravitational Geometrical Optics, Gravitational Aberration, Refraction versus Doppler Shift, Two-Time versus Two-Space Metrics, Self-Energy of a Test Particle

1. Introduction

The classical tests of general relativity involving the bending of light in a gravitational field, and the advance of the perihelion of Mercury still form the cornerstone upon which the theory rests. The former has been interpreted by the apparent slowing down of clocks in a gravitational potential, while the latter makes use of the equivalence between geodesics of light-rays propagating in a static gravitational field and geodesic paths of general relativity [1] .

One the one hand, there is remarkable numerical equivalence between predicted and observed phenomena of the two classical tests, yet on the other hand, there are huge discrepancies between the Schwarzschild metric and the laws of classical physics. Moreover, the phenomena have been given inaccurate physical explanations. This is not surprising insofar as the laws of Newtonian physics are independent of location whereas those of general relativity are apparently location dependent. Newtonian theory has no upper bound on the propagation speed, while general relativity has, since it is a generalization of the special theory.

There is even no clear meaning of the line element in general relativity. Einstein generalized the hyperbolic line element of special relativity by allowing the metric coefficients to become space dependent, and, therefore, to depend on the gravitational field. Yet, there are no known solutions to the two-body problem in general relativity, so the generalized line element describes a fictitious mass, or what is commonly designated as a “test” particle. We don’t even know if Newtonian attraction applies in general relativity.

In this regard, an anecdote of Levi-Civita [2] says it all:

During a conversation that I had with Einstein a few years ago, I asked him … if it was possible to give any concrete interpretation … to the elementary chromotopic interval 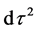… He replied to me that he did not know of any physical meaning…

The saving grace of general relativity, as exemplified in the calculation of the perihelion advance of Mercury, is that geodesic motion can be described by the propagation of wavefronts traveling at the speed of light that happen to coincide with the null geodesics of electromagnetic waves [1] . The equations of motion are formally the same because quantities like the angular momentum are referred to unit mass. Unlike electromagnetic waves, gravitational waves do not possess their own energy-stress tensor like electromagnetic waves so what is actually being propagated are electromagnetic disturbances in a static gravitational field. Poynting’s vector is constructed from a pseudo-gravitational tensor, which, however, can be made to vanish simply through a coordinate transformation. Poynting’s vector is therefore not a true vector, and there can be neither a localization of energy in the gravitational field, nor a direction given to the flux of energy.

Weyl [3] has shown that plane gravitational waves can be classified according to their polarizations. Eddington [4] showed that all but the transverse-trans- verse (TT) type are spurious insofar as they are “merely sinuosities in the coordinate system”, and disappear with a change in coordinates. The TT waves propagate at the speed of light, and Eddington found that light can be identified as a particular form of the TT wave. He also found that light cannot be propagated indefinitely for there was a term in the gravitational potential that blows up so that light cannot be propagated indefinitely in either space or time.

Studying the exact solution, Baldwin and Jeffrey [5] found that an infinite plane electromagnetic wave cannot be propagated without change in waveform. The equations are devoid of both mass and charge, yet the authors refer to them as gravitational waves, if only because they use Einstein’s equation with an electromagnetic energy tensor. They have applied the wrong theory to electromagnetic propagation, and, probably, for this reason, they concluded that “there can be no plane gravitational waves of this type [TT] for which the determinant g [of the metric coefficients] does not vanish…”. Einstein’s equations lead to “the study of divergent waves”, even though there has never been any experimental confirmation. So deeply rooted is the belief that Einstein’s equations have something to do with gravity―even when there is no mass to gravitate. The Riemann tensor is not synonymous to the gravitational field!

Later, Bonner [6] obtained exact solutions to Einstein’s equations representing the propagation of light in an static gravitational field. Although he refers to them as plane-fronted gravitational waves, their sources are entirely electromagnetic in origin.

Weyl [7] was later to realize that in the linearized version of general relativity, which coincides with Minkowski spacetime, the gravitational field exerts a “powerless shadow” on matter. If there were TT graviational waves, they would be powered by a pseudo-tensor that could be made to vanish by a mere coordinate transformation. Moreover, unlike Maxwell’s theory, the equations of motion are not separate entities distinct from Einstein’s equations, so that if the former yield geodesic motion then there can be no loss mechanism like gravitational radiation.

The thesis of this paper is that general relativity describes the propagation of electromagnetic disturbances through an inhomogeneous medium caused by the presence of a static gravitational potential. We will show that the only unambiguous interpretation applies to null geodesics which are derived from an eikonal equation in which the index of refraction is space dependent. Time, or times―whether local or coordinate―have no role in the theory. Rather, the metric has to be interpreted as a measure of the different arc lengths in vacuo and in a medium with an index of refraction. Doppler’s principle, in the form of a second-order frequency contraction, which is the result of setting the space part of the metric equal to zero [8] , is entirely inapplicable, and should be replaced by the law of refraction that relates two different wavelengths, not frequencies.

It is commonly accepted that clocks run slower in a gravitational field. Yet, Einstein’s equivalence principle allows one to replace gravitational acceleration by any other generic one, and it has been emphasized by Einstein himself that acceleration does not affect the rate at which clocks tick. The distinction between local and coordinate times in the metric are interpreted as differences in the paths due to discontinuities in the wave surfaces as light traverses media of different indices of refraction.

In §2, we will review the reasoning that led Einstein to originally determine the bending of light in a static gravitational field. In a series of mathematical manipulations, that maintain the same units but not the same physics, Einstein goes from a system of uniform velocity, whose frequencies experience a Doppler effect, to one of constant gravitational acceleration to, finally, to a static system in gravitational field. More recent authors have followed suit, thereby creating a great deal of confusion. Black body radiation is not something that is experienced by an accelerating observer that vanishes for a uniformly moving observer, or one that is at rest [9] !

In §3 we will repeat Ives’ incomplete derivation of the Schwarzschild metric from the analysis of an interferometer in a gravitational field. We will show that the propagation of a light beam in an interferometer placed in a static gravitational field is analogous to that in a birefringent material whose index of refraction is related to the ratio of distances in Euclidean and hyperbolic spaces. Local time cannot be equated with the length of the arm of the interferometer, and instead of a two-time metric, Ives should have obtained a two-space metric due to the presence of a static gravitational field.

In §4 we will analyze the incongruities when Newtonian forces that act in Euclidean spaces are assumed valid in a hyperbolic space of non-constant curvature given by the Schwarzschild metric. In §5, we will contrast the two-time interpretation of the metric with one of two-space scales, arguing that it is not a Doppler effect, but, rather, one described by Snell’s law of refraction that is caused by the presence of a static gravitational field.

The motion described by general relativity is geodesic, and, by definition, cannot radiate. The coincidence between Einstein’s equations of motion and those of null geodesics of electromagnetic waves has led to the incorrect conclusion that if electromagnetic waves radiate so, too, must gravitational waves. There is no self-energy of a particle in a gravitational field like there is in an electromagnetic field.

A well-defined energy stress tensor for electromagnetic waves does not carry over to their gravitational analogue; as Einstein [10] himself has shown, at most one can define a pseudo-tensor which can be made to vanish by a mere change of the coordinates. The analogy between electromagnetic and gravitational waves meant that the two must travel at the same speed. This implies they must show the same phenomena due to delayed action. However, gravitational aberration and diffraction have never been observed, even though, the more difficult phenomenon to detect, gravitational waves, have [11] .

In §6 we claim that since gravitational waves do not aberrate they cannot radiate; radiation occurs in the direction of the Euler force. A fortiori, they would need a Poynting vector to determine the direction of energy flow, and this would require the analog of a magnetic field, the so-called gravitomagnetic field [12] , which has never been observed. The linearized version of general relativity should be identical to Maxwell’s theory. Our conclusions are summarized in §7.

2. Einstein’s Gravitational Doppler Effect Analogy

By replacing the velocity in the Doppler expression for the frequency shift by the product of gravitational acceleration with time, and then replacing the latter by the ratio of the distance that light traverses in the given time interval, and the speed at which it propagates, Einstein was able to convert a uniform velocity into a completely static expression. The only common thread in the three expressions is the same units. However, it implied that a static gravitational field can cause a frequency shift [13] , and it should make clocks run slower.

Although it could have been derived simply by appealing to the viral theorem, it points to the fact that a medley of different physical situations can be used to transform the Doppler effect into something completely foreign to it. This has led to many completely erroneous claims like accelerations can cause Doppler shifts [14] , and the universal law of gravitation can become repulsive at a critical speed in the Schwarzschild metric [15] [16] .

When we realize that the sole generator of the Doppler effect is the uniform velocity of a body then any reference to acceleration, or to a static gravitational field, as the cause of the frequency shift is patently false. The Doppler effect maintains a constant speed of light so that any change in the frequency must be compensated by a corresponding change in the wavelength of light in order to keep their product constant. Other optical phenomena, like refraction, permit either frequency, or wavelength, to change at the expense of a constant speed of light, c.

When c is not a constant, we can have a stationary, but inhomogeneous medium, or a homogenous but non-stationary one. The gravitational field, 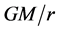belongs to the former category, as Eddington well-appreciated long ago. For, according to Eddington [17] , the bending of light as it grazes a massive body M can be likened to light moving

more slowly in a material medium than in vacuum, the velocity being inversely proportional to the refractive index of the medium. The phenomenon of refraction is in fact caused by the slewing of the wave-front in passing into a region of smaller velocity. We can thus imitate the gravitational effect on light precisely, if we imagine the space round the sun filled with a refracting medium which gives the appropriate velocity of light [my italics]. To give a velocity of, the refractive index must be

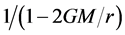, or, very approximately,…

Any problem on the paths of rays near the sun can … be solved by the methods of geometrical optics applied to the equivalent refracting medium … the total deflection of light passing at a distance r from the centre of the sun is

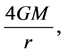
whereas the deflection of the same ray calculated on the basis of Newtonian theory would be

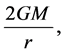
[in units where].

The factor of 2 is precisely that required to convert the angular velocity of a Keplerian orbit into an escape velocity.

We will argue that the tortuous derivation of Einstein [13] to derive the gravitational red-shift from the classical Doppler effect is an inaccurate descri- ption of the phenomenon. This will vindicate Essen’s [18] criticism that one cannot replace the velocity in the Doppler expression by an acceleration, then by a static potential and to refer to the result as a frequency shift derived from the Doppler effect. If velocity could be replaced by acceleration in the classical Doppler effect, the Hawking expression for the absolute temperature in terms of the surface gravity of a Schwarzschild black hole, or more generally the Unruh [9] expression for the same temperature in terms of any generic acceleration, could be justified on the formal similarity to the Planck factor in the black-body spectrum [14] . However, the Planck factor is only half the story because what is missing is the correct density of states so that integration over all frequencies does not give back Stefan’s T⁴-law for a black body [19] .

However, Einstein [13] was right about the non-constancy of the speed of light in a gravitational potential, albeit for the wrong reason. He argued that by using clocks of “identical constitution” we must find the same speed of light at all points in the “accelerated, gravitation-free system”. How clocks measure the “same magnitude” is not explained, but, according to Einstein this should also hold true in the non-accelerated system as well. He then claims, without justification however, that

from what just has been said we must use clocks of unlike constitution, for measuring time at places of different gravitational potential. For measuring time at a place which, relative to the origin of the coordinates, has a gravitational potential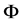, we must employ a clock which―when removed to the origin of the coordinates―goes 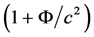times more slowly that the clock used for measuring time at the origin of the coordinates. If we call the velocity of light at the origin of the coordinates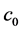, then the velocity of light 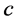at a place with the gravitational potential 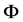will be given by the relation

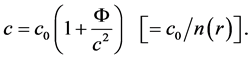(1)

The principle of the constancy of the velocity of light holds good according to this theory in a different form from that which usually underlies the ordinary theory of relativity.

By writing down (1), Einstein was admitting that the velocity of light has been decreased by an amount 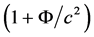due to a refractive index

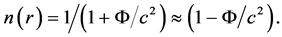(2)

However, (1) has nothing whatsoever to do with a frequency shift given by the classical Doppler effect,

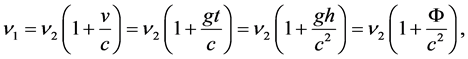(3)

where g is the gravitational acceleration at the surface of the earth, 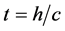is the time interval that the radiation is required to transit a distance h, and is the statistic gravitational potential. The height of the drop of photons, h, has been purposely confused with r, the radial distance between the body of mass M, and that of a “test” body which is non-existent. In effect, Einstein was confusing the motion of a body of velocity, v, with respect to a stationary system, with the speed of light that differs from c such that their product,

(4)

This is not not Kepler’s third law. The seeming analogy with the Doppler effect has allowed Einstein to introduce the discrepancy in the rates of clock motion in a gravitational field and in its absence. The Doppler effect has absolutely no place in the discussion, that can simply be treated as the propagation of light from the vacuum to a medium of index of refraction (2).

If it were the Doppler effect, the conservation of energy and momentum would have to be satisfied. Einstein writes the conservation of energy as

where the right-hand side represents “the increase in gravitational mass…, and therefore equal to the increase in inertia mass as given by the theory of relativity”.

The conservation of energy, according to Einstein’s Doppler principle is

(5)

where h is Planck’s constant. In addition, we must also have the conservation of momentum,

(6)

where m must be the peripheral mass that does not appear in Kepler’s law. Dividing (5) by (6),

(7)

where we imposed Einstein’s stationary condition, (4). There is no way that (7) can be satisfied so that we come out with

(8)

―even as an approximation. Einstein’s entire analogy with the Doppler effect, and, consequently, with it the different rates of ticking of clocks, is vacuous.

Rather, by writing down (1), Einstein has implicitly admitted that he is treating an inhomogenous, static medium that is amenable to Snell’s law of refraction. All what he said previously is completely superfluous to what follows, and by using instead of twice its value he comes out with half of the deflection of light that he will find several years later from his general theory. The last sentence of the quotation is a non seguitur since he has, otherwise, found himself in contraction with his second principle of special relativity which makes c a universal constant.

Snell’s law reads

(9)

where the angles of incidence, , and refraction, , are measured with respect to the normal. Since the frequencies must be the same for each of the two waves that have wavelengths, and, in the two media. The difference in the wavelengths does not give “the angle through which the light ray is deflected in the path”, where T must be identified as the period of the wave irrespective of the medium in which it is in. Only for small angles will the difference in sines be equal to the difference in the angles, , but, then, there is an undetermined constant which enters the proportionality so nothing can be said about a specific value of the deflection of light.

Einstein’s relation is completely fortuitous, and his result from his general theory of relativity cannot be related to the difference between proper and world times since, as we have shown, time and the rate of ticking of clocks do not enter into the physics of the problem.

3. Schwarzschild Metric from an Analysis of an Interferometer in a Gravitational Field

Ives’ [20] derivation of the Schwarzschild metric from an analysis of an interferometer placed in a static gravitational field shows clearly the optical roots of the Schwarzschild metric. The medium appears as a birefringent material with different indices of refraction depending on the direction of the gravitational field.

The length of the interferometer supposedly undergoes a Lorentz contraction by a factor,

(10)

where c is the speed of light, and V is the “escape” velocity

(11)

Reference to motion is completely superfluous, what Ives needs is the static contraction factor in (10).

The length of the stationary interferometer, L, will only be contracted in the direction of the field,

but not in the direction normal to the field. Frequencies will be shortened by the same factor while the period of the interferometer in a stationary field, , will be lengthened by the amount,

Ives first considered the interferometer in a “stationary” gravitational field where one arm is in the radial direction, while the other arm is normal to it. Light sent out and reflected from a mirror in the normal direction will take a time for it to return, where is the speed of light in the transverse direction.

In constrast, the time taken for light to make an out-and-back journey in the radial direction is, where is the velocity of light in the radial direction, and we have taken into account that the arm will be contracted in the radial direction. These times should be the same, and both should be equal to the clock interval,:

From this it follows that

(12)

Everything can be explained without recourse to contracted motion simply by considering a birefringent crystal with two indices of refraction: the index of refraction of the ordinary wave, , and the extraordinary one, so that. It would also appear that the light velocities (12) are “static” insofar as they do not involve the velocity of a moving platform.

Ives was also under the mistaken impression that the “ticks” on a clock should be altered when placed in a static gravitational field. Based on the result he wants to derive, namely, the Schwarzschild metric, he claims that the frequency of a light ray in the presence of a large mass will be “red-shifted”.

Ives then imagined that there is a hollow spherical sphere encompassing a diagonal mirror of the interferometer. When the gravitational field is turned on, the sphere of radius a becomes an oblate ellipsoid, with radii a, a, b, where. The time taken for a light signal to traverse the ellipsoid in the direction of the field is, while in the direction normal to the field, the time will be. Hence, and. All this occurs without the recourse to a moving platform on which the interferometer has been placed.

Ives went on to consider the speed of light along any radius r making an angle with the direction of the gravitational field. The ellipsoid can now be considered an ellipse with semi-major axis a, and semi-minor axis, b. The equation of the ellipse in polar coordinates is:

(13)

The velocity of light in any direction is, and making the above substitutions, Ives came out with

(14)

It is evident that if the radius is in the direction of the field, , and the speed of light is, while normal to the field, , and the speed is.

In terms of the polar coordinates, , the increment in the path of the interferometer is

so that its velocity will be. The length of the arm of the interferometer in any direction will be given by (13) with

so that

Ives then claimed that the velocity of light, , at an angle is given by

(15)

Why he has changed nomenclature is that from (14), so that from (15), which contradicts his result,

. All this is to get the desired result.

The generic speed (14) can now be expressed as:

(16)

The ratio,

(17)

is an index of refraction measuring the difference between Euclidean, , and hyperbolic, , lengths. It is equivalent to considering the ratio of the magnitude of orthogonal directions of trajectories to two contiguous surfaces separated by a discontinuity as the definition of the relative index of refraction [21] .

For (16) is c times the ratio of the Euclidean distance

and

(18)

is the hyperbolic distance of the well-known Beltrami metric, although it will not be of constant curvature unless has a specific form [22] . Ives has in place of in (16) because he knows the answer he wants, i.e., the Schwarzschild metric.

Instead of (15), the ratio of the velocity (16) to the radial coordinate r should be the same ratio as c to arm of the interferometer, L, viz.,

(19)

Thus, relative speed is

The time taken to complete the out-and-back journey in any arbitrary direction is

(20)

on the strength of (19). However, Ives uses (15) which replaces by in the denominator of (20).

If somehow could be identified as the proper time interval, , then (20), with standing in for c, would coincide the Schwarzschild metric,

(21)

Ives clearly realized this is the weak point of his derivation. As he openly admitted:

The identification of with the (undistorted) length of the inter- ferometer must be pondered, in connection with the somewhat mystical character which is ordinarily ascribed to this quantity using formula [(21)] to derive the motion of a particle in a gravitational field.

So what does (21) describe? According to Levi-Civita [23] :

Even the two-body problem, solved long ago by Newton, has very little chance of being solved successfully in general relativity. This is because, in the relativistic scheme, the reaction principle no longer holds, and we do not even know how to begin going about the reduction of the partial differential equations to ordinary differential equations―let alone integrate them.

Moreover, if the correct relation, (19), were used, the indefinite metric would be:

(22)

However, as far as the optical metric, , is concerned, (21) and (22) are indistinguishable since both yield the Beltrami metric, (18), when the mass M is replaced by the mass density, so as to get a hyperbolic disc of constant curvature [22] . This also has the effect of transforming Schwarzschild’s outer metric into his inner metric without even a hint of a black hole! The latter supposedly arises when r is made less than 2M which physically invalidates the hyperbolic metric. It would be the same as insisting that in the inner solution: r cannot be made larger than the radius of the hyperbolic disc!

It should be clear from Ives’ derivation of the Schwarzschild metric that two times, local, , and coordinate, t, are not involved. Time enters only in the measurement of lengths of trajectories: the trajectory where the speed of light is c, whose length is the Euclidean distance, , and the hyperbolic path length, , whose indices of refraction are (12), or and, depending upon the orthogonal direction. Recourse to an “escape” velocity is simpy a deus ex machina for introducing the contraction factor, , and no inertial motion of the interferometer was intended.

4. Gravitational Repulsion and Centrifugal Attraction

It is truly amazing that the metrics of general relatively can yield such precise values for the advance of the perihelion of Mercury, and the gravitational deflection of light by a celestial body, when the Schwarzschild metric cannot even distinguish between proper and coordinate time in deriving Newton’s law and Kepler’s second and third laws, predicting gravitational repulsion for velocities surpassing a critical value, and the existence of a critical radius where the centrifugal force becomes attractive. The interpretation of r as a radial coordinate is also troublesome since is impossible. It would appear that whereas Newton’s and Kepler’s laws are not position dependent, those of general relativity are. We will show here that there is no common ground between the two.

We will see that no matter how hard authors have tried to unite the Schwarzschild metric with the dynamical laws of physics it is impossible. Since discontinuities appear, the two-time interpretation of the metric is at fault and one must consider different measures of path lengths when different indices of refraction are present.

The Schwarzschild metric is both diagonal and time independent. If we write the Schwarzschild metric in polar coordinates, and avail ourselves of the symmetry that geodesics lie on a plane through the origin, then choosing the equatorial plane, gives the Schwarzschild metric (21).

The equations of motion for a free particle in general relativity are:

(23)

where are the Christoffel symbols of the second kind. The geodesic equations, (23), can be written more like an eikonal equation by using the components of the metric tensor themselves [24] ,

(24)

The equation of motion for the radial coordinate in the equatorial plane, , is:

(25)

where the prime stands for differentiation with respect to r.

The pure radial part of the equation of motion is obtained by setting in (25), viz.,

(26)

where the dot denotes differentiation with respect to coordinate time, t. Inserting the Schwarzschild metric (21) for radial motion,

gives:

(27)

which resembles Newton’s law but is not Newton’s law because it is expressed in proper time, , and not in coordinate time,.

Multiplying both sides of (27) by and integrating yields

(28)

apart from an arbitrary constant of integration we have set equal to zero. Hence, as a consequence of Newton’s law in proper time, (27), we get expression (28) for non-relativistic virial, also in proper time. This is indeed surprising since Newtonian mechanics knows no limit on the speed of particles, and, yet, general relativity predicts a critical speed, [cf., (35) below]!

Introducing this expression into the radial Schwarzschild metric,

(29)

gives:

(30)

after rearranging and taking the positive square root. This is not the same expression that would be obtained by considering only the temporial component of the Schwarzschild metric [8] . Since two times are involved in (30), it can rightly be considered as the classical Doppler shift due to a static gravitational field.

The velocity,

(31)

exists for all, while the acceleration,

(32)

is negative, and, hence, attractive, for, while it becomes positive, and, hence, repulsive in the interval,

(33)

For, (32), reduces to its Newtonian value,

(34)

Hilbert [15] cast the condition of gravitational repulsion, (33), in terms of a critical value of the velocity,

(35)

where is the radius for which the coordinate acceleration, (32) vanishes. Hilbert found that the speed has its maximum at.

It appears, however, that the first to introduce the notion of gravitational repulsion was Droste [25] . Droste came out with (32), which Drumaux [26] tried to remedy by considering

(36)

as the physical definition of the length. He then considered the actual time to be the “free-fall” time ():

(37)

and derived the “renormalized” velocity:

(38)

and acceleration:

(39)

in proper time.

However, it is not the proper time (30), and has nothing to do with the Schwarzschild metric since it is defined by (37) having set. True, the repulsion appearing in (32) has disappeared in (39), but what is the sense of expressing the velocity and acceleration in terms of R when the equation is given in terms of r, and there is only a differential relation between the two?

The distinction between local and coordinate times couldn’t be more paradoxical than in the case of purely rotational motion. When, the equation of motion (25) reduces to:

(40)

Neglecting the minor point that the Schwarzschild radial coordinate is not the Newtonian radial coordinate, (40) resembles Kepler’s third law, , where the angular velocity is defined in coordinate time, , and not by proper time,.

Consequently, the Schwarzschild metric, (21), yields two laws of classical physics, Newton’s, (27), and Kepler’s, (40), but with two different times!

Kepler’s third law, (40), can be extracted from the radial equation,

(41)

which reduces to

(42)

for a circular orbit, , in the equatorial plane,. Equation (42) is the same as Kepler’s law, (40), for coordinate time.

Yet, for Kepler’s second law, (24) gives:

(43)

The condition for a central force to hold is that Kepler’s second law has to be obeyed. Notwithstanding the claim that (43) is Kepler’s third law, and not Kepler’s second, Birkhoff [27] sees no problem in expressing (43) in “the local time at the particle”. Yet, the distinction between attractive and repulsive gravity has been made in coordinate time in (32), which favors von Laue’s Systemzeit “t” over Lorentz’s local time, “”, as the true time coordinate determining the dynamical evolution of the system.

Birkhoff also sees no problem in determining the time coordinate t by sending out radial light signals from the center. Yet, the coordinate velocity, (31), goes to zero at, while the velocity, as seen by our test particle, remains constant everywhere, and not just at the horizon, [28] .

The equation for the geodesics, (24), also gives

(44)

where k can be considered as the conserved energy when it is associated with the fourth component of a 4-velocity. This constant scaling factor is usually set equal to one for radial motion [29] . Combining (44) with the pure radial part of the Schwarzschild metric that was used in (26) gives

(45)

If the left-hand side is interpreted as the “radial” kinetic energy per unit mass [24] , (45) is off by a factor of 2 from the non-relativistic virial theorem.

Even worse, the radial equation of motion, (41), can be written explicitly as:

(46)

The left-hand side of (46) is the Schwarzschild radial acceleration, , and it can become positive for radial velocities greater than

(47)

implying gravitational repulsion.

Ohanian [30] contends that the direction of the force is always determined by the proper acceleration, (27), and is always attractive, i.e., directed downward. If so, why talk about attractive and repulsive gravity in (32)? In particular, for light

(48)

where the critical speed is (35), so that light would always be gravitationally repulsive, having a positive acceleration,

(49)

This would imply that light is bent backward away from the mass M which it grazes.

However, Einstein [13] found the angle of deflection positive meaning that the light ray will be bent toward, and not away from, the massive body. For, according to Einstein,

…a light ray passing along by a heavenly body suffers a deletion to the side directed toward the heavenly body.

It seems difficult then to accept that “light is repulsed everywhere, …increasing from zero at to 1 at” [31] .

The proper velocity is unity differing from the coordinate velocity by a factor, so that the proper acceleration vanishes, as it should for an object travelling at constant speed. Since photons possess a mass equivalent, shouldn’t they behave the same as our “test” particle?

Another disturbing feature is that that the speed of light should be frame independent, and here it is clearly not. In the laboratory frame the speed is (reinstating c), where, the index of refraction, while the proper speed is c with. Are we to believe that the transition from coordinate to proper time is equivalent to the transition between vacuum and a medium with an index of refraction, n?

Moreover, if light has mass equivalent, why should it be always repulsive in contrast to particle behavior? The deflection of light by a central mass would imply that light is attractive, and not repulsive. The fact that light is always repulsive and increases from 0 at to 1 at implies that we are dealing with a refraction phenomenon, and not a Doppler shift in the frequency.

It would not be unreasonable to question the fact that since the index of refraction is the ratio of the coordinate time to the proper time, according to (44), that is a camouflaged way of writing the classical Doppler shift. In fact, Møller [8] derives the frequency shift in a static gravitational potential by considering only the temporal component of the metric. However, two things should be observed: 1) for null geodesics like light rays, and 2) it is the square root of, and not, that appears in the equation. For weak fields, the quantity is reduced to half its value when the square root is approximated by its first two terms.

For light waves, the proper time interval vanishes. Since t is a measure of the distance along a light ray in units of the speed of light, we may introduce a geometrical length s of the light ray by using the relation so that [21] . The equation of motion of the radial coordinate (41) is thus equivalent to the eikonal equation of ray optics [29] :

(50)

where the only independent parameter measuring the length along the light rays is s which replaces the proper time.

More recent controversies regarding the existence of gravitational repulsion have arisen [32] . According to Ohanian [30] ,

the discrepancy between the signs of the accelerations of low-speed and high-speed particles is a perplexing violation of the equivalence principle. General Relativity attributes this discrepancy to a bad choice of coordi- nates―the coordinates r and t do not represent locally measured distances and times.

Reference to the equivalence principle is a red-herring, for we ask: equivalence of what? Reference to local coordinates is equally as bad: the Schwarzschild metric could not be more democratic for it gives us Newton’s and Kepler’s second law in proper time, while, simultaneously, it gives Kepler’s third law in coordinate time for circular motion!

Ohanian also misconstrues attraction and repulsion with acceleration and deceleration. Newton’s law has the same form as Coulomb’s law (which was Coulomb’s impetus for his derivation). There is one sign to Coulomb’s law for unlike charges and the other for like charges. The signs refer to attraction and repulsion, respectively.

Ohanian further faults Hilbert, who, “being a better mathematican than a physicist”,

unfortunately misconstrued this deceleration as a repulsive gravitational force… Hilbert naively assumed that the force is in the direction of the coordinate acceleration, whereas he should have known that the force is in the direction of the rate of change of the relativistic momentum,

(51)

which is always negative. Therefore the direction of the force (and also the direction of the proper, or relativistic acceleration) is always downwards, that is, the force is always attractive.

Reference to the rate of change of the momentum (51) is also a red-herring: the peripheral mass m is velocity independent so that it reduces to the acceleration (27). In the law of gravitation the peripheral mass does not appear at all. It means that the acceleration at any point in the field depends only on the point where the particle is situated and not on its mass. Moreover, if the momentum is a function of the proper time, so too should the angular velocity, and from (40) we know that it isn’t since otherwise we would not get Kepler’s law.

The confusion of what is the angular velocity leads to other incongruous results. The Schwarzschild metric can be cast in the form

(52)

Assuming J is angular momentum where is defined as, instead of, and it is somehow conserved, taking the derivative of (52) with respect to proper time, , we get, after simplification,

(53)

In comparison with “Newton’s” law, (27), there is now the additional term which looks like centrifugal force, except for a coefficient which can be both positive or negative. Both Hilbert and Einstein claimed that the radial coordinate of a circular trajectory must satisfy

(54)

in order that the centrifugal force be repulsive. However, the left-hand side of (53) does not represent acceleration, just like J does not represent angular momentum per unit mass.

Hilbert’s inequality (54) follows directly from the Schwarzschild metric,

(55)

for circular motion in the equatorial plane by dividing through by, and introducing Kepler’s law, (40). We then obtain

(56)

which clearly shows that inequality (54) must be strictly obeyed for circular orbits.

Although Kepler’s law does not condone restrictions on the radial coordinate, the Schwarzschild metric does! The inverse of the positive root of (56) can be considered as an index of refraction in the direction of rotation, just as the first expression in Ives’ expression (12). The times are proportional to the path lengths; there will be two of them: one for light in vacuum and the other in a medium of index of refraction, , according to (56).

The change in definition of the angular velocity, and the unwarranted conservation of the pseudo-angular momentum, (43), deform an otherwise circular orbit and, not unsurprisingly, predict the advance of the perihelion. Although the numerical result coincided with the advance of Mercury it did not fare as well with the other planetary orbits, which differed in all cases in magnitude, and, in some cases, even in sign [33] .

This, supposedly, applies to circular orbits for which. It is clear that there are no circular orbits for “a geodesic photon trajectory at”¹. It has even been claimed that the Rayleigh condition for orbital stability for requires angular momentum to be decreasing outwards [35] .

Inequality (54) has nothing to do with the centrifugal force changing sign and becoming attractive. Rather, it has to do with the curvature of the metric [22] . If we consider, instead, the Schwarzschild inner metric, ², the condition for “Rayleigh” stability would be

(57)

where is the Newtonian free-fall time. When (57) is ceased to be obeyed, the central mass, whose density is, would lose mass because it would be torn off by the centrifugal force. The centrifugal force does not change sign―either for the external, or internal, Schwarzschild solution.

Inequality (54) can be traced back to Hilbert [15] , which was later confirmed by Einstein [16] , long before [35] . Einstein obtained inequality (54) by employing isotropic coordinates in which the radial coordinate, , in is generalized to:

where. Notwithstanding Einstein’s reference to a stationary system with “many gravitating masses”, it has been repeatedly emphasized that the only known solutions to Einstein’s equation involve a single stationary mass [36] . How numerical relativity can treat binary systems, or the collision of two masses, is an enigma.

Parenthetically, we mention the black hole “excision” technique has been suggested [37] , and used in numerical relativity to remove the interior of the horizon while “still being able to obtain valid solutions outside the horizon”. They justify it by claiming that the event horizon disconnects causally the interior from the exterior. However, excision removes the invented problem entirely, which seems more like exorcism! An inhabitant of the hyperbolic plane can never reach the rim of the hyperbolic disc because his steps decrease in size like the measuring rods used to measure them [22] .

Notwithstanding how many “advances” in numerical relativity that have been made over the past two decades, and their supposed final experimental vindication in the LIGO claim of the observation of gravitational waves [11] , they cannot camouflage the nonsensical idea that the Schwarzschild external solution can be prolonged into the the region. This is highlighted by the so-called “puncture” technique [38] whereby the solution is factored into an analytical part containing the black hole singularity, and a numerical part which is singular free! This would be enough to make Schwarzschild turn over in his grave!

Instead of using the equation of motion (25), one [17] normally starts with the Schwarzschild metric (21) written in the form:

(58)

and using the first integrals, (43) and (44). J would be the conserved angular momentum in local time, but not in coordinate time, and k is an arbitrary constant of integration in (44), which we shall appreciate is not so arbitrary insofar as it is related to the total energy of the system. This is in contrast to the arbitrary constant of integration that emerges from the integration of Einstein’s equations under the condition of emptiness which is identified as the mass of the “empty” space.

With the aid of the first integrals, (43) and (44), (58) can be written as:

(59)

where an index of refraction has been defined as:

(60)

Light rays in this medium, with refractive index (60), are identical to the paths of particles that move in a Newtonian potential where [21] . The coincidence between light and particles exists because J is the angular momentum per unit mass, so that the peripherial mass never appears explicitly, just as it cancels out in Newton’s law relating the force to the gravitational potential.

The energy is. Neglecting the small term, , which is responsible for the deflection of light by a body of mass M, the curves of the paths of light rays will turn out to be conic sections which degenerate into parabolas in the limit. The index of refraction (60) then reduces to the usual form [39] , , and the equation of the orbit reduces to:

provided the small perturbation can be neglected. The solution is a parabola:

with its focus at the origin. The integration constant has been chosen so as to make r a minimum at. The light trajectories are indistinguishable from those of particle trajectories so long as we interpret J as the angular momentum per unit mass.

The Hamilton-Jacobi equation of geometrical optics is [21] :

(61)

where the indices vary over the space coordinates, and the index of refraction, n, is given by (60). If the all reduce to constants, (61) describes the propa- gation of electromagnetic wavefronts. The fact that the contain a static gravitational potential does not alter the nature of the wavefronts, other than to introduce inhomogeneities, and possible discontinuities. The same could be achieved when the wavefronts pass through any refracting surface. The origin of discontinuities lies on the right-hand side, the index of refraction, and not on the left-hand side, of the Hamilton-Jacobi equation, (61).

For a relativistic system, the right-hand side of (61) would be equal to the square of the peripheral mass [40] , which doesn’t exist in general relativity. We have said that the proper time vanishes for null geodesics, but not those that propagate through a medium with a different index of refraction. The proper time is the length of the path that the light traverses in the medium, while the coordinate time is the length of the same path in vacuum.

This resolves Ives’ conundrum of setting the length of the interferometer equal to a time interval. As far as gravity is concerned, the general relativistic metric does not relate proper time to coordinate time, but, rather, it relates two path lengths, or wavelengths, that occur when light traverses an inhomogeneous medium whose index of refraction is proportional to a static gravitational field.

In this case (60) reduces simply to:

(62)

or, equivalently,

(63)

upon taking the positive square root, where, the wavelength in the medium of the index of refraction, , while is the wavelength of light in vacuo. Then (63) is just Snell’s law,.

A light signal travels through space over a set of wavefronts:

(64)

that propagate at the speed of light, c, where S appears as Hamilton’s charac- teristic function. If they were particle trajectories then we could have some grounds of believing in gravitational waves. But there is no peripherial mass to be found. Whittaker [1] showed that the Hamilton-Jacobi equation (61) is identical to the electromagnetic field in Riemann spacetime with an indeter- minate metric tensor,. Levi-Civita [41] derived the characteristics of (61) which are light rays propagating in a medium of index of refraction, (60).

The fundamental distinction between electromagnetic and the putative gravitational waves is that the latter are not known to refract. In the presence of media with different indices of refraction, the characteristic hypersurface

in spacetime has electric and magnetic fields which are dis- continuous. Although this hypersurface is continuous, it does not necessarily have normals to the surface that are continuous. Yet, is a continuous solution of the Hamilton-Jacobi Equation (61), with sectionally continuous deri- vatives, even on the hypersurface where n is discontinuous [21] . The gravita- tional field does not have similar traits in being discontinuous and manifesting diffraction phenomena.

Even though Eddington [17] drew the analogy between optical and gravita- tional phenomena, he was not prepared to “contemplate a discontinuous trans- formation of coordinates”; otherwise, there is a “complete arbitrariness that allows motion to be brought to rest”. As Weyl [3] emphasized, every moving body can be brought to rest through a suitable change of the coordinates. How- ever, we are not contemplating motion, but, rather, the discontinuity of wave surfaces along which light rays propagate due to a change in the index of refrac- tion.

5. Doppler versus Snell

The fundamental property of light is its frequency. The wavelength of light keeps changing as light passes through different media having different indices of refraction which depend on the spatial coordinates. This guarantees that the ticks on a clock do not get lost as we pass from one frame to another. Even the eikonal approximation makes a demand on the wavelength irrespective of the frequency. Its validity requires that the wavelength change very slowly in com- parison to the characteristic dimension of the system. In contrast, there is no condition on the frequency that the wave can assume. The wavelength becomes proportional to the velocity of the electromagnetic wave, and, hence, inversely proportional to the index of refraction.

The relation between wavelength and the index of refraction is given by Snell’s law:

(65)

The index of refraction for the Schwarzschild metric is usually identified as:

(66)

which is a function of the radial distance, r, and differs from (2) by the famous factor of 2. However, we can consider the non-static case in which r remains constant, and the mass is a function of time [27] . The wavelength will not change because the leading and trailing edges of the wave encounter the same index of refraction at each instant of time; the index of refraction is a function of the time coordinate,. Hence, in this case, the frequency, and velocity of light, , will vary so as to leave the wavelength,

invariant. Introducing leads to Snell’s law,

(67)

for the frequency.

Again consider the Schwarzschild metric (21), but this time consider the system stationary, so that it reduces to

(68)

How do we know that (68) is not

(69)

on account of (11) for the escape velocity?

If an atom is emitting at a “proper” frequency, which is the number of light waves emitted in a unit time interval, then the number of light waves picked up by a standard clock at rest will be

(70)

If more pulses are transmitted than received then some pulses are lost [18] . Where have the pulses gone?

If we introduce (11) into (70) it will look like a second-order Doppler effect,

(71)

with the escape velocity, V, replacing the velocity in Kepler’s third law. What about the first-order Doppler effect? It is just a quirk that the time component of the Schwarzschild metric, (68), can be made to look like the Lorentz invariant definition of local time.

Another point is that (71) is not obtained from (70) for a system at rest. The spatial part of the metric is involved since it defines the velocity, but it is clear that it is not the Schwarzschild metric that is involved.

One could rightly argue that the calculation of the total transit time, (37) has no meaning since has no meaning. We could rather write (71) as the arithmetic average of forward and reverse, , Doppler shifts

(72)

Buy why should we contemplate an average of the forward and reverse Doppler shifts on going from coordinate time to proper time?

In other words, what is the relation between the indefinite spacetime metric and (72)? It is usually assumed that and represent inverse times, and

(73)

represents the time component of the metric under stationary conditions,. How can this be with? Moreover, (72) is valid for whatever c turns out to be. Finally, will be time-independent if V is [cf., (86) below].

If we consider, along with Faraday, two charges of opposite sign in relative motion, lines of force will emerge from the positive charge and terminate at the negative charge. If the negative charge is removed the lines of force on the positive charge in motion must terminate somewhere. Since the positive charge is confined by the walls of the room, the lines of force must terminate there. So without reference to the walls of the room there can be no Lorentz contraction.

We do not know what changes in frequency have to do with a stationary gravitational field, but we do know what Snell’s law is. If the refractive index can be factored into the product of spatial and temporial terms, then the time component will be equal on both sides of the interface so that it will have no effect on the change in wavelength as the rays cross the interface.

When a light wave travels from a low to a high index of refaction, the wavelength decreases so as to satisfy Snell’s law:

(74)

As the wave begins to coil up in the medium with the higher index of refraction, both and change but the frequency remains constant. Light pulses don’t get lost. The time component on both sides of (74) are the same and cancel out.

Can we imagine a situation in which the index of refraction is the same everywhere at any instant in time and yet is a decreasing function of time? If so, the leading and trailing edges of the wave will not encounter any change in the index of refraction so the wavelength remains the same but the number of pulses will change because the index of refraction is a decreasing function of time. We should thus expect a law of the form (67) because the and change but does not.

Consequently, (70) makes absolutely no sense: A static gravitational field has absolutely no effect upon the frequency. In other words, defining a velocity in (72) as would violate under time reversal. Then why does (74), with, appear to conform to experimental results?

If it is assumed that c is a constant, and vary inversely with respect to one another, and the same inverse relation applies to the γ’s in the coefficients of time and radial coordinates.

General relativity makes no qualms about being able to measure coordinate times and distances. The “metric” frequencies and wavelengths are obtained from the “locally” measured time intervals and (radial) space intervals, , of the metric. Since the Schwarzschild metric respects the inverse relation between and, at constant c, the blue-shift of the wavelength can be confused with the red-shift of the frequency.

According to Einstein, “nothing compels us to assume that clocks in different gravitational potentials must be regarded as going at the same rate”, but, by the same token, nothing compels us not to do so. Since we know from the work of Ives and Stilwell that velocity affects the ticking of a clock [18] :

There is no logical reason, nor any physical explanation, why it should be in any way caused by the acceleration that produces the motion? There is therefore no logical reason why it should be caused by a gravitational potential, which is assumed to be equivalent to acceleration.

Evidence supporting that the rate of a clock is impervious to accelerations comes from storage ring experiments on muons up to accelerations of the order of 10¹⁹ g. It is astonishing that Einstein, in one breath, should argue that accelerations should have no effect on the rate of a clock, while, in the next breath, he should argue that a static gravitational potential should affect the rate of ticking of a clock. What about his cherished equivalence principle?

It has been argued that “it does not matter if we put the refractive index n in the space part or its inverse in the time part, the speed of light is the same and so is the geometry” [42] . Apart from the fact that the Schwarzschild metric does not subscribe to this conformal invariance, the invariance is based on the conformal invariance of Maxwell’s equations in the absence of source terms. A geometry that mixes space and time mixes electric and magnetic fields. It is supposedly based on the invariancy of the electromagnetic waves. Inhomogeneous media with space dependent indices of refraction destroy space-time invariance as witnessed by Snell’s law [cf., (74)].

The contradiction between frequency shifts in Doppler’s law and wavelength variations in Snell’s law could not be made any more apparent than to consider the Schwarzschild metric in the equatorial plane. The Lagrangian describing this geometry is given by [43]

(75)

The dot denotes differentiation with respect to an affine parameter because the proper time parameter cannot be used as a differentiation variable since for null geodesics (light rays). must be chosen such that the null vector preserves its length under parallel transport [44] .

The variational principle,

(76)

yields the standard equations of motion, and determines up to a linear transformation of [44] . Because is time-independent, coordinate time t will be a cyclic variable along with the azimuthal angle,. Consequently, two first integrals of the motion are expected:

(77)

(78)

The Euler-Lagrange equations are, thus, given by [43] :

And the equation for the ray trajectory,

(79)

describes closed Keplerian orbits that are conic sections when. Precisely when, we get the “relativistic” corrections of the advance of the perihelion and the bending of light.

The constant in (77) represents the conservation of energy. It replaces the index of refraction in (79) [cf., (59)]. This would identify energy conservation with spatially homogeneous systems. It is well-known in general relativity that energy cannot be localized; but it is immaterial whether the system is homogeneous or not.

Any static spacetime metric, like Schwarzschild’s metric (21), gives the projections of light rays onto spatial sections that are geodesics of the Fermat, or optical, metric,

(80)

which is a positive-definite line element on a 2-dimensional surface with what was proper time, t, now serving as metrical distance [45] . Recall that the reason for the name optical metric is that the light trajectories are now geodesics.

Since the propagation time does not depend upon the independent variable r,

(81)

where the prime denotes differentiation with respect to r, the Euler-Lagrange equation reduces to the first integral, which is

Multiplying numerator and denominator by, we see that we have a right triangle with sides and so that the first integral may be written as:

which is none other than Snell’s law, , when we identify the index of refraction as (66). The angle is the angle that the radius vector makes with the ray.

Since is a cyclic coordinate, Fermat’s principle (81) also gives:

(82)

where instead of its square, (66), as in the first expression in (12). Equation (82) is analogous to, but not the same as, the conservation of angular momentum (78). It remains constant along one and the same ray where is the length of the perpendicular from the origin of the radius vector to the tangent of the ray at the point where the radius vector intersects it [21] .

Solving (82) for the inverse of, we obtain the equation of the ray trajectory as:

(83)

which is analogous to―but not the same as―the equation of the ray trajectory, (79).

Whereas (66) is a bona fide index of refraction, obeying, (77), would seem to imply that, which would be the analogous expression for the frequency shift. However, (77) claims that is a constant because the coordinate time t is a cyclic coordinate in the Lagrangian (75) implying a static process. And because, it would imply, and we would not be able to treat inhomogenous systems like those in a gravitational potential. The same is also true of the Schwarzschild metric.

The space dependency of will cause the wavelength to vary, but it will not cause variations in the frequency in (78). If the wavelength varies and the speed of light is different than c, the frequency remains constant. Consequently, the term in (79) can in no uncertain circumstances be identified as an index of refraction, and the first integral of the motion (77) has no other meaning than to say that proper and coordinate time are related up to a linear transformation.

This proves conclusively that there are no gravitational frequency shifts in the absence of radiation. Said differently, clocks do not slow down in a static gravitational field, because some form of energy transfer would be necessary to allow the frequency to increase when the body is approaching, or decrease when it is receding. In other words, the change in the frequency in the Doppler effect is sustained by the inertial motion of the body.

Whereas the time component of the full metric, (68), would evoke an analogy with a second-order Doppler shift [8] , the optical metric, , in

gives Snell’s law. The relevance of Snell’s law, as opposed to Doppler’s law, rests on the fact that the Schwarzschild metric is static, possessing a pair of Killing vectors. Moreover, is the most natural definition of distance of a light ray. And since light paths coincide with geodesics, Fermat geometry is sufficient to describe the bending of light rays [22] . Time and frequency do not enter such a description so Einstein confused Doppler with Snell; the gravitational potential creates another medium with index of refraction (66).

6. No Aberration No Radiation

In the presence of radiation things are different since the mass in would depend explicitly on time. The time rate of change of the mass would yield monopole radiation. Would it exist, it would follow from the frequency shift.

To predict the frequency shift, we need to determine the ratio of two time intervals: the time interval between two successive wave-crests at the emitter, and those at the receiver. Since we have no way of determining the successive light pulses emitted by the source, we assume that they are given by the same (constant) frequency as that found in the lab, , so the frequency shift would be given by:

(84)

When the source is infinitely far away, , (84) reduces to

(85)

Taking the time derivative of (85) we get:

(86)

Expression (86) says that the rate at which the frequency increases is directly proportional to that at which the mass increases, rather than the expected result that it should be the rate of decrease of frequency.

Consider a charged particle which travels a distance. During which time it emits radiation in all directions which encloses a shell of radius. The angle between the radiation in a given direction and the direction of the particle’s motion is. The angle between where the particle began and its present position, measured from the point on the shell where the radiation first intersects it, is. By the law of sines we have:

Remarkably, this, again, is Snell’s law. For a small angle, , and, we get the angle of aberration as:

(87)

If the charge accelerates for a small time, the enhancement factor is the ratio of the radiative transverse field, , to that of the static field,. The same should hold true for an accelerating mass. Consequently, when the enhancement factor,

is multiplied by the otherwise static gravitational field, , we get the transverse field in the shell,

(88)

where is the component of the mass’s acceleration perpendicular to the radial line. The acceleration in (88) is known as the Euler acceleration, and is responsible for the radiation field falling off as instead of the usual inverse square of the distance,. We thus conclude that without aberration, (87), there can be no radiation, (88).

Over a century ago, Barnett [46] observed that when an initially unmagnetized body is set in rotation a magnetic field is generated parallel to the axis of rotation, which is proportional to the angular velocity,. The gravitomagnetic counterpart, , can be considered as Larmor’s theorem applied to non-Newtonian gravity [12] .

Larmor’s theorem in electrodynamics is expressed in terms of mechanical quantities: the radiation field corresponds to acceleration, and the magnetic field to the velocity of the moving charge. In the gravitational analogue, the magnetic field would correspond to the angular velocity of the rotation of the mass.

This is certainly non-Newtonian on two counts: In Newtonian gravitation the force acts only in a radial direction, and the speed of propagation of the force is infinite so that there can be no aberration, (87). Consequently, there is no analogue of a magnetic field, and no propagation of gravitational waves at the speed of light.

This was appreciated long ago by Heaviside [47] in his construction of a Maxwellian theory of vector gravity, in which he wrote the mass’ acceleration as

in analogy with the Lorentz force, where is a new vector field that is proportional to the angular velocity. Without, there would be no “circuit” equations, and no wave propagation. Heaviside, though, did not place any special importance on the speed of propagation of the gravitational waves, and did not insist that their speed should coincide with the speed of light. For if they did propagate at the speed of light they should travel along null geodesics, and not by the full metric.

Thus, the gravitational Poynting’s vector is:

(89)

where is a unit vector pointed in the radial direction, and. The frequency varies as a function of time due to radiation. Although this is the same result found in ref. [48] , it does not explain why there is a loss of energy because the central mass M is constant, in contrast to (86). If the mass is constant, where is the energy coming from to give a non-vanishing value of Poynting’s vector?

According to some authors [49] , Einstein’s equivalence principle “forbids the very existence of a ‘gravitational Poynting vector’”. However, without a flux term it is impossible to formulate an energy balance equation. In fact, the original presentation of gravitational waves defines a Poynting vector in terms of the components of Einstein’s pseudo-tensor [4] .

Repeating Einstein’s calculation of the energy loss through gravitational radiation, Eddington [4] , begins with Weyl’s [3] classification of gravitational plane waves into three categories: LL (longitudinal-longitudinal), LT (longitu- dinal-transverse), and TT (transverse-transverse). Each class satisfies Einstein’s condition of emptiness:

(90)

for the linearized metric representing small deviations when a gravitational wave passes.

For LL and LT plane waves, the Riemann tensor, vanishes, and they disappear under an appropriate transformation of coordinates. The fact there is no condition on their speed of propagation led Eddington to picturesquely describe such waves as propagating at “the speed of thought”. This left only TT plane waves as possible candidates for gravitational waves, and the condition (90) fixed their speed at the speed of light.

Although Einstein’s condition of emptiness, (90), has been used to derive gravitational plane waves, the material energy tensor is not zero! For Einstein considers a spinning rod of length 2a, and density per unit length, , and obtains an integrated material energy tensor in the zz direction equal to, which is the negative of the stress in the yy direction, where is the angular speed of the spinning bar.

In this “region”, the Ricci tensor,

(91)

does not vanish. How far from this region do we have to be for (90) to apply? Einstein does not answer the question, but assumes that the metric coefficients are now functions of time only, and only those terms in the second derivatives of with respect to t must be retained [4] . Einstein’s equations are now

(92)

where only the yy and zz stresses act on the rod.

There is nothing abnormal about the stresses, yet, when the energy loss is calculated, the Poynting vector is defined in terms of the pseudo-tensor, correct to second order,

(93)

containing only first, and not second, derivatives of the small, perturbed metric, , whose smallness is rather dubious. Second derivatives are necessary to determine curvature, and to make (93) a bona fide tensor. It is rather odd that although the pseudo-tensor is used to form the Poynting vector, it does not contribute to the right-hand side of Einstein’s field equations, (92), and, by the same token, the matter stresses do not enter into the energy balance equation which the Poynting vector must partake in.

In the case of electromagnetic waves, the right-hand side of Einstein’s equations (cf., (94) below), partake in the derivation of Poynting’s vector, but, gravitation is not included in the matter-energy stresses,. The segregation of the gravitational contribution of the stresses from all other forms of mass-energy stresses, , is tantamount to segregating charge and current from the electromagnetic stresses, , which they create.

The pseudo-tensor (93) makes Poynting’s vector a pseudo-vector. And although the flow of energy is mainly along the axis of rotation of the rod, “eight times more intense than in the direction at right angles to the rod” [4] , it is most surprising to learn that the “results have no physical interpretation”.

As Einstein [10] has pointed out, his calculation “gives the total loss of energy of the material system correctly, but the intermediary steps are merely “ana- lytical”. The loss of energy is not localisable anywhere”. [4] It is therefore sur- prising that so much ado has been given to Eddington’s correction to Einstein’s result, , where I is the moment of inertia of the rod, by a mere factor of 2. It is not the numerical correctness that is involved; rather, it is the logical correctness and soundness of the theory that matters.

Gravitational waves need a medium to propagate in just like sound waves for they are the “ripples of spacetime”. However, whereas sound waves satisfy the wave equation “unconditionally”, gravitational waves also do so, “but not unconditionally”. The condition is that “sources shall be such as can occur in practical problems, but it none the less limits the number of admissible solutions” [4] . Simple spherical gravitational waves do not exist, nor are sound waves polarizable. Longitudinal and transverse waves are incomparable.

In contrast to TT plane gravitational waves, electromagnetic waves have a source term in Einstein’s equations [5] ,

(94)

written in terms of the contracted Riemann tensor (90) instead of the Ricci tensor, (91), where

(95)

is the electromagnetic stress tensor which is a bilinear product of the covariant curl of the covariant vector potential, ,

(96)

For (95) there is true Poynting vector, and energy flow is deducible together with the localization of energy. The waves carry away energy―“not a small quantity of second-order but a quantity of first-order” [4] .

Yet, whereas plane gravitational waves need a medium to propagate in, electromagnetic waves can propagate in vacuum. However, all that was done was to add an energy-stress tensor to the right-hand side of (90). The fact that the linearized Einstein equations are used would mean that they are formerly equivalent to Maxwell’s equations so there would be an equivalent to a magnetic field. The effect of the tensorial character of the pseudo tensor is felt beginning at second-order in the perturbed metric. Hence, the covariant character of linearized equations is with respect to Lorentz transformations only [50] , and are inertial. It also clashes with Weyl’s [3] finding that in the linearized version of Einstein’s field equations there is no force acting on matter; in his own words, the gravitational field is a “powerless shadow”.

According to Weyl [3] , “every change in the distribution of matter produces a gravitational effect which is propagated in space with the speed of light”. It appears, in general relativity, that the reciprocal is not true: there can be a gravitational field without matter―even in the absence of distant matter. Einstein’s law of gravitation for “empty” space, the vanishing of the contracted Riemann tensor (90) [51] ,

means that there is no matter present and no physical fields except the gravitational field.The gravitational field does not disturb the emptyness [sic]. Other fields do.

Undoubtedly what Dirac is referring to is what is “left-over” of the Riemann tensor when its trace vanishes on account of Einstein’s condition of emptiness, (90). The remainder, or trace-free part of the Riemann tensor, known as the Weyl tensor, can even be non-zero in the case of no sources. Such would be the case for gravitational waves propagating in spacetime.

The proponents of such an interpretation draw the analogy with electrody- namics waves propagating in a vacuum. However, we know what produced such waves―charges and currents―even if they are not physically present. Yet, we do not know what caused the non-vanishing of the Weyl tensor so the analogy is less than perfect, to say the least. If gravitational waves are the “ripples of spacetime”, or small variations in the curvature of spacetime, what created them? The role of matter can’t be subtracted that easily. And, as we have seen, it isn’t: the TT gravitational waves are derived from Einstein’s condition of emptiness, (90), yet when it comes to calculating energy losses, energy and matter suddenly appear!

It is obvious that if the metric coefficients, , both the Riemann tensor and its contraction³ will vanish. What causes the time-independent coefficients to vary is precisely mass, or mass density, which defines the non- Euclidean disc. In that case both Ricci and Riemann will not vanish.

Loinger [52] quotes Serini’s 1918 theorem to the effect that a zero time- independent contracted Riemann tensor implies a zero Riemann tensor if and only if there are no singularities. But, this defeats the purpose because mass will always create singularities. In that case we do not expect Einstein’s condition of emptiness, (90), to hold either. If the were time-dependent, Serini’s theorem would not be applicable even in the singularity free case. But, this would hardly apply to gravity, and the phenomena related to gravity.

Without an analogue of a magnetic field in the linearized equations there is no energy flow, and, consequently, no gravitational waves. Moreover, the aberration of gravitational waves should be easier to observe than the gravitational waves themselves, since it is basically an optical property due to their finite speed of propagation. There is no need to fabricate an explanation of why the Riemann curvature tensor should be different from zero when its comtraction vanishes [52] , because a double standard has been used to derive gravitational waves: they are the solutions to Einstein’s equations in vacuo, (90), yet in certain regions they possess stresses, (92).

Their distinction with electromagnetic waves are non-existent since the linearized Einstein equations are equivalent to Maxwell’s vector theory of elec- tromagnetic propagation except for the additional condition that Einstein’s equations be satisfied. The existence, or lack thereof, of a medium in which to propagate is not dealt with, and the conclusion that “light cannot be propagated without a change in wave-form” [5] has turned out to be completely unsub- stantiated.

7. Conclusions

The metrics of general relativity are non-Euclidean and hence incompatible with the classical laws of physics which live on Euclidean spaces. Newton’s law is universal whereas those derived from non-Euclidean metrics are limited in space because of the finiteness of the hyperbolic disc. Never has so much effort been consumed in trying to make them compatible, at least in limited domains. However, in no domain can gravity become repulsive nor centrifugal forces be attractive.

The metric itself is incompatible with the nature of the phenomenon being described. The two-time metric should be interpreted as a two-space metric, and phenomena arising from a static gravitational potential should be analyzed as optical phenomena in the realm of geometrical optics. It has been shown that this is the only consistent interpretation in which light rays pass through an inhomogeneous medium created by a static gravitational potential. Time does not enter into the considerations, but the in homogenity of the medium does.

In particular, we have shown that what has been interpreted as a gravita- tional frequency shift, at a constant speed of light, is a shift in the wavelength of light, at a variable speed of light. A discontinuity in the wave surfaces is due to a change in the index of refraction: it is Snell’s―and not Doppler’s―law that applies.

As Weyl [3] and von Laue [53] have remarked, and more recently Loinger [54] , the light emitted by celestial bodies that we observe is transmitted without any change in its frequency. The transmission is accounted for in the realm of geometrical optics. There is no distinction, at least to first-order in the pertur- bation of the metric tensor, between electromagnetic and gravitational wave propagation. Wave propagation is entirely an optical phenomenon. The effect of the static gravitational potential is to cause a discontinuity in the surface through which the wavefronts pass thereby giving rise to refraction.

The geodesic motion that determines the law of wavefronts concerns light rays and not test particles. Test particles are not sources of the gravitational field, and do not exist because no matter how small they would be they would have an associated gravitational field. The assumed smallness is not an argument to exclude such a field. It is inconceivable that such small effects, like the deflection of light by a massive body is being sought, and, yet, the gravitational field of a test particle is completely neglected.

The fact that Einstein’s equations are amenable to a geometric optics inter- pretation does not allow one to replace the propagation of an electromagnetic disturbance by a putative gravitational one. There is a well-defined energy tensor of the electromagnetic field whereas there is no corresponding tensor of the gravitational field, as Einstein realized and Levi-Civita [23] emphasized.

To first-order, there is no distinction between plane gravitational waves and electromagnetic waves. In general relativity there is no distinction between the presence or absence of a propagating medium. The distinction between the vanishing of the full Riemann tensor and its contraction is a red-herring since the material stresses for gravitational waves exist, and, consequently, both are non-zero, even though gravitational waves have been derived under condition (90). A finite propagation speed and a finite flow of energy of gravitational waves means that they behave exactly like electromagnetic waves.

Only geodesic motion has been discussed for which no radiation can occur. The finite propagation of gravitational waves would mean that they manifest the same optical phenomena as electromagnetic waves, which they don’t. Numerical relativity does not reflect the limitations imposed by general relativity nor is it correct in its prediction of gravitational waves. The interference fringes that LIGO [11] observed due to motion of the suspended mirrors cannot be isolated from the interaction with the light beam in the interferometer nor the devices that register them.

NOTES

¹Abramowicz [34] even claims that the correct definition of gravitational acceleration is , just like he would claim that the correct definition of angular momentum would be, according to (43).

²The metric for the inner Schwarzschild solution has constant negative curvature, whose optical metric is the Beltrami metric.

³It is rather fortunate that there is only one meaningful contraction of the Riemann tensor [43] .

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Whittaker, E.T. (1928) Mathematical Proceedings of the Cambridge Philosophical Society, 24, 32-34.
[2]	Levi-Civita, T. (1936) Il Nuovo Cimento, 13, 45-65. https://doi.org/10.1007/BF02961177
[3]	Weyl, H. (1988) Raum⋅Zeit⋅Materie. Springer-Verlag, Berlin. https://doi.org/10.1007/978-3-642-97861-6
[4]	Eddington, A.S. (1922) Proceedings of the Royal Society A, 102, 268-282. https://doi.org/10.1098/rspa.1922.0085
[5]	Baldwin, G.R. and Jeffery, G.B. (1926) Proceedings of the Royal Society A, A111, 95-104. https://doi.org/10.1098/rspa.1926.0051
[6]	Bonner, W.B. (1969) Communications in Mathematical Physics, 13, 163-174.
[7]	Weyl, H. (1944) American Journal of Mathematics, 66, 591-604. https://doi.org/10.2307/2371768
[8]	MØller, C. (1952) The Theory of Relativity. Clarendon Press, Oxford.
[9]	Unruh, W.G. (1976) Physical Review D, 14, 870-892. https://doi.org/10.1103/PhysRevD.14.870
[10]	Einstein, A. (1918) Berlin Sitzungsberichte. 154.
[11]	Abbott, B.P., et al. (2016) Physical Review Letters, 116, Article ID: 061102. https://doi.org/10.1103/PhysRevLett.116.061102
[12]	Mashhoon, B. (2000) Gravitoelectromagnetism. tech. rep. arXiv:grqc/0011014v1
[13]	Einstein, A. (1911) Annalen der Physik, 35, 898-908.
[14]	Alsing, P.M. and Milonni, P.W. (2004) American Journal of Physics, 72, 1524-1529. https://doi.org/10.1119/1.1761064
[15]	Hilbert, D. (1924) Mathematische Annalen, 92, 1-32. https://doi.org/10.1007/BF01448427
[16]	Einstein, A. (1939) Annals of Mathematics, 40, 922-936. https://doi.org/10.2307/1968902
[17]	Eddington, A.S. (1930) The Mathematical Theory of Relativity. 2nd Edition, Cambridge University Press, Cambridge.
[18]	Essen, L. (1971) The Special Relativity: A Critical Analysis. Clarendon Press, Oxford.
[19]	Lavenda, B. (2015) Where Physics Went Wrong. World Scientific, Singapore.
[20]	Ives, H.E. (1939) Journal of the Optical Society of America, 29, 183-187. https://doi.org/10.1364/JOSA.29.000183
[21]	Luneburg, R.K. (1966) Mathematical Theory of Optics. University of California Press, Oakland.
[22]	Lavenda, B.H. (2011) A New Perspective on Relativity: An Odyssey in Non-Eucli-dean Geometries. World Scientific, Singapore.
[23]	Levi-Civita, T. (1917) Ren. Acc. d. Lincei, 26, 381.
[24]	Moore, T.A. (2013) A General Relativity Workbook. University Science Books, Mill Valley, CA.
[25]	Droste, J. (1916) Kon. Ak. Wetensch. Amsterdam, 25, 163. English Translation (1917) Proc. Acad. Sci. Amsterdam, 19, 197.
[26]	Drumaux, P. (1936) Ann. Soc. Sci. Bruxelles, 56, 5.
[27]	Birkhoff, G.D. (1927) Relativity and Modern Physics. Harvard University Press, Cambridge, MA.
[28]	Loinger, A. and Marsico, T. (2009) On Hilbert’s Gravitational Repulsion (a Historical Note). tech. rep. arXiv:0904.1578
[29]	Wu, X. and Xu, C. (1988) Communications in Theoretical Physics (Beijing), 9, 119-125.
[30]	Ohanian, H. (2011) Reversed Gravitational Acceleration at High Speeds.
[31]	Loinger, A. and Marsico, T. (2010) Gravitational Collapses and Hilbertian Repulsion. tech. rep. arXiv:1008.3979
[32]	McGruber III, C. (1982) Physical Review D, 25, 3191-3194. https://doi.org/10.1103/PhysRevD.25.3191
[33]	Chazy, J. (1930) La Théorie de la Relativité et la Mécanique Céleste. Gauthier-Villars, Paris.
[34]	Abramowicz, M.A. (2016) Velocity, Acceleration and Gravity in Einstein’s Relativity. tech. rep. arXiv:1608.07136v1
[35]	Abramowicz, M.A. (1990) Monthly Notices of the Royal Astronomical Society, 245, 733-746.
[36]	McVittie, G.C. (1972) The Observatory, 98, 92.
[37]	Alcubierre, M. and Bruegmann, B. (2001) Physical Review D, 63, Article ID: 104006. https://doi.org/10.1103/PhysRevD.63.104006
[38]	Brandt, S. and Brügmann, B. (1997) Physical Review Letters, 78, 3606-3609. https://doi.org/10.1103/PhysRevLett.78.3606
[39]	Evans, J. and Rosenquist, M. (1986) American Journal of Physics, 54, 876-883. https://doi.org/10.1119/1.14861
[40]	Landau, L.D. and Lifshitz, E.M. (1987) Classical Theory of Fields. 4th Edition, Pergamon Press, Oxford.
[41]	Levi-Civita, T. (1930) Rend. Acc. dei Lincei, 11, 3, 113.
[42]	Leonhardt, U. and Philbin, T. (2010) Geometry and Light. Dover Publications, Mineola.
[43]	Alder, R., Bazin, M. and Schiffer, M. (1975) Introduction to General Relativity. McGraw-Hill, New York.
[44]	Schrödinger, E. (1956) Expanding Universes. Cambridge University Press, London.
[45]	Brown, K. (2015) Reflections on Relativity. lulu.com.
[46]	Barnett, S.J. (1915) Physical Review, 4,239. https://doi.org/10.1103/physrev.6.239
[47]	Heaviside, O. (1894) Electromagnetic Theory, Vol. I. The Electrician, London.
[48]	de Matos, C.J. and Tajmar, M. (2000) Gravitational Poynting Vector and Gravitational Larmor’s Theorem in Rotating Bodies with Angular Acceleration. tech. rep., arXiv:gr-qc/0107014
[49]	Poisson, E. and Will, C.M. (2014) Gravity: Newtonian, Post-Newtonian, Relativistic. Cambridge University Press, Cambridge. https://doi.org/10.1017/CBO9781139507486
[50]	Loinger, A. (2007) Einstein Manifolds and Gravitational Waves. tech. rep. arXiv:0711.3835.
[51]	Dirac, P.A.M. (1975) General Theory of Relativity. Wiley-Interscience, New York.
[52]	Loinger, A. (2005) On Serini’s Relativistic Theorem. tech. rep. arXiv:physics/050417
[53]	von Laue, M. (1956) Die Relativitätstheorie, Vol. II. Friedr. Viewed & Sohn, Braunschweig.
[54]	Loinger, A. and Marsico, T. (2011) On Gravitational Redshift of Spectral Lines. tech. rep. arXiv:1102.5394