

1. Introduction
Consider the following lattice differential equation
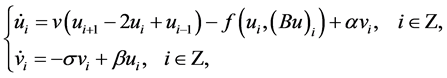 (1.1)
(1.1)
where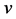 ,
, 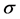 are positive constants,
are positive constants, 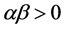 ,
, 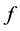 is a
is a 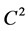 -function, and
-function, and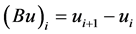 .
.
Lattice dynamical systems occur in a wide variety of applications, and a lot of studies have been done, e.g., see [1]-[4]. A pair of solutions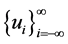 ,
, 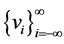 of (1.1) is called a traveling wave solution with wave
of (1.1) is called a traveling wave solution with wave
speed 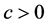 if there exist functions
if there exist functions 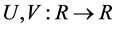 such that
such that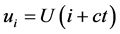 ,
, 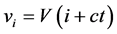 with
with 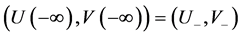 and
and![]() . Let
. Let![]() , note that (1.1) has a pair of traveling wave solutions if and only if
, note that (1.1) has a pair of traveling wave solutions if and only if![]() ,
, ![]() satisfy the functional differential equation
satisfy the functional differential equation
![]() (1.2)
(1.2)
Without loss of generality, we can impose (1.1) with asymptotic boundary conditions
![]() ,
, ![]() ,
, ![]() ,
,![]() . (1.3)
. (1.3)
By the property of equation, we can assume that![]() . In the following, we give some assumptions on nonlinear function
. In the following, we give some assumptions on nonlinear function![]() :
:
![]()
![]() ,
, ![]() ,
,![]() .
.
![]() There exists a positive-value continuous function
There exists a positive-value continuous function ![]() such that
such that
![]() ,
,![]() .
.
![]()
![]() ,
, ![]() ,
, ![]()
![]()
![]() for any
for any![]() ,
, ![]() ,
,
where![]() ,
, ![]() is given in Lemma 2.1.
is given in Lemma 2.1.
![]()
![]() for any
for any![]() .
.
Select positive constants ![]() such that
such that![]() ,
, ![]() , and define operators
, and define operators ![]() by
by
![]()
![]() . (1.4)
. (1.4)
Then, (1.2) can be rewritten as
![]() ,
,![]() . (1.5)
. (1.5)
Define the operators ![]() by
by
![]() .
.
Note that ![]() satisfy
satisfy ![]() and a fixed point of
and a fixed point of ![]() is a solution of (1.2). Denote
is a solution of (1.2). Denote ![]() the Euclidean norm in
the Euclidean norm in![]() . Define
. Define
![]() ,
,
where![]() . Note that
. Note that ![]() is a Banach space.
is a Banach space.
Definition 1.1. If the continuous functions ![]() are differentiable almost everywhere and satisfy
are differentiable almost everywhere and satisfy
![]() (1.6)
(1.6)
Then, ![]() is called an upper solution of (1.2).
is called an upper solution of (1.2).
Similarity, we can define a lower solution of (1.2). The main result of this paper is
Theorem 1.1. Assume that ![]() hold. Then there exists
hold. Then there exists ![]() such that for every
such that for every![]() , (1.2) admits a traveling wave solution
, (1.2) admits a traveling wave solution ![]() connecting
connecting ![]() and
and![]() . Moreover, each component of traveling wave solution is monotonically nondecreasing in
. Moreover, each component of traveling wave solution is monotonically nondecreasing in![]() , and for each
, and for each![]() ,
, ![]() ,
, ![]() also
also
satisfy![]() ,
, ![]() , where
, where ![]() is the smallest solution of the eq-
is the smallest solution of the eq-
uation
![]() .
.
2. Upper-Lower Solutions of (1.2)
Set![]() .
.
Lemma 2.1. Assume that ![]() holds. Then there exists a unique
holds. Then there exists a unique ![]() such that
such that ![]() if
if![]() , then there exist two positive numbers
, then there exist two positive numbers ![]() and
and ![]() with
with ![]() such that
such that ![]() ,
, ![]() in
in![]() , and
, and ![]() in
in![]() ;
; ![]() if
if![]() , then
, then ![]() for all
for all![]() ;
; ![]() if
if![]() , then
, then![]() , and
, and![]() .
.
Proof. Using assumption![]() , we can get the result directly.
, we can get the result directly. ![]()
Lemma 2.2. Assume that![]() ,
, ![]() and
and ![]() hold. Let
hold. Let![]() ,
, ![]() , and
, and ![]() be defined as in
be defined as in
Lemma 2.1, and ![]() be any number. Then for every
be any number. Then for every ![]() and
and![]() , there exists
, there exists
![]() such that for any
such that for any![]() ,
,
![]() ,
,
and
![]()
are a pair of upper solutions and a pair of lower solutions of (1.2), respectively.
Proof. Let
![]() (2.1)
(2.1)
![]() . (2.2)
. (2.2)
Since![]() , there exists
, there exists ![]() such that
such that![]() ,
,![]() . If
. If![]() , then
, then![]() ,
,
![]() . By
. By![]() , we get that
, we get that
![]() ,
,![]() .
.
If![]() , then
, then![]() . By
. By![]() ,
, ![]() , and using
, and using
Lemma 2.1, we get that
![]()
![]() (2.3)
(2.3)
Lemma 2.1 and ![]() yields
yields
![]() . (2.4)
. (2.4)
Thus,
![]()
Therefore, ![]() is an upper solution of (1.2). Similarly, we can prove that
is an upper solution of (1.2). Similarly, we can prove that ![]() is a lower solution.
is a lower solution. ![]()
3. Existence of Traveling Wave
Let![]() ,
,![]() . We have the fol-
. We have the fol-
lowing result.
Lemma 3.1 Assume that ![]() and
and ![]() hold. Then
hold. Then
![]()
![]() and
and ![]() for
for ![]() if
if
![]() satisfy
satisfy![]() ,
, ![]() for
for![]() ;
;
![]()
![]() are nondecreasing in
are nondecreasing in ![]() if
if ![]() is nondecreasing in
is nondecreasing in![]() .
.
Proof. If ![]() such that
such that ![]() and
and ![]() for
for ![]() , then by
, then by ![]() we have
we have
![]() (3.1)
(3.1)
where![]() . Note that
. Note that
![]() (3.2)
(3.2)
Thus, from (3.1)-(3.2), we have
![]()
which implies that![]() . A similar argument can be done for
. A similar argument can be done for![]() . Thus, we can get the desired results.
. Thus, we can get the desired results. ![]()
Lemma 3.2. Assume that ![]() and
and ![]() hold. Then
hold. Then ![]() is continuous
is continuous
with respect to the norm ![]() with
with![]() .
.
Proof. We first prove that ![]() are continuous. Denote
are continuous. Denote ![]()
![]() . For any
. For any![]() , choose
, choose![]() , where
, where
![]() . If
. If ![]() and
and ![]() satisfy
satisfy
![]() , then by (3.1),
, then by (3.1),
![]() (3.3)
(3.3)
Similarly, ![]() is continuous.
is continuous.
By definition of![]() , we have
, we have
![]() (3.4)
(3.4)
If![]() , it follows that
, it follows that
![]() . (3.5)
. (3.5)
If![]() , it follows that
, it follows that
![]() (3.6)
(3.6)
Combining (3.5) and (3.6), we get that ![]() is continuous with respect to the norm
is continuous with respect to the norm![]() . A Similar argument can be done for
. A Similar argument can be done for![]() .
. ![]()
Define
![]()
It is easy to verify that ![]() is nonempty, convex and compact in
is nonempty, convex and compact in![]() . As the proof of Claim 2 in the proof of Theorem A in [5], we have
. As the proof of Claim 2 in the proof of Theorem A in [5], we have
Lemma 3.3. Assume that ![]() hold. Then
hold. Then![]() .
.
Proof of Theorem 1.1. By the definition of![]() , Lemma 3.2-3.3 and Schauder’s fixed point theorem, we get that there exists a fixed point
, Lemma 3.2-3.3 and Schauder’s fixed point theorem, we get that there exists a fixed point![]() . Note that
. Note that ![]() is nondecreasing in
is nondecreasing in![]() , as-
, as-
sumption ![]() and Lemma 2.2 imply that
and Lemma 2.2 imply that ![]()
![]() . There-
. There-
fore, ![]() is a traveling wave solution of (1.1).
is a traveling wave solution of (1.1). ![]()
Acknowledgements
This work was supported by the NNSF of China Grant 11571092.